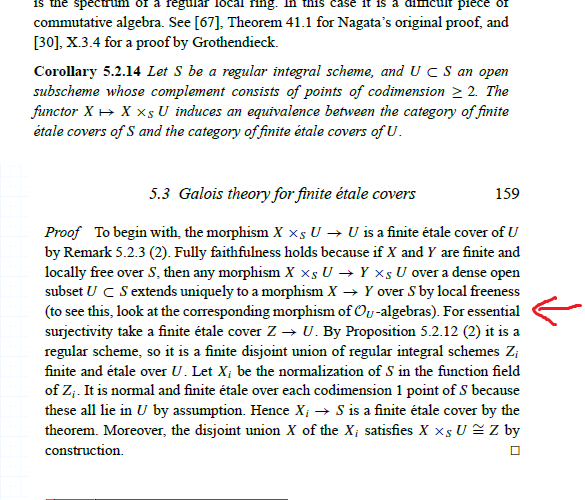
I have a question about following argument used in Szamuely's "Galois Groups and Fundamental Groups" in the excerpt below (or look up at page 159):
Let $X,Y$ schemes which are finite and locally free over base scheme $S$. Let $U \subset S$ be dense in $S$.
If we have a morphism $X \times_S U \to Y \times_S U$ over $U$ why does it extend to a morphism $X \to Y$ over $S$?
My considerations: The problem is local so wlog $S= Spec(R), X= Spec(R^n), Y= Spec(R^m)$(by locally freeness) and $U= D(f)$ for a $f \in R$.
Therefore the problem is reduced to the following affine case:
If $R^m \otimes_R R_f = R_f^m \to R_f^n$ a ring morphism why does it uniquely induce a ring morphism $R^m \to R^n$ uniquely?
Remark: Denote by $R_f$ the localization of $R$ at $f$.
Sure I can compose it with $R^m \to R^m_f$ but does the resulting map $R^m \to R_f^n$ factorize (uniquely) thought $R^n$?
Or did the author mean another approach?