I'm writing a paper and, at a certain point, I need the following, rather elementary
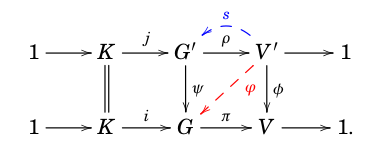
Lemma. Assume that we have a commutative diagram of short exact sequences of groups of the form
Then the liftings $\varphi \colon V' \to G$ of $\phi \colon V' \to V$ are in bijective correspondence to the splittings $s \colon V' \to G'$ of the top sequence.
One direction is clear: if $s \colon V' \to G'$ is a splitting of the top sequence, then $\varphi= \psi \circ s$ is a lifting of $\phi$. Conversely, a tedious but straightforward diagram chasing shows how to construct a splitting starting from a lifting.
Since the paper is quite long, I'm trying to shorten it and, in particular, I would like not to present the standard proof of this result. On the other hand, I do not like those sentences of the form "The straightforward proof of the following easy statement is left to the reader", hence I'm looking for a reference.
I searched some textbooks of Group Theory and Homological Algebra, so far without success. However, my impression is that it should be somewhere, maybe buried in some exercise.
Question. Where is it possible to find a proper reference to the above lemma?