6
$\begingroup$

Consider a unitary modular TQFT, defined by the F and R moves. More specifically, a braided tensor category relevant for anyon models in 2D topologically ordered phases of matter. I am interested in the value of the F-move, $[F^{abc}_1]_{\bar{a}\bar{c}}$, diagrammatically defined by 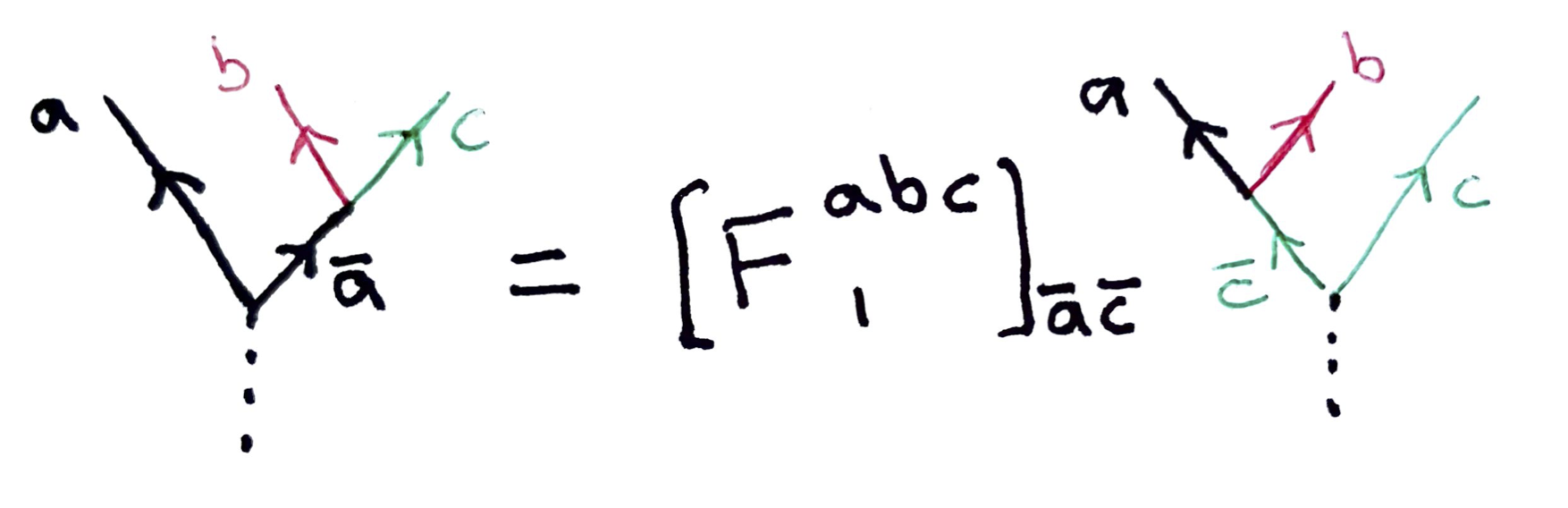enter image description here where $1$ is the vacuum and $\bar{a}$ is the inverse of $a$, i.e. $1 \in \{a\times \bar{a}\}$

I would naively expect this to be the identity. However, in https://thesis.library.caltech.edu/2447/2/thesis.pdf the author states that this need not be the case.

I think this is related to bending, and/or the $\mathbb{Z}_2$, or possibly the $\mathbb{Z}_3$, Frobenius Schur indicator. Any clarification (or references) on the value of this F-move would be much appreciated!

Improve this question
$\endgroup$
3
  • $\begingroup$ Maybe this is clear to experts, but what is the input data for this TQFT? Namely, what dimension is it in, and what structure is used as input to define the TQFT (fusion category? modular tensor category? etc.)? Alternatively, is this from a theory (or class of theories) with a specific name? $\endgroup$
    – Arun Debray
    Commented Apr 6, 2019 at 23:38
  • 1
    $\begingroup$ Sorry, I am far from an expert. I have added some clarification to the question. Is this better? $\endgroup$
    – as2457
    Commented Apr 7, 2019 at 7:48
  • $\begingroup$ yeah, that clears it up for me. Thank you! $\endgroup$
    – Arun Debray
    Commented Apr 7, 2019 at 20:40

1 Answer 1

Reset to default
4
$\begingroup$

Consider the case $a=b=c$. Then the fusion space $V_{aaa}$ affords a representation of $\mathbb Z/3$ via a $2\pi/3$ rotation. The F-move in your question is essentially this $2\pi/3$ rotation, and the rotation need not act trivially (its eigenvalues might be non-trivial 3rd roots of unity).

See for example eqn 470 of https://arxiv.org/pdf/1709.01941.pdf , which cites https://arxiv.org/pdf/0704.0208.pdf .

[edit:]

In response to the questions in the comments:

(1) Yes, this is sometimes called the $\mathbb Z/3$ Frobenius-Schur indicator.

(2) Away from the case $a=b=c$, it does not (strictly speaking) make sense to ask whether the F-moves in question are the identity, because they are linear maps between distinct vector spaces $V_{abc}$, $V_{bca}$ and $V_{cab}$. [Another edit: I'm using here the fact that $V_{1a\overline a}$ and $V_{1\overline cc}$ (from the figure in the question) are 1-dimensional and have canonical generators, so that in this particular case the F-move is equivalent to a map from $V_{abc}$ to $V_{cab}$.] However, if we chose bases of those vector spaces appropriately, we can arrange for the matrix representations of the F-moves to be the "identity" matrix (1s down the diagonal). Note that in general these matrices are only well-defined up to unitary change of bases in the various $V_{xyz}$, so with a different choice of basis we can arrange for the F-matrix in the question to be any unitary matrix that we like.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Is this the only case that this would be different to the identity? $\endgroup$
    – as2457
    Commented Apr 7, 2019 at 7:37
  • $\begingroup$ What you're referring to is sometimes called the $\mathbb{Z}_3$ Frobenius Schur indicator, is this correct? $\endgroup$
    – as2457
    Commented Apr 7, 2019 at 7:53
  • 1
    $\begingroup$ I added some remarks to the answer. $\endgroup$
    – Kevin Walker
    Commented Apr 7, 2019 at 13:31
  • $\begingroup$ Thanks for the clarification. However, I'm not sure about your second point. I don't think the F-moves relate different vector spaces, they relate the different decompositions of the same vector spaces. That is, they define the associativity of the tensor product of vector spaces. Please correct me if I'm wrong. I also agree that there is a gauge freedom (except for certain gauge invariant F-moves). I guess the more precise question is, can we always fix the gauge to make this element equal to 1? $\endgroup$
    – as2457
    Commented Apr 7, 2019 at 14:59
  • $\begingroup$ I'll add some clarification to the answer. I'm using certain canonical elements of the 1-dimensional vector spaces $V_{1a\overline a}$ and $V_{1\overline cc}$. $\endgroup$
    – Kevin Walker
    Commented Apr 7, 2019 at 17:59

You must log in to answer this question.