0
$\begingroup$

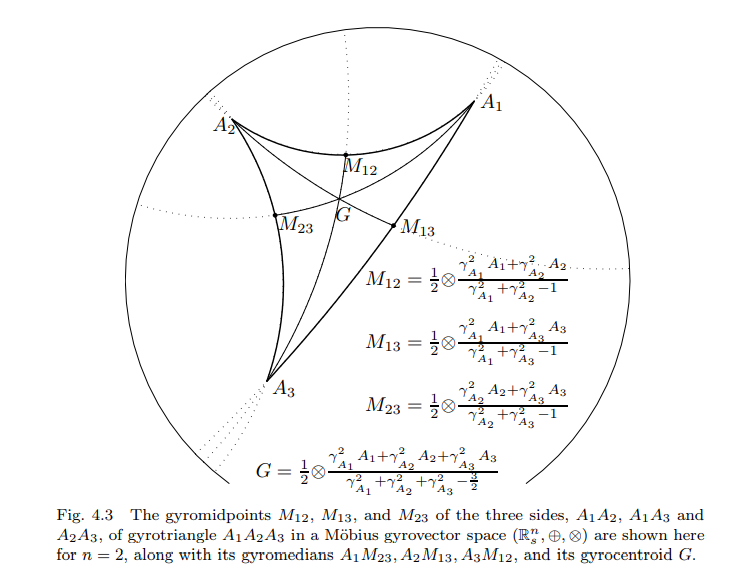
In his book Barycentric calculs in Euclidean and hyperbolic geometry, A. A. Ungar defines the gyromidpoint of a segment in a Möbius gyrovector space. The Poincaré disk model in dimension 2 and the Poincaré ball model in dimension 3 can play the role of the Möbius gyrovector space. That said, the formula for the gyromidpoint does not work when a vertex of the hyperbolic segment is at the boundary of the disk/ball (there are some divisions by zero and some infinite quantities, and I'm not able to see whether there's a limit when a vertex approaches the boundary). Is it possible to define the gyromidpoint in this case and what is its formula?

enter image description here

Improve this question
$\endgroup$
2
  • $\begingroup$ Could you define what this point is supposed to represent using the language of hyperbolic geometry (rather than the one of gyro vector spaces)? Ungar's terminology tends to be nonstandard and I doubt anybody at MO knows the meaning. $\endgroup$
    – Misha
    Commented Apr 10, 2019 at 18:55
  • $\begingroup$ @Misha The midpoint is equidistant from the two points. I finally think it makes no sense if a point is at the boundary, because the distance is infinite. $\endgroup$
    – Stéphane Laurent
    Commented Apr 11, 2019 at 6:06

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .