Symmetries for Julia sets of perturbations of polynomial maps

Question

This is a naive question. Consider the Julia sets of the map $$ z \mapsto z^n + \lambda / z^k $$ with $z,\lambda \in \mathbb{C}$, and the exponents $n,k \in \mathbb{N}$. For example, for $n=k=3$, here are three Julia sets depictions for three different (relatively small) $\lambda$'s:

---

         
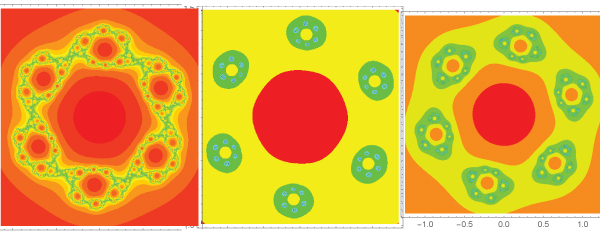
          ^{L-to-R:    $\lambda=0.2-0.1 i$;   $\lambda=2-i$;    $\lambda=0.07-0.5i$.}

---

There is obvious hexagonal symmetry independent of $\lambda$. My question is:

Q. Given $n$ and $k$, are the symmetries of the Julia sets of this map, for relatively small $\lambda$, known? Should they always have $(n+k)$-gon-like symmetries?

I can imagine that many details of these maps are unknown, but perhaps at this high-level viewpoint, the gross structure of the Julia sets is known? So for $n=4$ and $k=5$, should we expect to see nonagonal symmetries?

Answer 1

Yes. Let $\zeta=e^{2\pi i/(k+n)}$. Then $f(\zeta^j z)=\zeta^{nj}f(z)$. Iterating this, the orbit of $\zeta^j z$ agrees with the orbit of $z$ up to multiplying by powers of $\zeta$. In particular, $z$ lies in the Julia set if and only if $\zeta z$ lies in the Julia set.