1
$\begingroup$

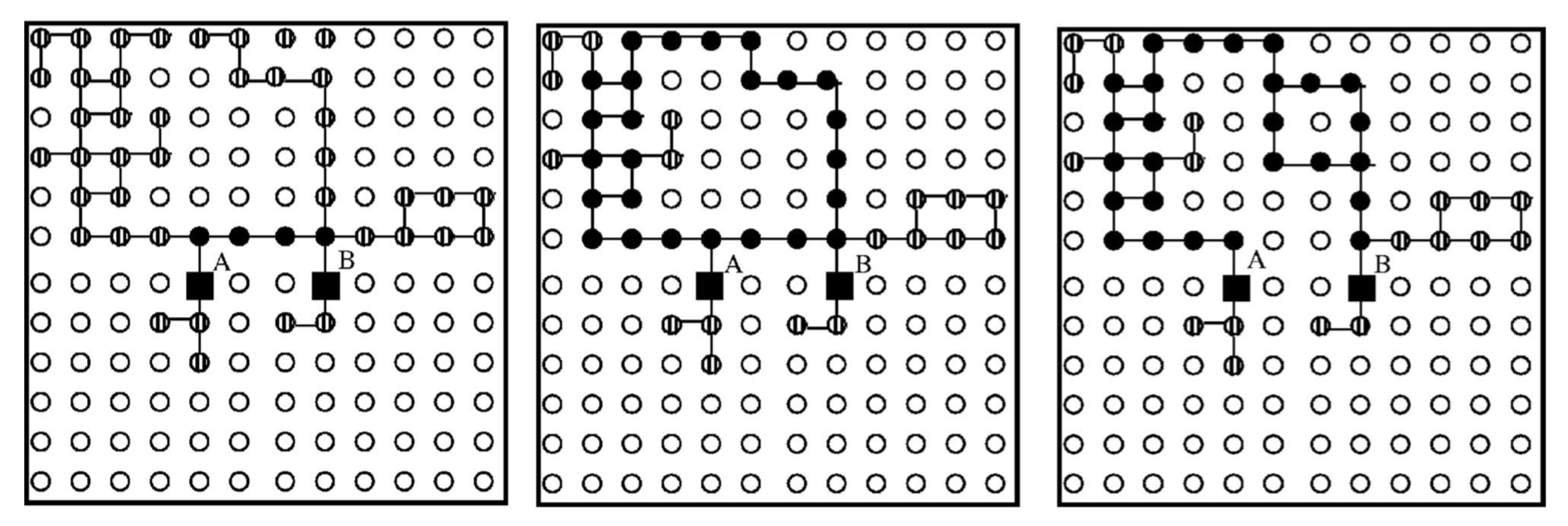
In the context of percolation, e.g., bond/site percolation, random graph connectivity in 2-3 dimensions, etc., once the percolation threshold is reached, that is the system is spanned by an infinite cluster (or in the finite case a cluster connecting two boundaries of the system), the behaviour of backbone of this cluster may be of particular interest. In general, skimming through various related literature one comes across slightly differing definitions for the backbone that tend to coincide. To name a few formulations for the backbone:

a) Suppose we have two vertices $i$ and $j,$ if we apply a potential difference between them, then the set of current carrying bonds (bonds of the percolating cluster having non-zero flow of current) between $i$ and $j$ is called the backbone, and the removed ones are dangling ends. In other words, the backbone is a subset of the percolation cluster formed by removing all dangling ends, branches, etc. that are not necessary for maintaining the connectivity of the structure.

  • The definition in a) given in terms of current flow between two nodes (so defined closely related to flow problems), does not seem to exclude the possibility of having loops/cycles in the backbone? But given Kirchhoff's second law, namely that the sum of all potential differences around a complete loop is zero, such loops are trivially dismissed (?) in the definition of a), or possibly their inclusion would bear no difference on the properties of the backbone of the percolating cluster.

b) However, leaning more on a mathematical definition, the backbone is also sometimes defined as a cluster connecting $i$ and $j$ such that it can be explored by a self-avoiding walk. On the other hand, sometimes it is given a more practical definition related to shortest path between $i$ and $j,$ namely, a backbone can be found by choosing a vertex randomly on the cluster (connecting $i$ and $j$) and retaining only the vertices and bonds that belong to the shortest path between them. Finally, more descriptively, the backbone is also described as the part of the percolating path that is free of cycles and dangling ends.

  • Compared to a), the types of definitions in b) seem to suggest more clearly that loops are not taken as part of the backbone (as e.g. it would undermine the self-avoiding walk). Similarly, when defined in relation to the shortest path, the loops would become irrelevant.

Question:

  • Given the definitions in a) and b), can a consistent definition be drawn for what is meant by the backbone of a percolating cluster? Are the existence of these somewhat differing definitions a case of rigour versus practicality?
  • On the computationally practical side, what would be a reasonable working definition for the backbone?

I admit I may have mis-interpreted the above definitions (e.g. the loop aspect when considering current flow between $i$ and $j$), or the self-avoiding walk description, which to my understanding, would dismiss dangling ends and loops from the backbone.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$


source

Both striped sites and black sites belong to the percolation cluster, but only the black sites are part of the backbone. The backbone can be defined as the set of current carrying paths from A to B, or equivalently as the set of self-avoiding paths from A to B. As you can see, loops are included in the backbone, according to both definitions. The shortest path length must be less than the number of sites on the backbone (the "backbone mass"), but it can be much shorter --- as you see in the central panel.

Note in particular that loops are included also in the second definition, because you can take two self-avoiding walks, one that goes clockwise and one counterclockwise through the loop. Dangling ends are indeed excluded.

There is a different concept known as the "elastic backbone", that is the union of all shortest paths. An efficient computational method to obtain both the backbone and the elastic backbone is presented in Backbone and elastic backbone of percolation clusters obtained by the new method of 'burning' (1984).

Improve this answer
$\endgroup$
3
  • $\begingroup$ Many thanks, this is indeed very clarifying. So essentially, from a computational point of view, once the dangling ends are removed from the percolating cluster then we'll have extracted the backbone? (that is, any non-dangling part qualifies as current carrying?) I got confused about the loops essentially after this paper: iopscience.iop.org/article/10.1088/1751-8113/40/49/… where above the Fig.2 they mention that "The backbone can be identified after all the resistors ... As shown in figure 2, all of the dangling ends, loops and arcs carry no current." $\endgroup$
    – user929304
    Commented Mar 8, 2019 at 10:14
  • $\begingroup$ if you look at figure 2, you see that the yellow parts do carry a current; the caption refers to these as "blobs", but these are what you would call "loops". $\endgroup$
    – Carlo Beenakker
    Commented Mar 8, 2019 at 10:43
  • $\begingroup$ Indeed, this is quite strange in that paper, as they seem to talk about "loops" and "blobs" as though they are different structures. $\endgroup$
    – user929304
    Commented Mar 8, 2019 at 11:23

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .