What are some mathematical sculptures?

Question

Either intentionally or unintentionally. Include location and sculptor, if known.

Answer 1

The Mathematical Research Institute in Oberwolfach have a sculpture on their grounds depicting Boy's surface:

_{(source: mfo.de)}

Answer 2

Helaman Ferguson. My department has one of these in the main office:

_{(source: helasculpt.com)}

Keizo Ushio. He made this during the 2006 ICM in Madrid:
_{(source: memenet.or.jp)}

Answer 3

Robert Longhurst made some surfaces as wood carvings.

Answer 4

The centrepiece of McAllister building, which houses the math department at Penn State, is the Octacube, designed by Adrian Ocneanu.

There's a bit of a description of the mathematics behind the Octacube on the Penn State website, but unfortunately, that's the most material that I can find online. There are all kinds of animations set up to display on a computer terminal in McAllister building, but they don't seem to be available online anymore.

Very briefly, the mathematics of the sculpture is as follows: consider the four-dimensional regular convex polytope whose vertex set is the union of the vertex sets for the four-dimensional cube {(±1,±1,±1,±1)} and the four-dimensional octahedron {(±2,0,0,0), (0,±2,0,0), (0,0,±2,0), (0,0,0,±2)}. Consider the 1-skeleton of this polytope (vertices and edges), and project radially to S³ ⊂ ℝ⁴. Project the resulting "inflated polytope" stereographically to ℝ³, and "fatten" the edges so that a cross-section of an edge is no longer just a point, but a Y-shape (see the corners of the sculpture). What you get is the sculpture shown.

Answer 5

MoMath, in partnership with Make, has a regular feature on DIY math sculptures. I personally like the space filling curve made of steel pipe ells by Chaim Goodman-Strauss and Eugene Sargent.

Answer 6

A model of the Riemann zeta function suggesting the zeroes and pole. One of several models at the University of Regensburg Math Dept.

3-D Permutahedron Sundial by Stefano Buonsignori (16th century) in the Medici collection presented by Museo Galileo. The 3-D permutahedron:

(The combinatorics underlying the geometry of the permutahedra are related to multiplicative inversion and, consequently, to Koszul duality and the formalism of Appell sequences and associated matrices, so I find this particular jewel at the juncture of art, engineering, geometry, combinatorics, and analysis particularly exciting. If only he had done one for the associahedron!)

Calabi-Yau space: the shape of the inner space of the universe. In Yau's hometown in south China.

See 3-D animation in "Where math meets physics" by Brockmeier, also intro in "Hidden dimensions" by Freiberger and the survey "The Calabi-Yau Landscape:from Geometry, to Physics, to Machine-Learning" by Yang-Hui He.

Eversion of a sphere: Models in resin made by Stewart Dickson of clay models made by Bernard Morin (a blind topologist!) of different stages of the eversion of a sphere. The resin models were presented at the Maubeuge symposium "Arts et Mathématiques." (Photo by John Sullivan.)

Excerpt (p. 146) from "Poincare's Prize" by G Szipiro:

(Smale) proved a theorem that showed it is possible to evert the sphere. But the procedure implicit in his theorem was so complicated that nobody could visualize it. Smale, and others who tried, wanted to see with their own eyes how the sphere would evert. For once, the ability to visualize may actually have been a handicap. It was left to the blind mathematician Bernard Morin to devise a procedure to turn a sphere inside out that could actually be implemented (if the membrane is able to pass through itself, that is.)

A sculpture by Gideon Weisz of an approximation of Alexander's horned sphere to five levels.

(Photo from “The Universal Book of Mathematics: From Abracadabra to Zeno's Paradoxes” by David Darling.)

'Tripartite unit' [Unidade Tripartida], Max Bill

This sculpture is topologically equivalent to the connected sum of three projective planes with a point removed, corresponding to the only boundary component of the represented surface.

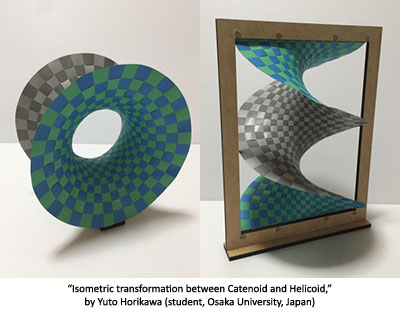
Isometric transformation between a catenoid and helicoid

Presented at the 2019 Mathematical Art Exhibition held at the AMS Joint Mathematical Meetings in Baltimore, MD. (30 x 25 x 25 cm, paper, mdf, steel, magnet, 2018.)

To the left is a paper-crafted catenoid, and to the right, a helicoid. These surfaces can be transformed isometrically into each other, i.e., without any strain, because the first fundamental form of the surfaces are identical.

I made this model by weaving together paper strips whose shapes were obtained by minimizing the strain energy defined on a Riemannian manifold. The optimization problem was solved numerically using NURBS-based isogeometric analysis.

• Yuto Horikawa

Dandelin spheres (description)

Clebsch diagonal surface (description)

The two models above are part of the M. Schelling Collection of the Geometric Models Collection at V. N. Karazin Karkiv National Univ. (See historical notes for the collection and in comments below.)

Fermi surface of copper with permutohedral Brillouin zone

(Cavendish Laboratory Museum)

As Kaganov and Lifshitz put it, the Fermi surface is‘the stage on which the ‘drama of the life of the electron’ is played out. -- Dugdale from Life on the edge: a beginner’s guide to the Fermi surface

See also "The Magic Wand Theorem of A. Eskin and M. Mirzakhani" by Zorich

Gates to the Center for Mathematical Studies at Cambridge

From "What is ... a Skein Module" by Lickorish:

Mathematical Gates. The main entrance to the Centre for Mathematical Sciences in Cambridge is sealed by iron gates. Medallions in these gates show the only two knots with at most eleven crossings, other than the unknot, that have trivial Alexander polynomial. One gate shows the knot studied by S. Kinoshita and H. Terasaka, whilst the other gate shows the knot discovered by J. H. Conway in his classification of eleven-crossing knots. Related as they are by one of Conway’s mutations they cannot be distinguished by any invariant based on skein theory. The medallions were created by John Robinson and donated by Damon de Laszlo and Robert Hefner III.

Dodecahedron at Burning Man

From Satayan L. Devadoss:

UNFOLDING HUMANITY: BURNING MAN (2018)

A two-ton metal, wood, and acrylic interactive sculpture, showcasing unsolved problems of mathematics, came to life in the middle of the Nevada desert. Rising 12 feet tall with an 18-foot wingspan, the unfolding dodecahedron was externally skinned with black panels containing 2240 acrylic windows, illuminated by over 16,000 LEDs which were programmed and driven by 20 controllers. The interior, large enough to hold 15 people, was fully lined with a massive mirror over each of the twelve pentagonal faces.

Our motivating question asks, what could it look like for vibrant and unsolved mathematics to be made embodied and physical, to engage the general public? And so, this sculpture deals with two unsolved questions: the unknown possibility of unfolding any convex polyhedra (motivated by 500-year-old works of Albrecht Dürer) and the shape of our universe (from WMAP cosmic microwave background radiation data and the Poincaré dodecahedral space).

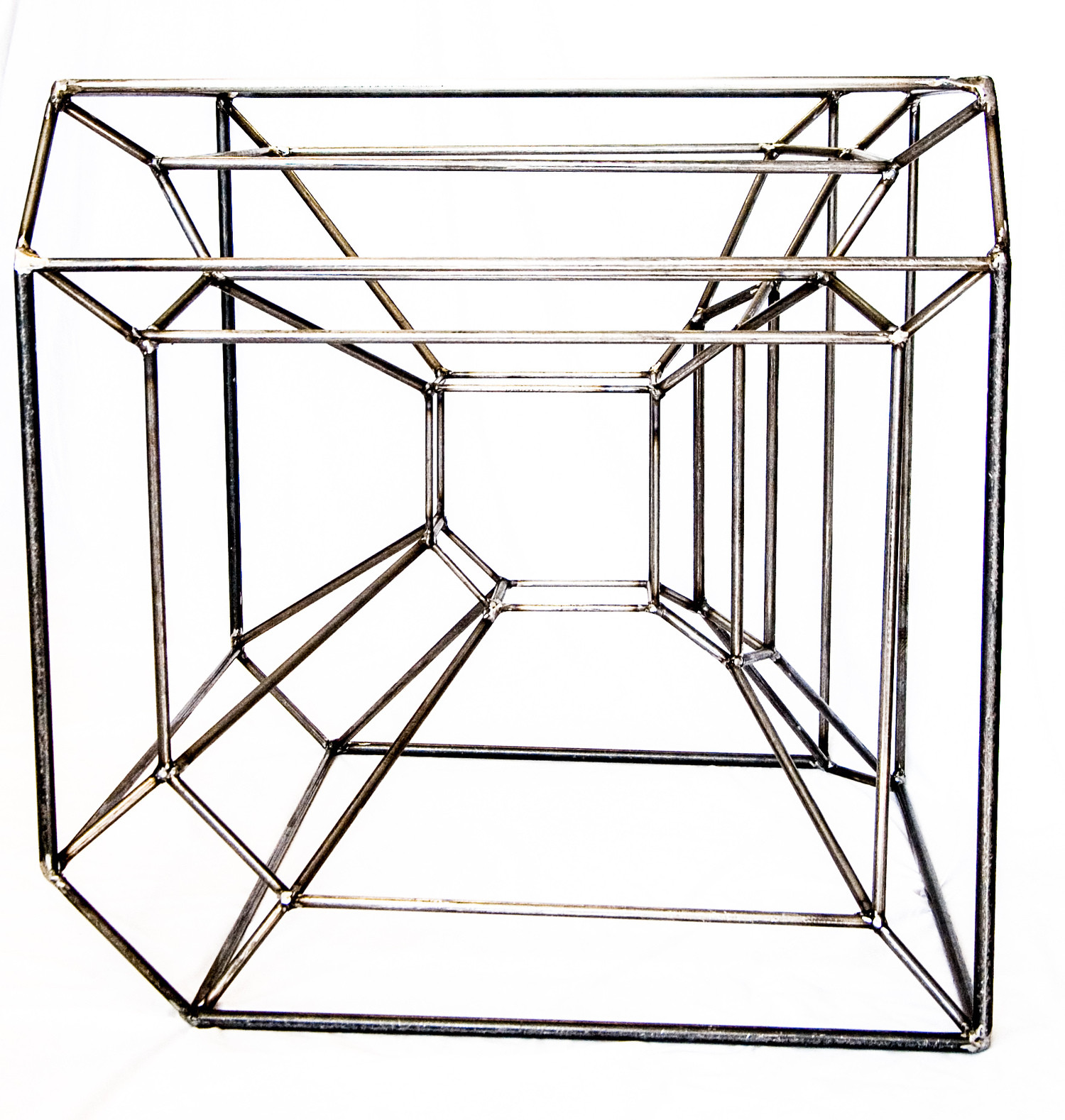
3-D Projection of a 4-D Associahedron

A metal skeletal framework created by Eric Jonash and Sam Kapala via truncations of successively higher dimensional cells of the 4-simplex. See See Figs. 3.14 on pg. 76 and 7.28 on pg. 241 of Discrete and Computational Geometry by Devadoss and O'Rourke. "The vertices of this polytope correspond to all ways of triangulating the regular seven-gon, and the edges connect two such triangulations related by a flip."--Devadoss at link above.

A Seifert Surface Bordered by Borromean Rings

This surface has three edges, each a simple closed loop, which are locked together in an ancient form called the Borromean Rings. Named after its use in an Italian coat of arms, these three rings are locked together inextricably although no two of them are linked. Their Seifert surface twists through the loops smoothly and gracefully, ... . -- Bathsheba Sculpture

See also J. van Wijk's Visualization of Seifert Surfaces.

Answer 7

Adding to the list two of my favorite mathematical sculptors:

George Hart:

http://www.georgehart.com/sculpture/sculpture.html

Bathsheba:

http://www.bathsheba.com/

Finally, there are a lot of nice things at the new Geometry Playground in the Exploratorium, for anyone coming through the SF bay area:

http://www.exploratorium.edu/geometryplayground/

Answer 8

A month ago our team completed, over four days, a very large geometric sculpture out of 20 tons of snow. Eva Hild of Sweden designed it and came over to work with us. The complete story is at

http://stanwagon.com/snow/breck2011/index.html

The videos linked at the top of the page allow you to walk around the work.

Eva Hild, her model, and our completed work. A view at night, showing the effects of the new LED lighting system.

Stan Wagon

Answer 9

at Ohio State ...

The Garden of Constants is a sculptural garden of large numerals that highlight the activities performed in nearby College of Engineering buildings. The installation, by Barbara Grygutis, includes a black walkway featuring 50 individual formulas cast in bronze and embedded in handmade pavers. The Garden of Constants is on the lawn of Dreese Laboratories.

Here we see 6 and 8. The perfect number is golden colored.

Answer 10

Borromean rings on campus of George Washington University in Washington, DC. Unfortunately, I didn't make a note of the name of the artist.

Answer 11

I suspect that the Octacube is also the only mathematical sculpture (possibly the only mathematical topic?) to appear in the journal Playboy (March 2006).

Answer 12

Batsheba Grossman

(displayed this one here on MO before)

Answer 13

Cliff Stoll makes Klein bottles and sells them too. He's made the "worlds biggest klein bottle". You can see that here.

Answer 14

I think tensegrity sculptures are cool. Kenneth Snelson does a lot of these.

_{(source: well.com)}

Answer 15

Alessandro Giorgi has made a bunch of statues concerned with the mathematics of juggling, e.g. 1 and 2.

Answer 16

The sculptures of Morton C Bradley are not so widely known. He was originally an art conservator in Boston, but he also explored geometric shapes and color to create many sculptures. They are "a reflection of his fascination with the science of color, his admiration for traditional patterns, his exploration of mathematical designs." (Quote from web site below.) He never sold a single piece of work. At his death he donated his entire estate to Indiana University. The IU Art Museum is cataloging his work and arranging exhibits. They've created a web site http://www.iub.edu/~iuam/online_modules/bradley/ that discusses and displays some of his work. In comparison with other work already mentioned here, perhaps the most significant thing is his deep exploration of color in his sculptures.

Answer 17

I am by coincidence in Paris at this moment, attending a meeting (the first) of ESMA, the European Society for Math and Art. George Hart is giving a talk in two hours on his sculpture. The website of ESMA is here: http://mathart.eu/ and the program of the meeting here: http://mathart.eu/en/conf10program.html, and a web tour of the associated exhibition is here: http://mathart.eu/ihp10/index.html There was a talk yesterday about Stan Wagon's snow sculptures by John Sullivan, one of the team.

Answer 18

A ruled surface seen at the Peggy Guggenheim collection in Venice (I believe it is by Antoine Pevsner):

Answer 19

Here are some sculptures by Henry Segerman: https://plus.google.com/u/0/photos/102006004474081559466/albums/5946201022131183089

Answer 20

John Robinson

Among several of his sculptures, personally for me the neatest one -

(it's at the Newton Institute)

Answer 21

Stan Wagon (with various collaborators) is known to make snow sculptures that are mathematically pleasant.

Answer 22

I like the 4 dimensional mathematical sculptures by Bathsheba Grossman, such as the 24-cell:

http://www.bathsheba.com/math/24cell/24cell_new_th.jpg

Are the cryptographic sculptures by Jim Sanborn -- Cyrillic Projector, etc. -- close enough to a "mathematical sculpture"?

http://www.ncarts.org/images/afsb_art/workpix/14.jpg

Answer 23

The MSRIs eightfold way

Answer 24

Does the National Aquatics Centre in Beijing count? It illustrates the Weaire-Phelan structure, a recent (and the first) counterexample to Kelvin's conjecture.

Here's one of a large number of nice mathematical sculptures at the Science Museum in London.

This sculpture is made of 30 interlocking pieces and requires many hands to assemble or disassemble.

This sprinkler illustrates a theorem that a 3d solid can be constructed to cast any desired set of silhouettes (also illustrated on the cover of Gödel, Escher, Bach).

The last three were found on the three-dimensional geometry page of this wonderful collection.

Finally, a shameless plug of one of my own questions.

Answer 25

Check these out...they spring into shape by creating parallel folds on an initial paper shape:

http://erikdemaine.org/curved/

Erik Demaine is a mathematician (computational geometer) at MIT.

Answer 26

The Found Math galleries at the MAA website include many mathematical sculptures.

Answer 27

There are a few lying around Fine Hall in Princeton. One I think is "Five Disks: One Empty" by Alexander Calder.

There's also Marc Pelletier's "Polydodecahedron" which was gifted to JH Conway. http://www.princetonoccasion.org/quarkpark/pages_statements/Conway.html

There's also this big Obelisk in the common room (or did it get moved recently? I seem to remember its relocation when they redid the flooring). I had forgotten what it is actually called and what mathematics it is supposed to represent. Someone should edit this to add in the details.

Answer 28

"Tucker's group of genus 2" by Duane Martinez and DeWitt Godfrey

http://www.colgate.edu/news/blog/archives/archivedisplay?nwID=5031

http://commons.wikimedia.org/wiki/File:Tucker%27s_Genus_Two_Group.jpg

Answer 29

The french sculptor Bernar (sic) Venet: see http://images.math.cnrs.fr/Bernar-Venet-de-l-art-et-des.html

Answer 30

The gömböc—as, for example, in this image—is a homogeneous convex solid with one stable, one unstable, and no neutral point of equilibrium on a horizontal plane. Its existence was conjectured by Vladimir Arnol'd in 1995 proved in 2006 by Gábor Domokos and Péter Várkonyi. Its manufacture requires great precision, and true gömböcs are not hand-made by individual artists.