Suppose $c>2$ is a real number, and $x$ solves equation $f(\frac{1}{2}+x,m,2m)=(2m+1)cx$ for some $m>0$ integer number, where $f$ is probability mass function for binomial distribution, with probability $\frac{1}{2}+x$ and population size $2m$.
Simulations show that it must hold: $Pr(X\geq m+1)\leq \frac{1}{2}+\frac{1}{c}$, where $X$ random variable is distributed binomially with probability $\frac{1}{2}+x$ and population size $2m+1$. Hints how to prove the inequality?
Let us prove a result stronger than what was requested. As in the OP, let $X=X_p$ be a random variable (r.v.) distributed binomially with parameters $2m+1$ and $p:=\frac12+x$ (so that $x=p-\frac12$) and let $Y=Y_p$ be a r.v. distributed binomially with parameters $2m$ and $p=\frac12+x$.
Then the condition $f(\frac12+x,m,2m)=(2m+1)cx$ in the OP can be rewritten as \begin{equation*} c=c_p:=\frac{P(Y_p=m)}{(2m+1)x}, \end{equation*} so that $0<x\le\frac12$. The conjecture in the OP can now be rewritten as \begin{equation*} g(p):=P(X_p\ge m+1)\overset{\text{(?)}}\le\frac12+\frac1c=\frac12+\frac{(2m+1)x}{P(Y_p=m)} \end{equation*} for $p\in(\frac12,1]$.
Let us prove the following much stronger result: \begin{equation*} P(X_p\ge m+1)=g(p)\overset{\text{(?)}}\le h(p):=\tfrac12+(2m+1)x\,P(Y_{1/2}=m) \tag{1} \end{equation*} for $p\in[\frac12,1]$.
We have \begin{equation*} g(p)=\sum_{j=m+1}^{2m+1}\binom{2m+1}j p^j q^{2m+1-j}, \end{equation*} where $q:=1-p$, whence \begin{align*} g'(p)&=\sum_{j=m+1}^{2m+1}\frac{(2m+1)!}{j!(2m+1-j)!} jp^{j-1} q^{2m+1-j} \\ &-\sum_{j=m+1}^{2m+1}\frac{(2m+1)!}{j!(2m+1-j)!}(2m+1-j)p^j q^{2m-j} \\ &=(2m+1)\sum_{j=m+1}^{2m+1}\binom{2m}{j-1} p^{j-1} q^{2m+1-j} \\ &-(2m+1)\sum_{j=m+1}^{2m}\binom{2m}j p^j q^{2m-j} \\ &=(2m+1)\binom{2m}m p^m q^m \\ &\le(2m+1)\binom{2m}m (\tfrac12)^{2m} =(2m+1)P(Y_{1/2}=m). \end{align*} So, for $p\in[\frac12,1]$, \begin{equation*} g(p)\le g(\tfrac12)+(2m+1)P(Y_{1/2}=m)(p-\tfrac12)= \tfrac12+(2m+1)P(Y_{1/2}=m)x=h(p), \end{equation*} so that the question mark in (1) can be removed.
Remark. Using the first 5 lines of the above 6-line display, we can also get the following lower bound on $P(X_p\ge m+1)$: \begin{equation*} P(X_p\ge m+1)\ge \tfrac12+(2m+1)x\,P(Y_p=m); \end{equation*} cf. the upper bound on $P(X_p\ge m+1)$ in (1).
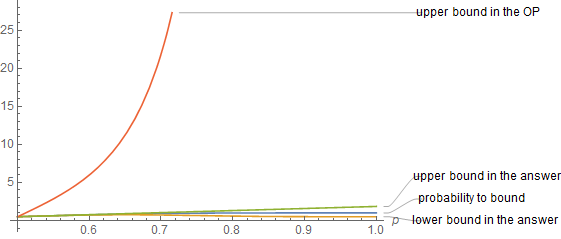
Graphs of the probability $P(X_p\ge m+1)$ to bound, of the upper and lower bounds on it given in this answer, and of the upper bound conjectured in the OP (as functions of $p$) are shown in the picture below for $m=5$:
