Does there exist a discrete gauge theory as a TQFT detecting the figure-8 knot?

Question

My question: Does there exist a discrete gauge theory as TQFT detecting the figure-8 knot?

By detecting, I mean that computing the path integral (partition function with insertions of the knot/link configuration from line operators of TQFTs), and there is a nontrivial expectation value $$ Z=<\text{knot/link configuration}> \neq \# \exp{(i \theta)}. $$ A nontrivial expectation value means that the $\theta$ is not 0 mod 2 \pi. The number $\#$ depends on the global topology of the spacetime which the link/knot is evaluated at, such as a 3-sphere.

If the answer is negative, is there a way to prove such a discrete gauge TQFT detecting the figure-8 knot make NON-sense?

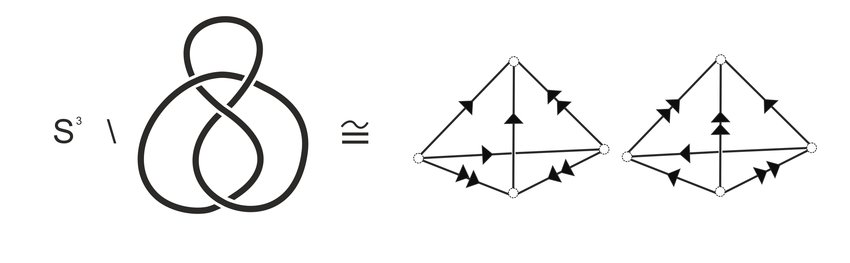
Background info: One of the best-studied complement space of 3-sphere is that of the figure eight knot, or Listing’s knot, say this space is called M8 (in Thurston book). The analysis is facilitated by the simplicity of the combinatorial structure of M8: it is the union of two tetrahedra, with a corresponding combinatorial decomposition of its universal cover being the tessellation of hyperbolic 3-space by regular ideal hyperbolic tetrahedra.

I know various literature using the discrete gauge theory (such as Dijkgraaf-Witten gauge theory and its generalization) as TQFT to detect their interesting corresponding link invariants. This approach from discrete gauge theory/TQFT (such as a $\mathbb{Z}_N$ gauge theory as level-$N$ $BF$with an action $S=\int_{M^3} BdA$ theory over 3-manifold $M^3$) makes up a nice list, a correspondence can be following:

from this article: Braiding statistics and link invariants of bosonic/fermionic topological quantum matter in 2+1 and 3+1 dimensions

Annals of Physics Volume 384, September 2017, Pages 254-287

https://doi.org/10.1016/j.aop.2017.06.019

Back to My question: the above list of TQFTs as discrete gauge theory do not detect the figure-8 knot. Are there possible other discrete gauge theories as TQFT or more general TQFT can detect the figure-8 knot?

For example, we know the 3d SU(2)$_k$ Chern-Simons gauge theory can detect the figure-8 knot. But the 3d SU(2)$_k$ Chern-Simons gauge theory is a 3d TQFT but NOT a 3d discrete gauge theory.

Answer 1

Short answer: Untwisted Dijkgraaf-Witten theories with non-abelian gauge groups (e.g. $S_3$) distinguish most knots from the unknot and from each other.

Longer answer:

Here's my understanding of your question. (Please correct me if I've misinterpreted it.) For any 3d TQFT, the path integral $Z(M)$ of a 3-manifold $M$ should give a vector in the Hilbert space $H(\partial M)$ of the boundary $\partial M$. Given 3-manifolds $M_1$ and $M_2$ with the same boundary $Y$, we say that the TQFT distinguishes $M_1$ from $M_2$ if $Z(M_1) \ne Z(M_2)$ as vectors in $H(Y)$. You are looking for a discrete gauge theory (e.g. untwisted DW theory) which distinguishes the complement of the figure-8 knot from the complement of the unknot, both of which have boundary the torus $T^2$. (Both knots must be framed in order to identify the boundaries of their complements with $T^2$.)

For an untwisted DW theory with group $G$, one basis of the Hilbert space $H(Y)$ is the set of group homomorphisms $\rho: \pi_1(Y) \to G$, modulo group conjugation. (So when $Y = T^2$, this is the set of $(m, l) \in G\times G$ such that $mlm^{-1}l^{-1} = 1$, modulo the relation $(m, l) \sim (g^{-1}mg, g^{-1}lg$.)

Let $\partial M = Y$. The component of $Z(M)$ at the basis vector corresponding to a homomorphism $\rho : \pi_1(Y) \to G$ is equal to the number of extensions of $\rho$ to $\rho':\pi_1(M) \to G$, each counted with weight $1/r$, where $r$ is the number of elements in the stabilizer of $\rho'$ (group elements $g$ such that $\rho'$ conjugated by $g$ is equal to $\rho'$).

In particular, for a framed knot $K$ in $S^3$, and for $G$ the symmetric group $S_k$, the DW path integral $Z(S^3 \setminus ndb(K))$ determines the number of homomorphisms from $\pi_1(S^3 \setminus ndb(K))$ to $S_k$ such that the meridian is taken to the transposition $(1,2)$ and the longitude is taken to an element which does not commute with $(1,2)$.

A simple calculation shows that the above homomorphism-counting invariant distinguishes the figure-8 knot from the unknot for $k=3$.

More generally, in the early, pre-Jones-polynomial days of knot tabulation, one of the most powerful tools for distinguishing knots from each other was to count homomorphisms of $\pi_1(S^3 \setminus ndb(K))$ to $S_k$ for small values of $k$ (e.g. 3, 4, 5). It was observed that these invariants nearly always distinguished non-equivalent knots. It follows that untwisted DW TQFTs do a very good job of distinguishing knots.

A good reference for untwisted DW theories is a paper by Freed and Quinn from the early 1990s. http://de.arxiv.org/abs/hep-th/9111004 .