I'm reading the introduction of An Introduction to the Trace Formula by James Arthur and wanted to understand something in the introduction.
Let $H$ be a unimodular locally compact Hausdorff group, and $\Gamma$ a discrete subgroup of $H$. Let $\mathscr H = L^2(\Gamma \backslash H)$ be the Hilbert space of measurable functions $\phi: \Gamma \backslash H \rightarrow \mathbb C$ satisfying $||\phi||^2 = \int\limits_{\Gamma \backslash H} |\phi(h)|dh < \infty$.
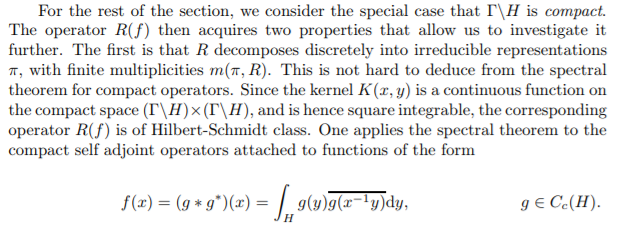
Assume $\Gamma \backslash H$ is compact. Fix $f \in C_c^{\infty}(H)$, and let $K \in L^2(\Gamma \backslash H \times \Gamma \backslash H)$ be the function defined by
$$K(x,y) = \sum\limits_{\gamma \in \Gamma} f(x^{-1}\gamma y)$$ which is a finite sum. To this kernel we can associate a compact operator $R(f)$ on $\mathscr H$ defined by
$$[R(f)\phi](x) = \int\limits_{\Gamma \backslash H} K(x,y)\phi(y)dy$$
It can be shown that this integral is equal to just $\int\limits_H f(y)\phi(xy)dy$.
Arthur arranges that $R(f)$ is a compact self adjoint operator, and claims the decomposition of $\mathscr H$ into a Hilbert space direct sum of irreducible subrepresentations (under the action of $H$ by right translation) follows from the spectral theorem for self adjoint operators. How does this follow?