I believe I may have a counterexample to Theorem 5.3.1 on page 179 from the book book Discriminants, Resultants and Multidimensional Determinants by Gel'fand, Kapranov, and Zelevinsky. To summarize the notation (see the section "Notation from the book" below for more details):
- $A$ is finite;
- $X_A$ is the (not necessarily normal) projective toric variety induced by $A$;
- $S$ is the affine semigroup generated $A\times \{1\}$ in $\Xi=\Bbb Z^k$;
- $S/\Gamma$ is the image of $S$ in $\Xi/(\Xi \cap \operatorname{Lin}_\Bbb R(\Gamma))$, where $\operatorname{Lin}_{\Bbb R}(\Gamma)$ denotes the $\Bbb R$-linear span of $\Gamma\times\{1\}$; and
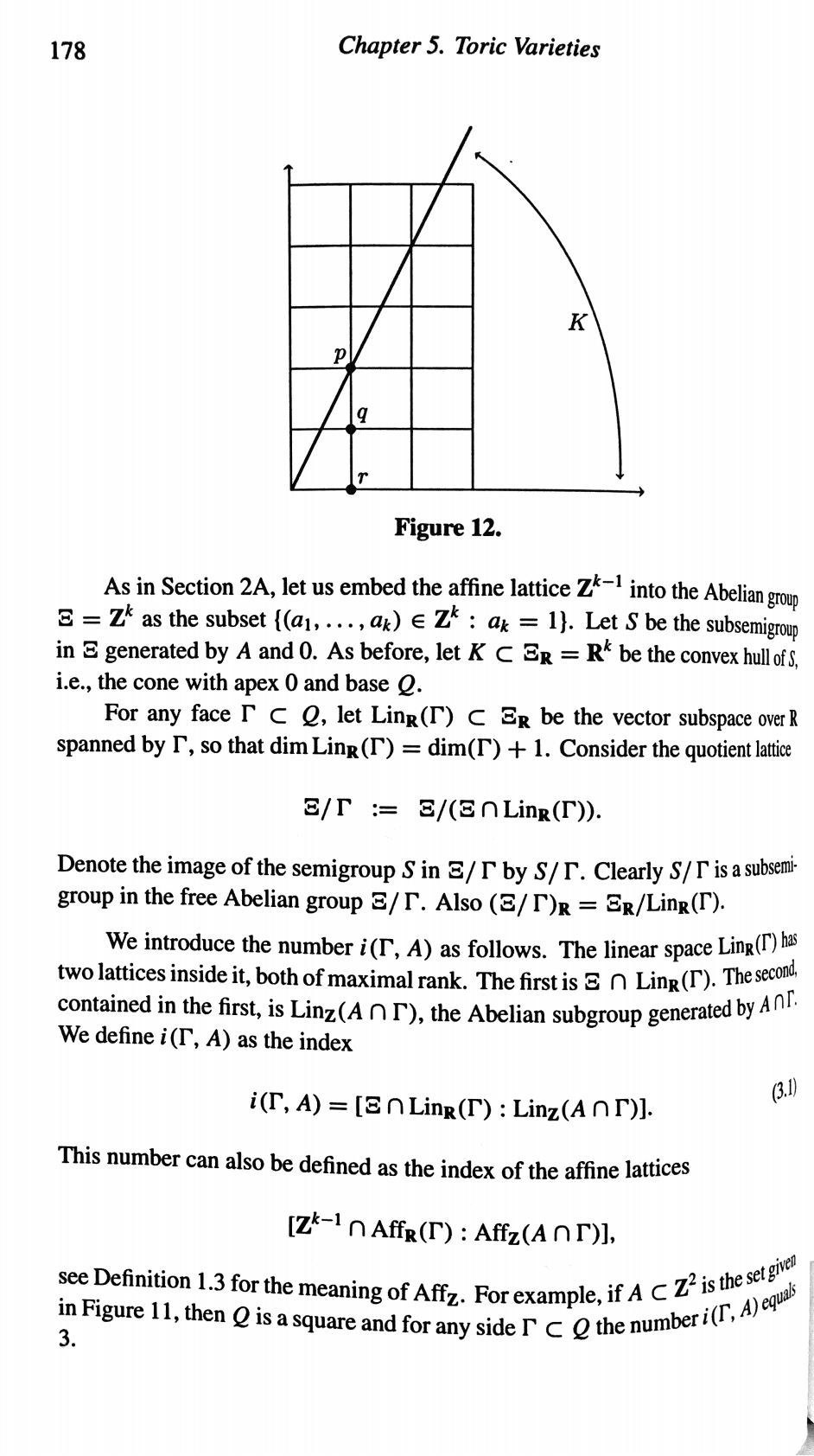
- $i(\Gamma, A) = [\Xi\cap \operatorname{Lin}_\Bbb R(\Gamma) : \operatorname{Lin}_\Bbb Z (A\cap \Gamma)]$.
Here's the theorem in question:
The only part of the theorem that I have issue with is the final sentence, and I believe a counterexample to this part.
Counterexample?
Let \begin{equation*} A = \begin{bmatrix} 1 &0 &0 &1 &0\\ 0 &1 &0 &1 &0\\ 0 &0 &2 &1 &0\\ 1 &1 &1 &1 &1 \end{bmatrix} \subseteq \Xi, \qquad \Gamma = \operatorname{conv}(\omega^{(3)},\omega^{(5)}). \end{equation*} (I'm identifying $A$ with the set of its columns). Note that each column of $A$ is a vertex of $Q=\operatorname{conv}(A)$, and $Q$ is a simplicial polytope. Hence, $\Gamma$ is indeed a face of $Q$.
Claim: The local structure of $X_A$ along the orbit $X^0(\Gamma)$ corresponding to $\Gamma$ is two copies of $\operatorname{Spec}(S/\Gamma) \times X^0(\Gamma)$ glued along a subvariety of dimension $2=1 + \dim(X^0(\Gamma))$. This contradicts the claim in the Theorem that the two copies of $\operatorname{Spec}(S/\Gamma)$ are glued along $\{0\}\times X^0(\Gamma)$.
Proof. I'm going to start along a similar route as in the proof given in the book. Let $Y_A=\operatorname{Spec}\Bbb C[S]$---this is the cone over $X_A$. Let $Y^0(\Gamma)\subseteq Y_A$ be the cone over the orbit of $X^0(\Gamma)$. As the book states, "[t]he local structure of $Y_A$ along $Y^0(\Gamma)$ is the same as that of $X_A$ along $X^0(\Gamma)$ so we shall study $Y_A$."
Outline of proof: We first show that the local structure of the orbit $Y^0(\Gamma)$ near the distinguished point is two copies of $\Bbb C^2\times Y^0(\Gamma)$ glued along a subvariety of dimension $3=1 + \dim(Y^0(\Gamma))$. We then show that $\operatorname{Spec}(S/\Gamma)\cong \Bbb C^2$.
The proof itself: The toric ideal of $A$ is $I_A= (x^2y^2z - u^2v^3)\subseteq \Bbb C[x,y,z,u,v]$. Let $P$ be the distinguished point of the orbit $Y^0(\Gamma)$ in $Y_A$ corresponding to $\Gamma$ ($P$ is called $y_0$ in the book). Let $$ U = \operatorname{Spec}\Bbb C[\operatorname{Lin}_\Bbb Z(A\cap \Gamma) + S] = \operatorname{Spec}(\Bbb C[x,y,z^\pm,u,v^\pm]/(x^2y^2z - u^2v^3))$$ be the smallest torus-invariant open subset of $Y_A$ containing $Y^0(\Gamma)$ (in the book, $U$ is called $Z$). Consider the projection $$p\colon U \to Y^0(\Gamma)$$ induced by the inclusion $\operatorname{Lin}_\Bbb Z(A\cap \Gamma)\hookrightarrow \operatorname{Lin}_\Bbb Z(A\cap \Gamma) + S$. We have \begin{align*} p^{-1}(P) &= \operatorname{Spec} \bigl(\Bbb C[\operatorname{Lin}_\Bbb Z(A\cap \Gamma) + S]/(z-1, v-1) \bigr)\\ &= \operatorname{Spec} \bigl(\Bbb C[x,y,z^\pm,u,v^\pm]/(z-1, v-1, x^2y^2z - u^2v^3)\bigr)\\ &\cong \operatorname{Spec} \bigl(\Bbb C[x,y,u]/(x^2y^2-u^2)\bigr)\\ &= V(xy-u)\cup V(xy+u) \subseteq \Bbb C^3. \end{align*} (Note that $V(xy-u)\cong V(xy+u)\cong \Bbb C^2$). In addition, $$V(xy-u)\cap V(xy+u) = V(xy-u, xy+u) = V(xy,u) = V(x,u)\cup V(y, u)$$ is the union of two lines---in particular, it has dimension greater than 0. So, $p^{-1}(P)$ is the union of two irreducible varieties glued along a variety of dimension greater than 0.
We now show that these two varieties are isomorphic to $\operatorname{Spec} \Bbb C[S/ \Gamma]$. Because $V(xy-u)\cong V(xy+u)$, it suffices to show that $\Bbb C[S/\Gamma] \cong \Bbb C[x,y,u]/(xy-u)$.
By Macaulay2, we have (with $t$ corresponding to the point $(0,0,1,1)$) \begin{align*} \Bbb C[S + \Xi\cap \Bbb R_{\geq0}(\Gamma\times \{0\})] &= \Bbb C[x,y,z,u,v,t]/(t^2-zv, tuv-xyz, txy-uv^2). \end{align*} Then \begin{align*} \Bbb C[S/\Gamma] &= \Bbb C[S + \Xi \cap \operatorname{Lin}_\Bbb R(\Gamma)]/(z-1, v-1, t-1)\\ &= \Bbb C[x,y,z^\pm, u, v^\pm, t^\pm]/ (t^2-zv, tuv-xyz, txy-uv^2, z-1, v-1, t-1)\\ &= \Bbb C[x,y,u]/(u-xy). \qquad\qquad \Box \end{align*}
Notation from the book
Here are pictures of the relevant notation from the book. The only things missing is the definition of $X_A$ and $X^0(\Gamma)$. If $A=\{\omega^{(1)},\ldots,\omega^{(n)}\}$, then $X_A$ is the closure in $\Bbb P^{n-1}$ of the set
$$ X_A^0 = \{(x^{\omega^{(1)}},\ldots,x^{\omega^{(n)}}) : x = (x_1,\ldots,x_{k-1})\in (\Bbb C^*)^{k-1}\},$$
and
$$ X^0(\Gamma) = \{(z_1,\ldots,z_n) \in X_A : z_i=0 \text{ iff }\omega^{(i)}\notin \Gamma\}.$$
