10
$\begingroup$

Consider a Riemannian manifold $\Sigma$ of dimension two homeomorphic to a torus. When is there a non-closed geodesic on $\Sigma$ which does not intersect itself — are there reasonable necessary or sufficient conditions for this?

(This is a reference request. My guess is that this question does not have an amazing answer, but I am curious if it was considered in the literature. By the way, a flat torus obviously has this property, but I am interested in a more general setting.)

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ If you look at the flat torus $\mathbb{R}^2 / \mathbb{Z}^2$ than any line with an irrational slope is a non closed geodesic without intersections. My suspicion would be that the situation is the same for any other metric on the torus. That is starting at a point, there is an infinite density zero set of directions that leads to closed geodesics and for all other directions the geodesic is non-closed (fairly sure) and has no self intersections (somewhat sure). $\endgroup$
    – quarague
    Commented Jan 23, 2019 at 9:49
  • 2
    $\begingroup$ @quarague, what if you stick a toothpick in a donut, and smooth over the result with icing? The surface is still a torus, but geodesics which wind up the toothpick one way may well intersect themselves on the way down. Since almost any geodesic will hit the toothpick multiple times, that seems to be a metric where almost every geodesic will self-intersect. $\endgroup$
    – user44143
    Commented Jan 23, 2019 at 10:56
  • $\begingroup$ @MattF. That is an interesting example. I was more picturing a donut with a few small bumps where the kind of intersection you described does not occur. Intuitevely you need fairly high curvature locally around a bump to get a geodesic with a self intersection through winding around the bump. I wonder whether one can make this into a rigirous argument with a curvature bound. Looks like the problem is more complicated than I originally thought. $\endgroup$
    – quarague
    Commented Jan 23, 2019 at 11:42
  • 2
    $\begingroup$ Any small bump will get hit infinitely often, and each time it will turn the geodesic a little (from the direction it would have had without the bump), so I would guess that small bumps will cause the typical geodesic to have infinitely many self intersections. $\endgroup$
    – Ben McKay
    Commented Jan 23, 2019 at 12:39

1 Answer 1

Reset to default
9
$\begingroup$

This is more of a comment intended to provide examples. One can construct a reasonably large class of examples of metrics on $T^2$ satisfying your property. I believe moreover that this class of metrics (described below) is stable with respect to small $C^{\infty}$ perturbation.

Infinite geodesics on surfaces without self intersections are closely connected to geodesic lamintations. Now, hyperbolic surfaces have plenty of geodesic laminations. So we can do the following. First take a hyperbolic metric on $T^2$ with a cusp and then smoothen the cusp close to infinity. Then there still will be a geodesic lamination in the hyperbolic part of $T^2$. For a concrete example, look at Fig 11 here: (Geodesic lamination on surfaces by Bonahon)

https://www-bcf.usc.edu/~fbonahon/Research/Preprints/StonyBrookProc.ps

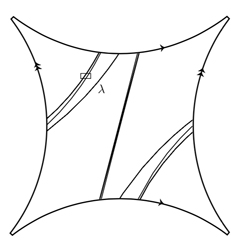          Fig11
          Fig.11. A geodesic lamination on the punctured torus.

Or one can use a different construction, identifying a part of $T^2$ with a part of hyperbolic surface of genus $2$ as on Fig 5 in the same text. In this case one will get in infinite geodesic in $T^2$ that accumulates to two closed geodesics on both ends.

          Fig5

I believe that both examples are stable under small perturbation of the metric.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you, this may be helpful. $\endgroup$
    – Alex Gavrilov
    Commented Jan 29, 2019 at 5:38

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .