4
$\begingroup$

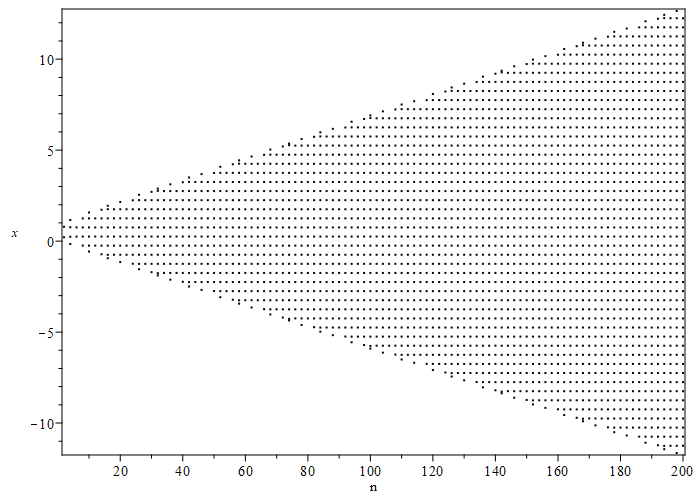
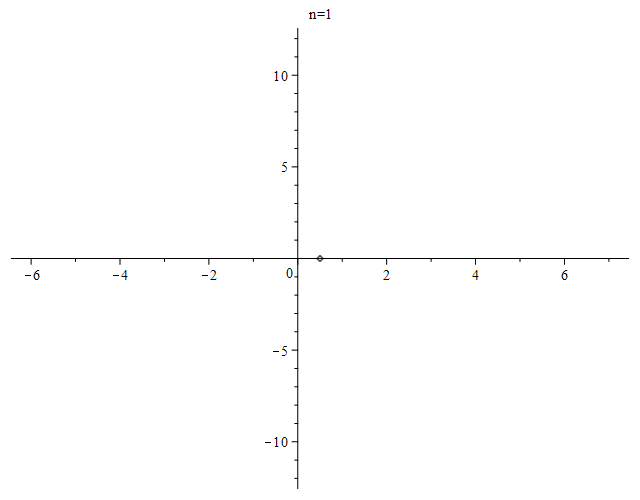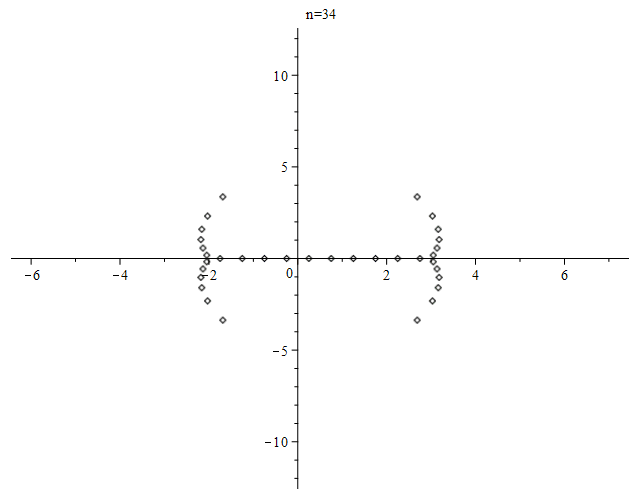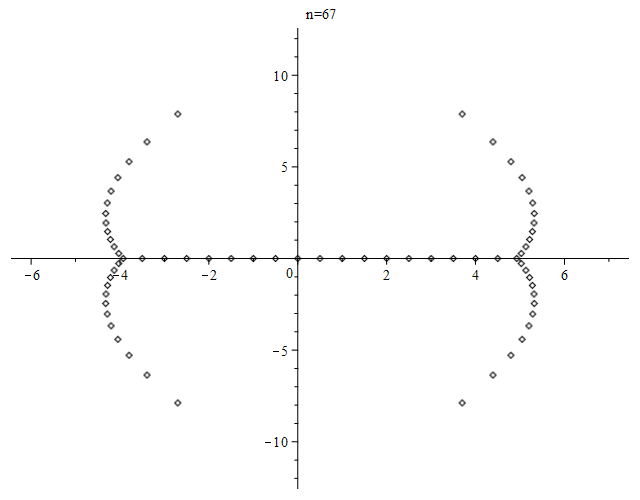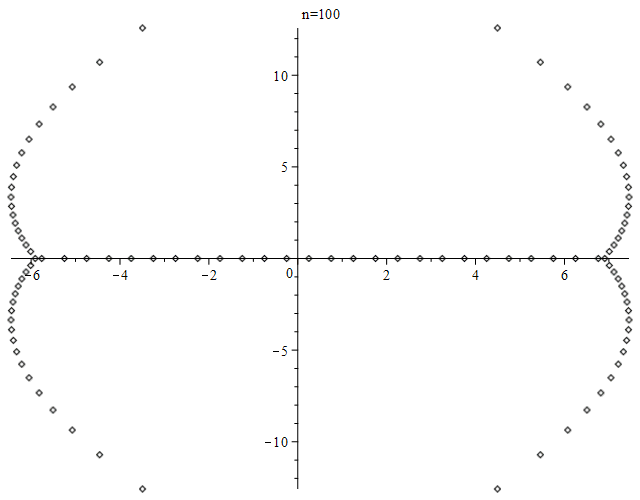Consider the Bernoulli polynomials denoted by $B_n(z)$. Now, start plotting the set of all (combined) complex roots $\mathcal{A}_N$ of $B_n(z)$, say for $n=1,2,\dots,N$ for some enough large $N$. It appears that $\mathcal{A}_N$ branches into several "curves".

QUESTION: Or, does it? If so, what are these curves?

Request: Can someone post the complex plot here?

Improve this question
$\endgroup$
2

1 Answer 1

Reset to default
12
$\begingroup$

Here is an animation of the zeros of the first $100$ Bernoulli polynomials, produced using Maple.

enter image description here

For the number of real roots, see OEIS sequence A094937 and references there.

EDIT: As requested by Wolfgang, here is a plot of the real roots for even $n$ up to $200$.

enter image description here

Improve this answer
$\endgroup$
4
  • $\begingroup$ Many thanks for the quick and generous response to my request for the plots. $\endgroup$
    – T. Amdeberhan
    Commented Jan 23, 2019 at 4:44
  • $\begingroup$ That is nice! It looks like the real roots essentially oscillate between even and odd degrees. Could you add a plot only for, say, even n? $\endgroup$
    – Wolfgang
    Commented Jan 26, 2019 at 15:31
  • $\begingroup$ Very nice! Can share the code, please? Put it here or link to github or like that... $\endgroup$
    – Alexander Chervov
    Commented Jan 27, 2019 at 14:41
  • $\begingroup$ plots[display]([seq(plots[pointplot](map([Re,Im],[fsolve(bernoulli(n,z),z,complex)])),n=1..100)],insequence=true); plots[pointplot]([seq(op(map(t -> [n,t],[fsolve(bernoulli(n,z),z)])),n=2..200,2)],symbol=point,axes=box); $\endgroup$
    – Robert Israel
    Commented Jan 27, 2019 at 16:45

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .