I've been very positively impressed by Tristan Needham's book "Visual Complex Analysis", a very original and atypical mathematics book which is more oriented to helping intuition and insight than to rigorous formalization. I'm wondering if anybody knows of other nice math books which share this particular style of exposition.
$\begingroup$
$\endgroup$
5
-
9$\begingroup$ You SHOULD be impressed,Marco. It's a truly remarkable book that should be read by anyone seriously interested in analysis or physics,particularly for it's historical insight. $\endgroup$– The MathemagicianCommented Jul 14, 2010 at 19:11
-
19$\begingroup$ On one hand I want to +1 because that's indeed a great book. On the other hand I should probably -1 because it's a bad question (well, at least as long as it's phrased the way it is and since it's not community-wiki). So I'll do neither for now. I'll just leave this comment: while many books come to mind, one particular author who has a style similar to that of Needham's (that is, his books are full of intuition and historical insight) is John Stillwell. I'm not going to recommend a particular book because most of them are great (I say 'most' and not 'all' because I haven't read them all). $\endgroup$– danseeteaCommented Jul 14, 2010 at 19:22
-
8$\begingroup$ @danseetea The similarity between the texts of Stillwell and Needham's masterwork is indeed striking and is not an accident:Professor Stillwell is acknowledged in the introduction to Needham's book as a strong influence on the style of the text. We can all learn a great deal from Professor Stillwell's texts,emphasizing the historical development of the great edifice of mathematics. $\endgroup$– The MathemagicianCommented Jul 14, 2010 at 22:32
-
$\begingroup$ @Andrew L: Does that mean that Needham's text is useful for the student who has not yet understood the rigorous formalism of complex analysis and wants to understand it? Or should a student who already understands complex analysis reasonable well still read Needham's book? $\endgroup$– David CorwinCommented Jul 15, 2010 at 10:36
-
5$\begingroup$ @Davidac897 Both,ideally.A student struggling with a more rigorous presentation-such as Alfhors or Narisham-will benefit tremendously from the geometric and historical presentation of the basics and drawing connections between them. A student who has mastered a rigorous presentation will gain much deeper insight into what the abstract construction "means". $\endgroup$– The MathemagicianCommented Jul 15, 2010 at 19:35
Add a comment
|
44 Answers
$\begingroup$
$\endgroup$
4
John Stillwell's recent book Naive Lie Theory is amazing and in a similar vein. It provides great geometrical intuition for many of the common matrix groups. What is particularly impressive about this book is how he motivates more complicated ideas, such as maximal tori, in a very elementary fashion. It is perfect for undergrads looking for a good introduction.
-
8$\begingroup$ I would just like to second this opinion! There are several concepts I never entirely understood until a single sentence of Stillwell's--perfectly phrased--cleared years of fog. Understanding how Lie brackets connect to the conventional cross-product is one such "Oh!" moment. $\endgroup$ Commented Jul 14, 2010 at 20:48
-
2$\begingroup$ Agreed. The historical notes at the end of the book are particularly enlightening. $\endgroup$ Commented Jul 15, 2010 at 1:22
-
1$\begingroup$ Absolutely agreed. I also had the sheer pleasure of being lectured by John Stillwell when he was here in Australia in the early to mid 1990s: see my answer on Stillwell's "Classical Topology and Combinatorial Group Theory”. $\endgroup$ Commented May 23, 2011 at 7:13
-
1$\begingroup$ Re @JosephO'Rourke's comment, nothing about this book, but I have always liked a remark of Howe's that, much like an abstract group can be very loosely thought of as many cyclic subgroups fit together, a Lie group can be very loosely thought of as many one-parameter subgroups fit together. $\endgroup$– LSpiceCommented Jun 2, 2022 at 3:26
$\begingroup$
$\endgroup$
3
I share your admiration for Needham's book!
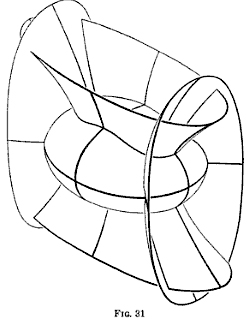
One of my favorites is Geometry and the Imagination by David Hilbert and Stephan Cohn-Vossen.
Some of their figures are stunning, almost works of art, and of course all drawn before computers!
Here they are explaining how one ellipsoid, one hyperboloid of one sheet, and one hyperboloid of two sheets, pass through any point in space:
-
2$\begingroup$ The original German edition of the book is true marvel to behold (I own a Russian translation and have used AMS translation, but they are not in the same league). $\endgroup$ Commented Jul 15, 2010 at 1:15
-
9$\begingroup$ What I find mind boggling about mathematics and physics of times gone by is the staggering ability of people to draw, graph and visualise WITHOUT COMPUTERS. They either did it with superb insight or with an almost soul-crushing slog of work - both to be admired. My day job is optical engineering and I am putting together a Lie theory text in my spare time - I truly believe that the only significant (but absolutely fundamental) way my jobs would differ without computers is that I would be lost without the ability to graph and visualise that they give .... $\endgroup$ Commented May 23, 2011 at 6:42
-
7$\begingroup$ .... (Comment continued) Think of Abramowitz and Stegun - all those graphs drawn by hand. Or, there is a plot of the radiation pattern from a Mie scattering sphere in Born and Wolf, Principles of Optics. It has exquisitely fine structure in the plot, which was all done by hand calculation. I had to write a Mie scattering software function once, and I used Born and Wolf's hand calculations to debug my work - the hand calculations were right and I was wrong! It should have been the other way around!! $\endgroup$ Commented May 23, 2011 at 6:45
$\begingroup$
$\endgroup$
I've just been reading "Visual Group Theory" by Nathan Carter. The similarity of title to Needham's may be coincidence, but the book has exactly the same effect: it SHOWS you WHY all these things are true, when conventional proofs so often just TELL you WHAT is true.
$\begingroup$
$\endgroup$
2
Tristan Needham has a new book coming out in 2021:
Visual Differential Geometry and Forms: A Mathematical Drama in Five Acts. Princeton link.
-
-
1$\begingroup$ One of the most beautiful books ever. I sleep with it by my side. $\endgroup$– BrianCommented Dec 8, 2021 at 10:16
$\begingroup$
$\endgroup$
5
"Mathematical Methods of Classical Mechanics" and "Ordinary differential equations" by late V.I. Arnold. In my opinion, these are THE books for anyone who wants to understand geometric theory of ODEs. I agree with Andrew's comment though, that the books might be a difficult read for an undergraduate (particularly, the first one).
My pick for Fourier analysis is, well, "Fourier analysis" by T.W. Körner. Very pedagogical, with lots of historical sections and nice illustrations. And it is probably more in the spirit of Needham's book than the books by Arnold.
-
1$\begingroup$ I totally agree,but these books are considerably harder then Needham's. But if you're serious about understanding the deeper aspects of the theory of ODE's,they are a necessity. Indeed-all Arnold's book are a testament to one of the great mathematicians and teachers of all time and his awesome perspective unifying both mathematics and physical sciences through the shared language of geometry. $\endgroup$ Commented Jul 14, 2010 at 22:34
-
4$\begingroup$ "Ordinary Differential Equations" is the only one I have read, but it is among the best math books I've come across. I wouldn't have called it difficult, though -- Arnold's style makes it a comfortable read for most graduate students, I'd say. (It actually makes quite nice "pleasure reading" when you want a break from more strenuous stuff.) $\endgroup$ Commented Jul 15, 2010 at 3:05
-
$\begingroup$ @Tom Yes,for GRADUATE students with very good command of linear algebra and basic analysis,yes,I agree. But an undergraduate-unless they're superior-will find them pretty tough.Especially the Classical Mechanics text,which is clearly a graduate level text by anyone's measure. $\endgroup$ Commented Jul 15, 2010 at 8:22
-
-
1$\begingroup$ I believe that Arnold's textbook on ODE is oriented to undergrads. I read it when I first learn ODE when I was a sophomore. Arnold's lecture notes on PDE seem more demanding, which covers symplectic structures at the beginning. $\endgroup$– user20948Commented Jan 17, 2020 at 21:20
$\begingroup$
$\endgroup$
3
My favorite along with the Visual Complex Analysis by Tristan Needham
is Grad, Div, Curl and all that by H. M. Schey
NOTE: Alice's Adventures in Wonderland by Lewis Carroll is still the best mathematical book I have ever read.
-
4$\begingroup$ Re Alice's Adventures, you may want to take a look at bl.uk/onlinegallery/ttp/alice/accessible/introduction.html $\endgroup$ Commented Jul 14, 2010 at 19:53
-
$\begingroup$ @Andres Caicedo: Great link! Thanks! $\endgroup$ Commented Jul 14, 2010 at 20:41
-
$\begingroup$ This is an engineering book, not a mathematics book. $\endgroup$– BrianCommented Apr 29, 2023 at 0:33
$\begingroup$
[![alt text][1]][1]
$\endgroup$
4
"Indra's Pearls: The Vision of Felix Klein" by David Mumford, Caroline Series and David Wright.
Most certainly visual, this book is not in the same category as most of the books mentioned so far. In fact, it defies categorization at all because it is a combination of an amazing ground level introduction to group theory and a monograph on Shottky groups, which grew out of desire to find mathematics to go along with stunning computer graphics. And it has outstanding cartoons by Larry Gonick.
[![alt text][1]][1]
1:
-
$\begingroup$ I wasn't able to find the picture of the front cover to embed (and David Wright's website seems to be down). If you know where to get one, please, put it in! $\endgroup$ Commented Jul 15, 2010 at 1:06
-
-
$\begingroup$ Great, thank you very much, Joseph! $\endgroup$ Commented Jul 15, 2010 at 2:06
-
$\begingroup$ It certainly catches the eye and made me discover this great book ,thank you! $\endgroup$ Commented Feb 18, 2015 at 18:03
$\begingroup$
$\endgroup$
3
John Stillwell's "Classical Topology and Combinatorial Group Theory (Springer-Verlag Graduate Texts in Mathematics)". See also the answer on his "Naive Lie Theory".
I also had the sheer pleasure of being lectured by John Stillwell when he was here in Australia in the early to mid 1990s. I took four of his courses in all, in general group and Galois theory as well two topics (topology and Riemann Surfaces) which were very much subtopics of the above book. I hope he wouldn't mind my saying that his gift for explanation did not appear magically: sheer hard work was evident in his lecture notes and he gave me the impression of someone never happy with an explanation as it was, he was always striving for a simpler and cleaner one for everything he lectured. Perhaps a mathematical analogue of Richard Feynman as a teacher. In his Galois theory lectures I and a few other students were lucky enough to join him as fellow learners: he was still getting his lectures straight and, in his honest way, warned us that this would be the case. So we "read" Emil Artin's "Galois Theory" together. Thus I got to see first hand the staggering amount of work he put into building his explanations.
-
3$\begingroup$ In his own words: "I read the books of Edwards, Tignol, Artin, Kaplansky, MacLane and Birkhoff and Lang, taught a course on Galois theory, and then discarded 90% of what I had learned." - from 'Galois Theory for Beginners'. $\endgroup$ Commented Sep 1, 2013 at 11:26
-
2$\begingroup$ I believe John Stillwell may be the single greatest author of mathematical textbooks that's currently active.He's going to leave behind a remarkable legacy of such textbooks.His topology and geometry textbooks alone would earn him this legacy,but he's written so many more such jewels. Let's hope he produces many more. $\endgroup$ Commented Apr 22, 2016 at 4:36
-
2$\begingroup$ @TheMathemagician Have you seen this: youtube.com/watch?v=9MV65airaPA. It's a wonderful talk in many ways, but one of the many things that really caught my attention was his beautiful one sentence description of induction to the lay person in the first few minutes. My daughter of ten grasped it in an instant after she had been reading about induction and had tried to learn more from me. His sentence drew the comment from her, "Wow, this guy is a way better stuff-explainer than you, Papa". $\endgroup$ Commented Apr 22, 2016 at 23:22
$\begingroup$
$\endgroup$
1
I haven't read Needham's book so I'm not totally sure what you mean, but it sounds like you might like "Three-Dimensional Geometry and Topology" by Bill Thurston and Silvio Levy.
-
$\begingroup$ I think it is far from didactic though trying to be clear but hard. Nothing to compare with the MO book. $\endgroup$ Commented Jul 9, 2020 at 11:47
$\begingroup$
$\endgroup$
A Panoramic View of Riemannian Geometry by Marcel Berger.
It gets into quite advanced and sometimes technical topics, but geometric intuition is always at the fore. Lots of great pictures! It must be impossible to read this book without getting passionately excited about differential geometry. Berger's other books on geometry are similarly outstanding, if more conventional.
$\begingroup$
$\endgroup$
Roger Penrose's The Road to Reality. Needham says in VCA that Penrose taught him what good style is.
$\begingroup$
$\endgroup$
Another book to try is Michio Kuga's Galois' Dream. It certainly has its own unique style (very playful) but I think its focus on intuition sets it apart from many other math books. Apparently it was quite a pop-culture hit in Japan!
$\begingroup$
$\endgroup$
1
Nonlinear dynamics and chaos by Steven Strogatz. Lots of pictures, intutive and clear explanations, interesting applications, great humor.
-
$\begingroup$ Yes! This is the book that to me comes closest to Visual Complex Analysis in spirit. $\endgroup$– dfanCommented Dec 8, 2015 at 16:28
$\begingroup$
$\endgroup$
A quite recent book is Advanced Calculus: A Geometric View by James Callahan. It is liberally illustrated and even contains a section on Morse's lemma in the chapter on critical points. Bear in mind, though, that the book is not intended for absolute novices to multivariable calculus. Familiarity with basic concepts such as partial derivatives is expected, as is some knowledge of linear algebra.
Edit: A review by William J. Satzer is available at http://www.maa.org/publications/maa-reviews/advanced-calculus-a-geometric-view.
$\begingroup$
$\endgroup$
1
It's not exactly as visual as Visual Complex Analysis, but Michael Spivak's A Comprehensive Introduction to Differential Geometry has a lot of the same appeal to intuition and conversational style. (Well, I've only read Volume 1, there's a total of 5, but if they're anything like other Spivak books I've read, this holds true of them as well).
-
5$\begingroup$ I like the artwork on the cover of these books! $\endgroup$ Commented Jun 16, 2011 at 21:32
$\begingroup$
$\endgroup$
0
David Bressoud's "Proofs and Confirmations: The Story of the Alternating-Sign Matrix Conjecture" is also wonderful. Emphasis on how the conjecture was proved, and its connections to many interesting areas of math.
$\begingroup$
$\endgroup$
1
A wonderful book which overviews a lot of these kinds of ideas is Glimpses of Algebra and Geometry by Gabor Toth. From the product description, "The purpose of Glimpses of Algebra and Geometry is to fill a gap between undergraduate and graduate mathematics studies. It is one of the few undergraduate texts to explore the subtle and sometimes puzzling connections between number theory, classical geometry and modern algebra in a clear and easily understandable style."
-
$\begingroup$ A very good book indeed. $\endgroup$ Commented Jul 16, 2010 at 2:16
$\begingroup$
$\endgroup$
1
The Shape of Algebra in The Mirrors of Mathematics by G. Katz and V. Nodelman
The Wild World of 4-Manifolds by Alexandru Scorpan
Discrete Differential Geometry: An Applied Introduction by Keenan Crane
Calculus Blue Multivariable Volume 4: Fields by Robert Ghrist
A Gateway to Number Theory: Applying the Power of Algebraic Curves by Keith Kendig
Conics by Keith Kendig
How Surfaces Intersect in Space: An Introduction to Topology by J. Scott Carter
As a complement to Needham, for superb visualizations and conceptual analysis: "Exploring Visualization Methods for Complex Variables" by Andrew J. Hanson and Ji-Ping Sha.
-
$\begingroup$ Geometry of Mobius Transformations: Elliptic, Parabolic, and Hyperbolic Actions of SL2(R) by Vladimir Kisil $\endgroup$ Commented Sep 3, 2022 at 19:59
$\begingroup$
$\endgroup$
1
If I may add my two cents, I would add two more books that are an integral part of my library, and which I have presently lent to a gifted middle school student. One is the 'shape of space' by Jeff Weeks, and the other is 'Symmetry of things' by John Conway
Jeff Weeks's book is an incredibly enjoyable account of the topology of 3-manifolds. I came across someone mentioning the late Bill Thurston's book in this post. While Thurston's book is definitely more rigorous, I would say that Weeks's book is an overlooked classic. His invitation to experiment with intuition to extrapolate to the abstract, and tying in a theoretician's mental forays with cosmological measurements is quite an eye-opener.
John Conway's book, on the other hand, while it showcases some ideas of symmetry through the work of some artists like Bathsheba Grossman, is largely about abstraction. It is a major work, the latter part technical enough to challenge and inspire mathematicians on the forefront of their field (in his words, not mine!).
-
$\begingroup$ Giving credit where credit is due, let's mention Conway's co-authors, Heidi Burgiel and Chaim Goodman-Strauss. Also, the full title is, The Symmetries of Things. goodreads.com/en/book/show/2376525 $\endgroup$ Commented May 15, 2023 at 3:12
$\begingroup$
$\endgroup$
Elementary Applied Topology by Robert Ghrist.
Book is full of interesting applications of ideas from topology/geometry. Good for adding some colour to a standard course.
$\begingroup$
$\endgroup$
A Singular Mathematical Promenade by Étienne Ghys is a perfect example in my opinion. The word singular in the title refers primarily to singularity theory, but the book is also singular in that its style is unique. Here is how the book begins.
In March 2009, I attended an administrative meeting and the colleague sitting next to me was even more bored than I was. Obviously Maxim Kontsevich had something else in his mind. Suddenly, he passed me a Parisian métro ticket containing a scribble and a single word: “impossible”. That was the new theorem he wanted to share with me! It took me a few minutes and some whispering before I could guess the statement of the theorem and a few more minutes to find the proof. Here is the statement.
Theorem. Four polynomials P1, P2, P3, P4 of a real variable x cannot satisfy
• P1(x) < P2(x) < P3(x) < P4(x) for small x < 0,
• P2(x) < P4(x) < P1(x) < P3(x) for small x > 0.
The relative position of the graphs of four real polynomials is subject to some constraints. I was fascinated: a new elementary result on four polynomials in 2009!
In the book, Ghys takes the reader on a leisurely tour through an astonishingly wide swath of mathematics, nominally with the goal of proving a generalization of the above theorem, but actually using that as an excuse to showcase many beautiful gems, including the resolution of singularities, the Hopf fibration, permutation pattern avoidance, the associahedron, the fundamental theorem of algebra, operads, Kontsevich's universal invariant for knots, and much more. The book is lavishly illustrated with diagrams in full color, and best of all, the PDF can be freely downloaded completely legally.
$\begingroup$
$\endgroup$
Roger Godement, Analysis, vols. I to IV (Springer). Contains many interesting historical, heuristic and motivational comments. Includes several details on Bourbaki ("bande militante") in Vol. III. Great mathematical content, plus some provocative thoughts.
$\begingroup$
$\endgroup$
Parallel Coordinates: Visual Multidimensional Geometry and its Applications by Alfred Inselberg
Has been praised by Stephen Hawking and others
https://www.amazon.com/Parallel-Coordinates-Multidimensional-Geometry-Applications/dp/0387215077
The barrier, imposed by our three dimensional habitation and perceptual experience, has been breached by this innovative and versatile methodology. There are beautiful visuals of multidimensional objects and insights into multidimensional problems: Air Traffic, Data Mining, Intelligent Process Control
$\begingroup$
$\endgroup$
1
David Bressoud's book Second Year Calculus: From Celestial Mechanics to Special Relativity is something like Needham's book. Both have an emphasis on history and applications.
-
4$\begingroup$ Speaking of Bressoud, the "history+applications" thread also runs through his other book "A Radical Approach to Real Analysis". It was truly a joy to peruse. $\endgroup$ Commented Aug 5, 2010 at 10:35
$\begingroup$
$\endgroup$
1
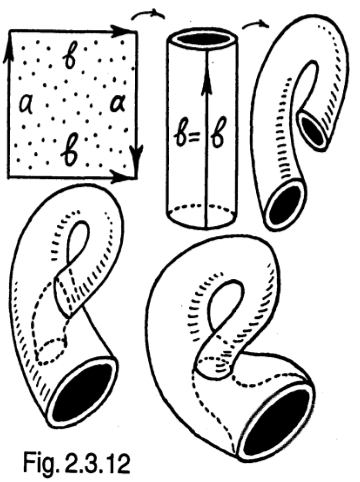
Visual Geometry and Topology by Anatoly Fomenko (co-authored the celebrated three-volume Modern Geometry Methods and Applications) is packed with epic illustrations like these:
- Klein bottle
- pretzel
-
3$\begingroup$ Fomenko has other illustrations too, and they are all fantastic! $\endgroup$ Commented Apr 26, 2022 at 14:49
$\begingroup$
$\endgroup$
Even more visual, even less formal, is "Dynamics, the Geometry of Behavior," by Ralph Abraham and Chris Shaw. I find the approach very useful for a difficult subject, however it needs to be supplemented with more rigorous material.
A digital edition can be purchased through Aerial Press http://www.aerialpress.com
$\begingroup$
$\endgroup$
I would recommend two books by David Bressoud
A Radical Approach to Real Analysis http://www.maa.org/press/books/a-radical-approach-to-real-analysis
A Radical Approach to Lebesgue's Theory of Integration https://books.google.no/books?id=TxxMoGjXC-wC&printsec=frontcover&source=gbs_ge_summary_r&cad=0#v=onepage&q&f=false
They are not as visual as the book by Needham, but like the books by Stillwell, they combine history and insight.
By the way, Needham and Stillwell both work at Univ. of San Francisco. I visted there once. Great place!
$\begingroup$
$\endgroup$
An Illustrated Introduction to Topology and Homotopy by Kalajdzievski, Sasho.
In my opinion this is a book hundred times better than Munkre's. It is similar to Needham in sense that it explains with pictures. Here are, for examples, some images for the proof of Urysohn's Lemma
Enjoy :D
$\begingroup$
$\endgroup$
I strongly recommend "The Essence of Chaos" by Edward N Lorenz. Not only considerable historical background, but a wonderful discussion of chaos, a unique and realistic model development and classic models. All without deep mathematics, but detailed so that one can program his model of a sled on a snow covered hill with moguls. A true classic that should be on every book shelf (after having read it in depth!)
$\begingroup$
$\endgroup$
if you are interested in dynamical systems/oscillators/differential equations, Pikovsky's Synchronization: A Universal Concept in Nonlinear Sciences is very well-written.
