Since 2013, I found Some problems on Apollonian Gasket as following. These problem also is higher level of Eppstein Point. I am looking for a proof of one of these problems:
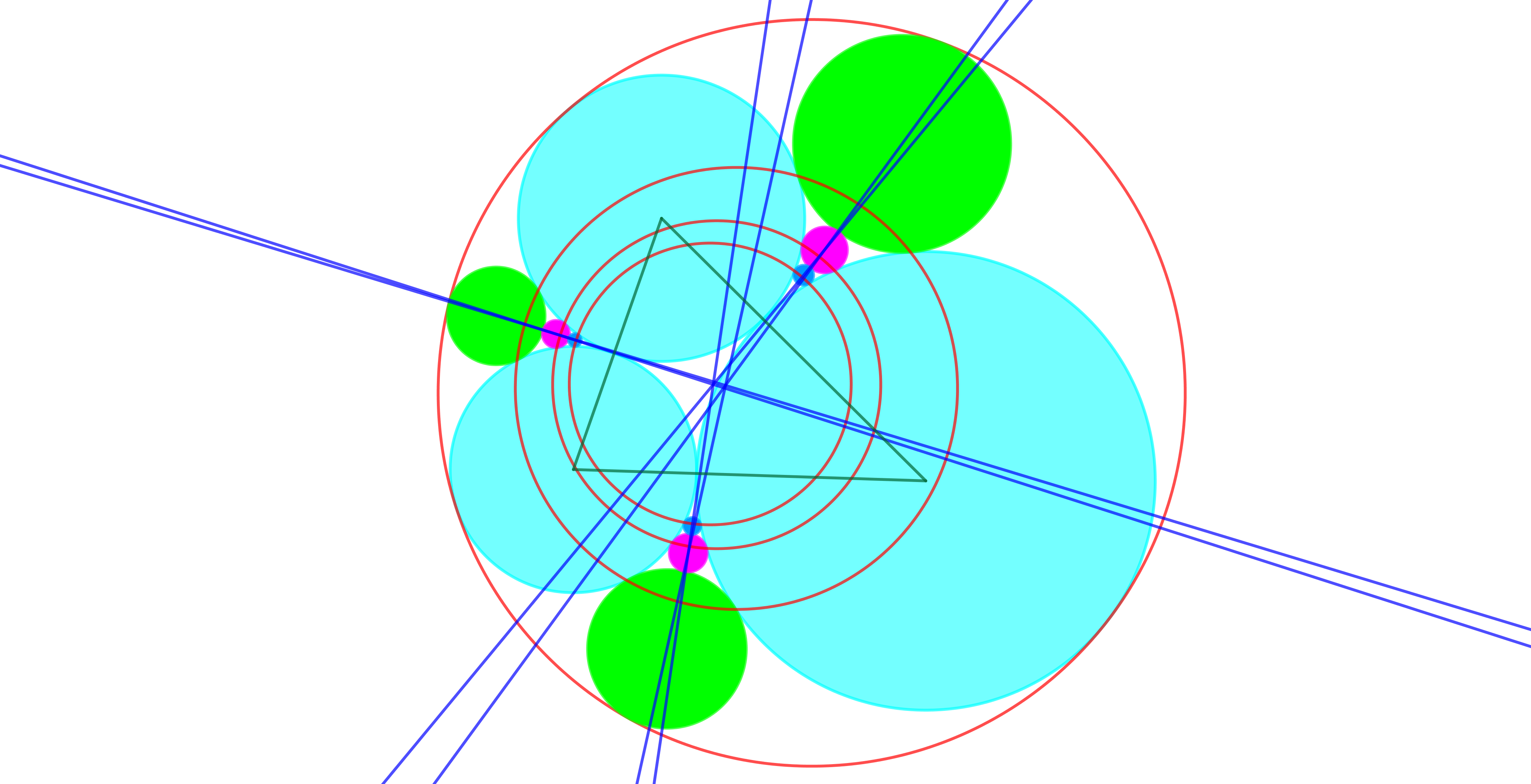
Let three $(A)$, $(B)$, $(C)$ circle (cyan circles) are tangent together and tangent with $(G)$ and $(K)$. Two circles $(G)$, $K$ are Soddy circles of triangle $\triangle ABC$
Construct three circle $(M_{11})$, $(M_{21})$, $(M_{31})$ (blue)
$(M_{11})$ is tangent $(B)$ and $(C)$ and $(G)$ (or $(K)$)
$(M_{21})$ is tangent $(A)$ and $(C)$ and $(G)$ (or $(K)$)
$(M_{31})$ is tangent $(A)$ and $(B)$ and $(G)$ (or $(K)$)
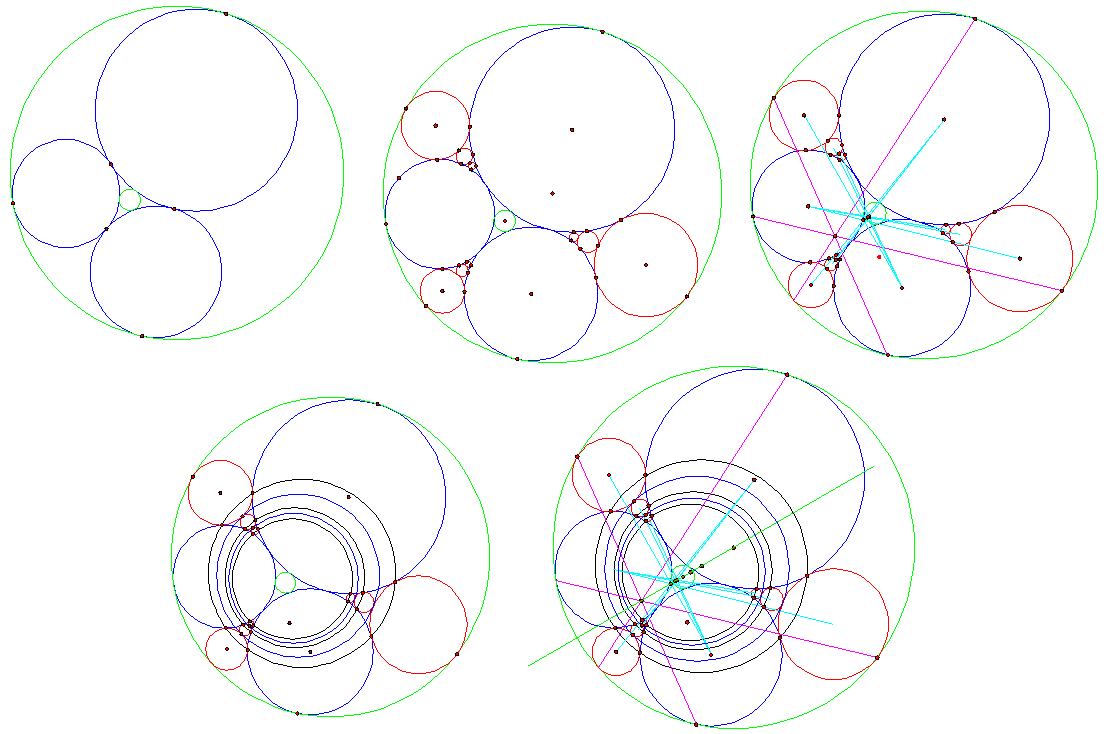
Continuing construct three circles $(M_{1i})$, $(M_{2i})$, $(M_{3i})$ so that:
$(M_{1i})$ is tangent $(B)$ and $(C)$ at $P_{1ib}$, $P_{1ic}$ and $(M_{1i})$ tangent to $(M_{1(i-1)})$ at $P_{1{i-1}}$
$(M_{2i})$ is tangent $(A)$ and $(C)$ at $P_{2ia}$, $P_{2ic}$ and $(M_{2i})$ tangent to $(M_{2(i-1)})$ at $P_{2{i-1}}$
$(M_{3i})$ is tangent $(A)$ and $(B)$ at $P_{3ia}$, $P_{3ic}$ and $(M_{3i})$ tangent to $(M_{3(i-1)})$ at $P_{3{i-1}}$
For $i=2, 3, ...n$
Denote:
- $(H_i)$ is circle through tangent point $(M_{ki})$ and $(M_{k(i-1)})$ for $k=1$, $2$, $3$
Problem 1: Three lines $AM_{1i}$, $BM_{2i}$, $CM_{3i}$, $P_{2ia}$, $P_{2ic}$, $P_{3ia}$, $P_{3ic}$ are concurrent; denote the points of concurrence is $K_i$ for $i=1, 2, ..., n$
Problem 2: Three lines $M_{1k}M_{1i}$, $M_{2k}M_{2i}$, $M_{3k}M_{3i}$ are concurrent, denote the points of concurrence is $H_{ik}$ for $i, k=1, 2, ..., n$
Problem 3: Six point points $P_{1ib}$, $P_{1ic}$, $P_{2ia}$, $P_{2ic}$, $P_{3ia}$, $P_{3ic}$ are concyclic, denote $O_i$ is the circumcenter of this circle.
Problem 4: These points $K_i$, $H_{ik}$, $(O_i)$ lie on a line for $i, k=1, 2, ..., n$
More properties in here