2
$\begingroup$

Let $T$ be a lattice triangle in $\Bbb R^2$ (i.e. the convex hull of three noncolinear points in $\Bbb Z^2$), and assume it has at least one interior lattice point. Is it always possible to find a point $p\in \operatorname{interior}(T)\cap \Bbb Z^2$ satisfying the following condition:

(*) $\operatorname{conv}(p,v)\cap \Bbb Z^2 = \{p,v\}$ for every vertex $v\in T$, where $\operatorname{conv}$ is convex hull.

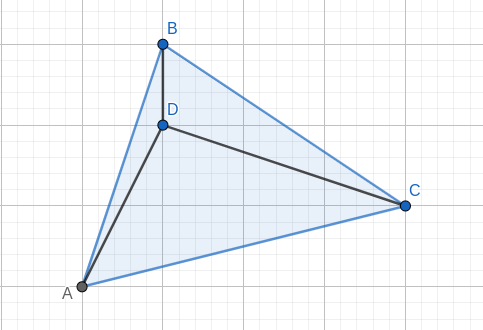
For example: In this picture:

good

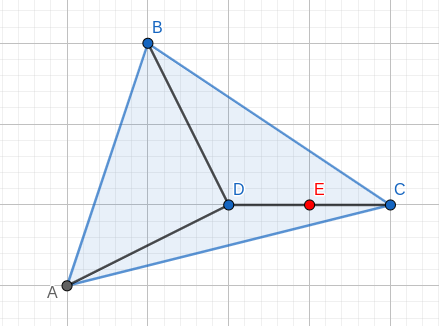
$p=D$ works, whereas in this picture:

enter image description here

$p=D$ does not work because the line segment $DC$ passes through $E$.

Edit: In light of fedja's counterexample (the triangle with vertices $(0,0)$, $(3,2)$, and $(1,3)$, and at their suggestion, I'm modifying the question to ask if (*) holds when $T$ contains "sufficiently many" points in the interior.

Improve this question
$\endgroup$
9
  • $\begingroup$ It looks to me like your definition of $T$ is a bit ambiguous, as it first refers to the entire triangle (with interior), but later (possibly) refers to only the three vertices of it. $\endgroup$
    – Marcus M
    Commented Dec 12, 2018 at 21:15
  • $\begingroup$ @MarcusM I'm not sure what you mean. $\endgroup$
    – Avi Steiner
    Commented Dec 12, 2018 at 21:16
  • $\begingroup$ When you say $p \in T \cap \mathbb{Z}^2$, it looks like you mean for $T$ to include the interior of the triangle; however, your condition for $p$ that you seek is more ambiguous. It says $\text{conv}(p,v) = \{p,v\}$ for all $v \in T$, however in your image you only tested in for $v$ being the extreme points (i.e. the three vertices) of the triangle. Is that the condition that you seek? $\endgroup$
    – Marcus M
    Commented Dec 12, 2018 at 21:18
  • 3
    $\begingroup$ How about (0,0), (3,2),(1,3) for the vertices of $T$? $\endgroup$
    – fedja
    Commented Dec 13, 2018 at 0:09
  • 1
    $\begingroup$ There are general visibility results by Adikhari which may be a start on this. Essentially, if an integer interval is large enough, it contains an integer coprime to a given integer N, and this has implications to your problem. Search for work of Granville in this area too. Gerhard "Will Help Look For Forest" Paseman, 2018.12.12. $\endgroup$
    – Gerhard Paseman
    Commented Dec 13, 2018 at 2:06

0

Reset to default

You must log in to answer this question.