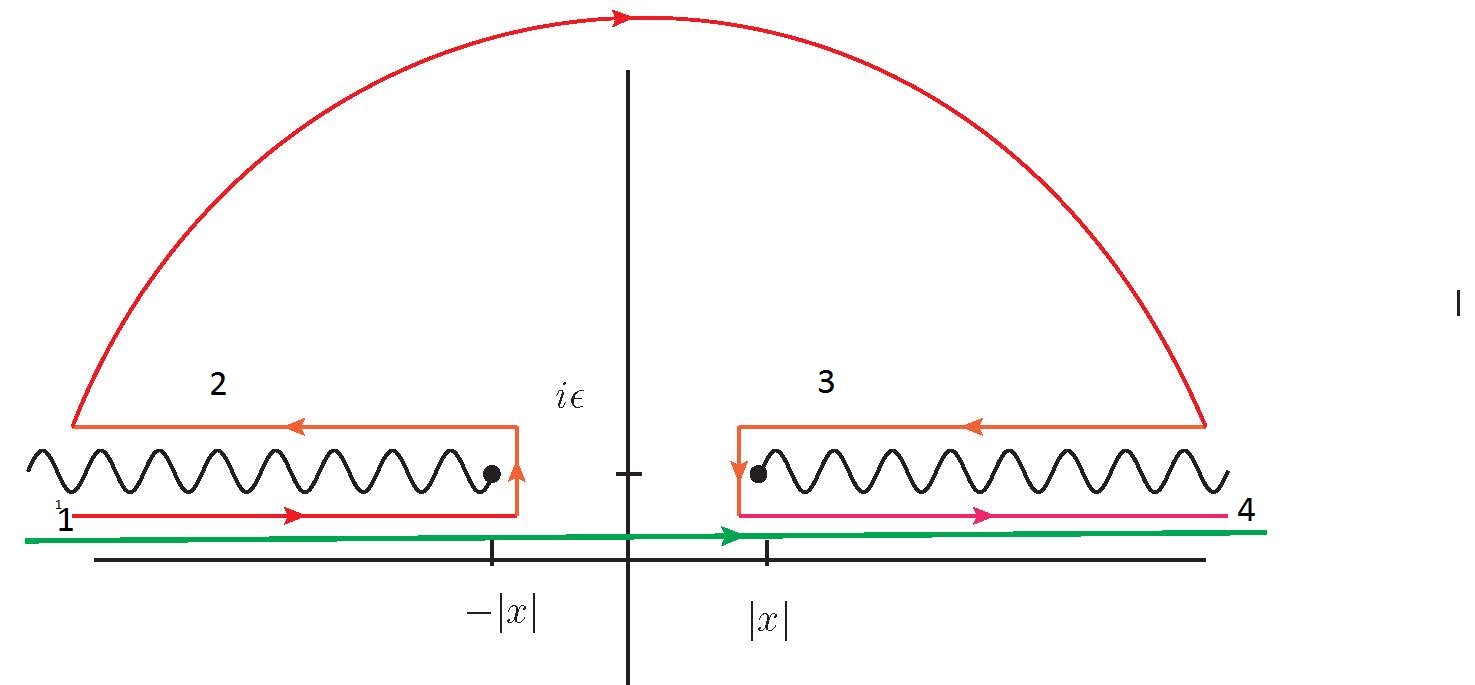
I am sorry to ask such an embarrassingly simple question here. My question is about contour integral of the multivalude function. I want to calculate the Fourier transformation of a muti-valued function $f$ $$G(\omega,\mathbf{k})=\int dt d^{d-1}\mathbf{x} f(t,\mathbf{x}) e^{i\omega t-i\mathbf{k}\cdot\mathbf{x}}$$ with condition that $$\omega>0,\,\,\,\,\,\,\,\omega^2-\mathbf{k}^2>0$$ so that we can deform the contour from the green one to the red one. Function $f$ is given by $$ f(t,\mathbf{x})= \big(\frac{-1}{t^2-\mathbf{x}^2-i\epsilon t}\big)^\Delta $$ For $t<0$, $f$ can be written as $$ f(t,\mathbf{x})= \frac{e^{i\pi \Delta}}{ \big(t^2-x^2\big)^\Delta} $$
For $t>0$, $f$ can be written as $$ f(t,\mathbf{x})= \frac{e^{-i\pi \Delta}}{ \big(t^2-x^2\big)^\Delta} $$
So I need to write down the function on the four legs, $1,2,3,4$ as shown in the figure. My question is how to fied the multi-valued function on each contour? Thanks for any suggestions in advance.