Explaining patterns in modular multiplication graphs

Question

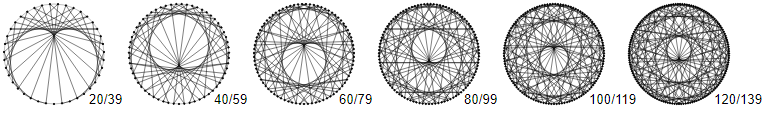
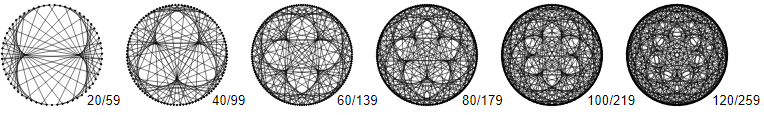
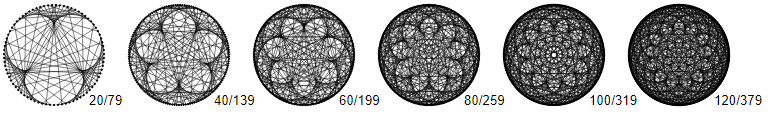
Let the multiplication graph $n/m$ be the graph with $m$ points distributed evenly on a circle and a line between two points $a$, $b$ when $an \equiv b\operatorname{mod} m$.

These graphs often look somehow random but by carefully choosing $n$ and $m$ one finds intricate patterns. Let $n = 20, 40, 60, 80, 100, 120$ and

$m = n + 19$

$m = 2n + 19$

$m = 3n + 19$

Note that it seems essential that $19 = 20 -1$ which guarantees that $m,n$ are coprime.

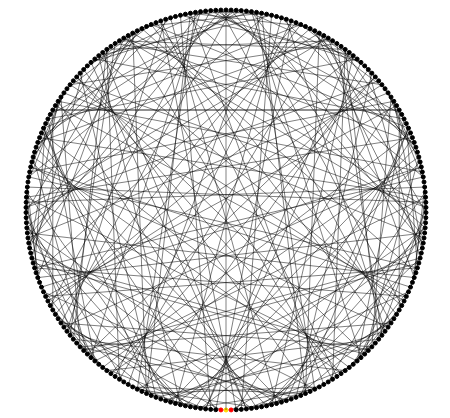
Let $A, B, C$ be arbitrary positive numbers.

Why does the graph $n/m$ have $(C-1)A+1$ petals when $n = AB$ and $m = Cn + B - 1 = B(AC +1) - 1$?

(Note that the number of petals doesn't depend on $B$!)

For example with $A=2$, $B=19$, $C=6$, the graph $38/246$ with $38 = 2\cdot 19$ and $246 = 6\cdot 38 + 18 = 2\cdot 3\cdot 41$ has $11 = 5\cdot 2 + 1$ petals:

Answer 1

The following does not answer the question about the number of cusps, but might be useful.

Consider a continuous version of your construction: draw a line through the points $(\cos t, \sin t)$ and $(\cos nt, \sin nt)$ for every $t$ and look at its envelope. If $F(x,y,t)=0$ is an equation of this line, then the envelope is found from resolving the system of equations $$ F(x,y,t)=0, \quad \frac{\partial F}{\partial t}(x,y,t)=0 $$ with respect to $t$. The result is $$ \gamma(t) = \frac{1}{n+1} \begin{pmatrix} \cos nt + n\cos t\\ \sin nt + n\sin t \end{pmatrix} $$ which parametrizes the trajectory of a point on a circle of radius $\frac{1}{n+1}$ rolling on a circle of radius $\frac{n-1}{n+1}$.

(That this trajectory has the $t$-$nt$ lines as tangents can also be proved geometrically by looking at the instantaneous motion of the point: it rotates about the point of contact between the two circles.)

The curve is called epicycloid, and the construction is attributed to Cremona, see for example this webpage.

It has $n-1$ cusp, which is different from your result. Probably the reason is that you consider a discrete set of lines. By the way, your pictures look similar to those for epicycloids for rational non-integer ratio of the circles radii, see the Wikipedia page.

Also I found a page discussing Mathematica codes for Cremona construction.