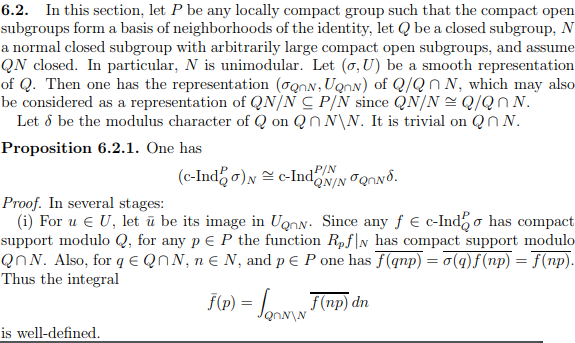
There is a claim in a proof in Casselman's notes on representation theory which I have not been able to verify. I have asked several people and nobody knows why it is true.
The thing I can't figure out is why if $f \in \operatorname{c-Ind}_Q^{P} \sigma$, then the restriction $R_pf$ to $N$ has compact support modulo $Q \cap N$. Clearly we may assume $p = 1$.
Our hypothesis on $f$ is that there exists a compact set $\Omega \subset P$ such that $\{ p \in P : f(p) \neq 0\}$ is contained in the product set $Q. \Omega$. And we want to find a compact set $\Omega_0 \subset N$ such that $\{n \in N : f(n) \neq 0\}$ is contained in $(Q \cap N).\Omega_0$.