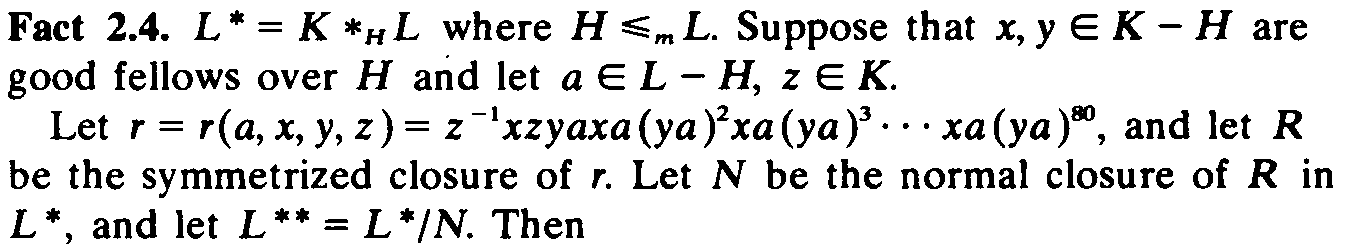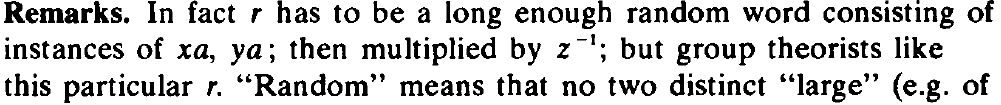In the paper "On a Problem of Kurosh, Jonsson Groups, and Applications", Shelah proved the following
Theorem 2.1. There exists a number $n_0$ such that for every infinite cardinal $\lambda$ with $\lambda^+=2^\lambda$ there exists a group $G$ of cardinality $|G|=\lambda^+$ such that $G=A^{n_0}$ for any subset $A\subset G$ of cardinality $|A|=|G|$.
I would like to deduce the value of $n_0$ from the Shelah's proof (of his Theorem 2.1).
On page 383 Shelah writes that $n_0$ is the length of the word $r$ in Fact 2.4:
The word $r$ in Fact 2.4 is here:
Just after Fact 2.4 in Remarks Shelah writes:
- I have a doubt that this word $r$ should start with $z^{-1}xz$ and suggest that it should be $z^{-1}xa$.
Note that in Remarks, Shelah writes that $r$ consists of instances of $xa$ and $ya$, then multiplied by $z^{-1}$. So, it is natural to suggest that only one letter $z$ should occur at the very end (or beginning, depending from which side start counting) of the word $r$.
Also discussing the Small Cancellation Theory in $\S 1$, Shelah cites a result of Schupp concerning the symmetrized closure of the word $$ax(ay)ax(ay)^2ax(ay)^3\cdots ax(ay)^{80}:$$
The length of the word $r$ in Fact 2.4 equals $1+2\cdot 80+2\frac{80\cdot 81}2=1+80\cdot 83=6641$.
It seems that the Shelah's construction for any subset $A\subset G$ of cardinality $|A|=|G|=\lambda^+$ and any element $z\in G$ yields elements $x,a,b\in A$ such that $r(a,x,y,z)=1$, so if my suggestion 1 is true, then $z$ belongs to $A^{l(r)-1}=A^{6440}$, where $l(r)=6641$ is the length of the word $r$.
Therefore, if my suggestions (1)-(3) are correct, then Theorem 2.1 has the following more precise form:
Theorem. For every infinite cardinal $\lambda$ with $\lambda^+=2^\lambda$ there exists a group $G$ of cardinality $|G|=\lambda^+$ such that $G=A^{6440}$ for any subset $A\subset G$ of cardinality $|A|=|G|$.
So, my question:
Is $n_0$ in Shelah's Theorem 2.1 equal to 6640 or 6641? Or maybe to some other number?