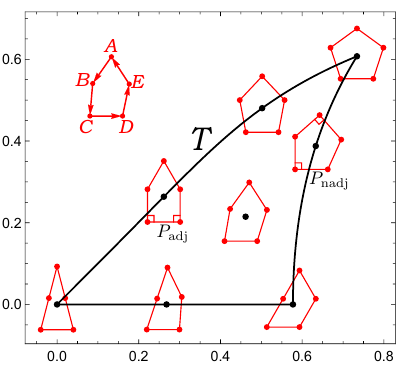
Here is a triangular region $T$ whose two curved edges are complicated analytic curves that I know only numerically, but can compute to any desired precision:
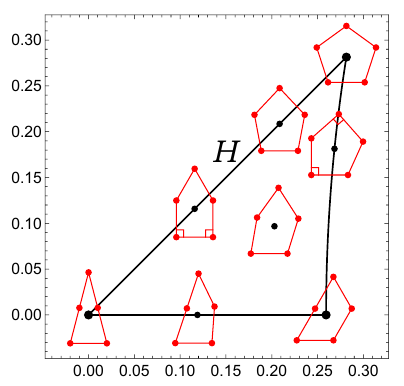
And here is a simpler region $H$ whose one curved side is $\pi/20=9^\circ$ of a circular arc:
Both regions have vertex angles of $\pi/5$, $\pi/4$, and $\pi/2$; so there is a unique conformal map $f\colon T\to H$ that takes each vertex of $T$ to the corresponding vertex of $H$. And the map $f$ is holomorphic, not only on the interior of $T$, but also on its boundary.
I have managed to approximate $f$ numerically by a degree-13 polynomial $p(z)$ of a complex variable $z$ whose coefficients are real numbers. So $p$ maps the real axis precisely to itself. And $p$ maps the two curved boundaries of $T$ to analytic curves that are never farther than 0.00003 from the corresponding boundary of $H$. I have thus approximated $f$ to roughly four digits.
But I would like to approximate $f$ much more precisely --- to at least ten digits, maybe twenty. Can anyone suggest a technique by which I could achieve this? Replacing $p$ by a polynomial of higher degree isn't an attractive strategy, since the precision achieved is likely to grow only slowly with increasing degree, while solving for the coefficients of a high-degree polynomial is numerically challenging. Indeed, polynomials may not be a good library of functions to use as approximations to $f$, since polynomials are entire, while $f$ probably has singularities at finite points outside of $T$.
There are Fortran libraries for numerically approximating conformal maps. But they typically use a fixed precision, either single or double; and they deal with conformal maps either to or from the upper half-plane. I am hoping to implement something in Mathematica, so that I can specify a high working precision. And approximating $f$ directly, rather than detouring through the upper half-plane, seems like it should be easier, since $f$ is holomorphic also on the boundary of $T$.
I will append a few remarks about how this problem arose, but I won't give all the details, since the full story is rather complicated.
Suppose that we identify labeled equilateral pentagons $ABCDE$ in the Euclidean plane that differ by any combination of translation, magnification, and rotation --- but that we don't identify a pentagon with its reflection. The resulting moduli space $M$ is known, topologically, to be a compact, orientable, smooth 2-manifold of genus 4. The region $T$ is the triangular subset of $M$ in which the pentagons are convex, are traversed counterclockwise, and have $A$ and $B$ as their narrowest and widest vertices. The region $T$ turns out to be $1/240^\text{th}$ of $M$. (Along the bottom boundary, the widest vertex $B$ straightens out; along the upper-left boundary, the vertices $B$ and $E$ are tied for widest; and along the right-hand curved boundary, the vertices $A$ and $C$ are tied for narrowest.)
A metric on $M$ needs to assign some length to each tangent vector, where a tangent vector at a pentagon $P$ is a recipe for altering the shape of $P$ as function of time. Extend all pairs of the edges of $P$ until they intersect. We can then define the length of that tangent vector as the Euclidean norm of the vector in $\mathbb{R}^{10}$ whose coordinates are the rates of change of the ten resulting angles, say in radians per time step. Call that the ten-angles metric. (We use all ten intersections, rather than just the five vertex angles, to achieve greater symmetry.)
Equipping the moduli space $M$ with this ten-angles metric gives us a Riemannian manifold with an isometry group of order 240, a manifold that is partitioned into 240 regions, each isometric to $T$. The Gaussian curvature of this manifold is everywhere negative, but is not constant.
Pulling the metric of the hyperbolic plane back onto $M$ through the conformal map $f$ will allow us to view $M$, with the conformal structure of the ten-angles metric, as the quotient of the hyperbolic plane by a Fuchsian group, an index-240 subgroup of the (2,4,5)-triangle group. (Indeed, $M$ with this conformal structure is conformally equivaent to the Bring sextic, the most symmetric compact Riemann surface of genus 4.)
The opening illustration of $T$ is drawn using a coordinate system that is isothermal for the ten-angles metric. I compute my isothermal coordinate system using Gauss's technique for reducing the Beltrami partial differential equation, in the real-analytic case, into an ordinary differential equation over the complex numbers. That ODE has the form $q'(t)=F(t,q(t))$ where the function $F$ is algebraic, but is so complicated that solving the ODE in closed form seems quite unlikely. Instead, I have Mathematica solve it numerically.
Thus, while I don't know the curved boundaries of $T$ in closed form, I could easily compute thousands of points along each of them, each point to any desired precision (within reason). Note also that I don't need to refine my approximation to $f$ efficiently; I'll be happy to spend weeks of CPU cycles, is that's what it takes to get $f$ to twenty digits.
The sample pentagons in the drawing of $H$ are positioned using $p$, my degree-13 polynomial approximation to $f$. I would like to approximate $f$ more accurately since I would like to know, for example, just where the two pentagons $P_\text{adj}$ and $P_\text{nadj}$ end up along the boundary of $H$, those being the equilateral pentagons with two right angles, either adjacent or nonadjacent.