As far as I understood, for the Galton-Watson tree process, the offspring are of one type. I am thinking of the case where we have offspring of different types. I have illustrated this in the example below:
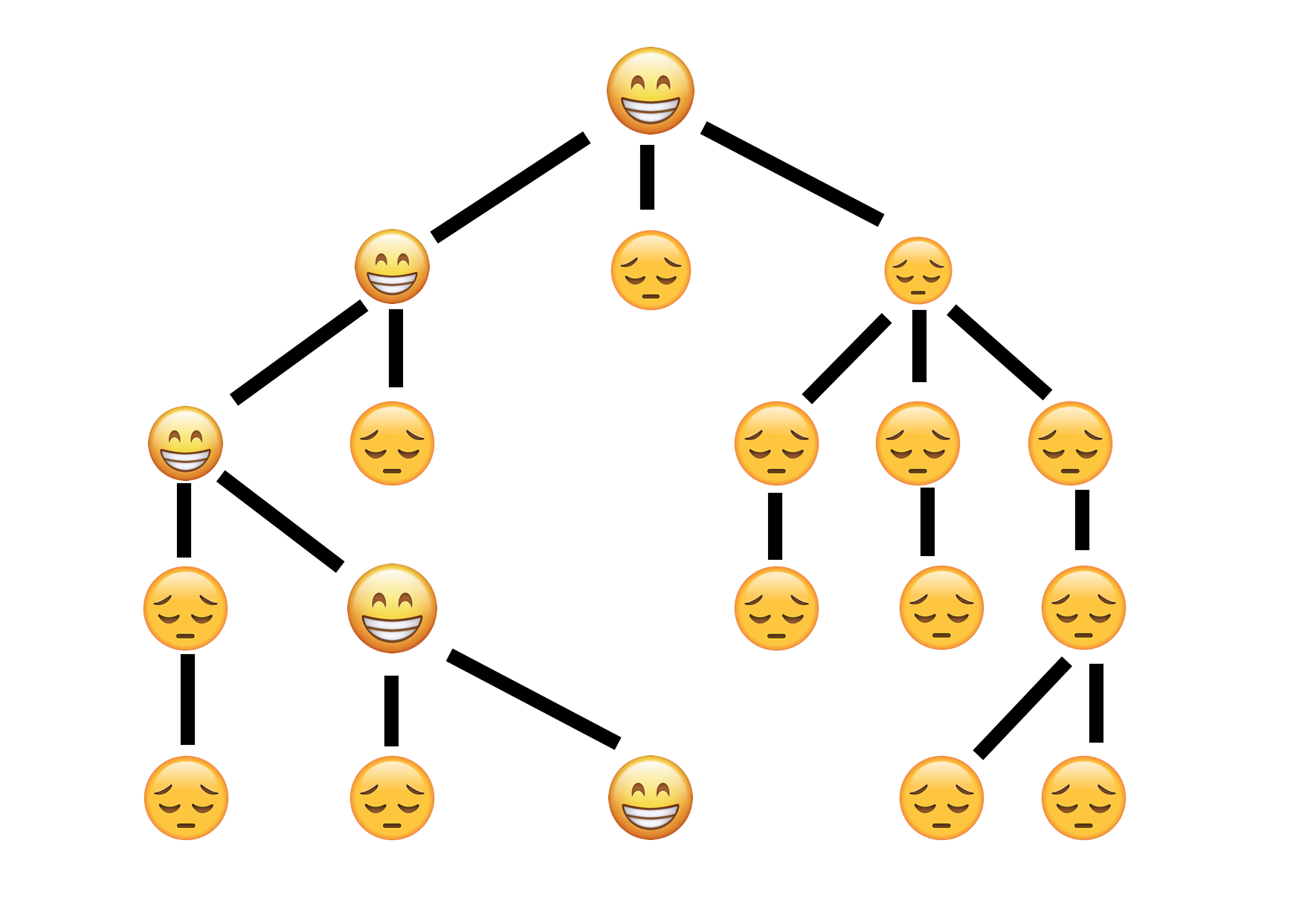
It can be seen that we have both sad face and happy face, rather than just a single type emoji. I am interested to find out the expected height of such trees for a given face (happy or sad). Let us assume that the reproduction goes with Poisson distribution.
I know that if we had only one type of children we could write the expectation via a generating function. First the generating function would be: $$f(s)=\sum_{k=0}^{\infty}P(Z_n=k)s^k$$ where $k$ is the number of children and $P(Z_n=k)$ the probability and $s$ is a dummy variable in that case we can define: $$m=E[Z_n]=f'(s)|_{s=1}=\sum_{k=0}^{\infty}P(Z_n=k)k$$ I am not sure but I think for the two type (Happy and Sad) we should have something like: $$E[Z_0]=\sum_{n=0}^\infty P[n=k](1/2)\max _{i=1...k}(1+E[X_i])�$$ where $\max _{i=1...k}(1+E[X_i])$ is the maximum expectation for each child and factor of 1/2 appears as we assume 50-50 chance of being happy or sad. Are there such situations in literature?
Update: Since becoming familiar with multi type branching processes, I formatted my problem as follow:
I am considering a branching process with two types. I shall designate them with 1 and 2. The branching process follows Poisson distribution. So to that end I may write the probability generating functions as: $$f^1(\mathbf s)=\sum^{\infty}_{k_1=0}p_{k_1}s^{k_1}=e^{\lambda(s_1-1)}$$ $$f^2(\mathbf s)=\sum^{\infty}_{k_2=0}p_{k_2}s^{k_2}=e^{\lambda(s_2-1)}$$ I have borrowed this notation from the fact that, $$f^i({\mathbf s})=\sum_{\mathbf k}p_i(\mathbf k)s^{k_1}_{1}s^{k_2}_{2}\cdots s^{k_m}_{1}$$ Now I wonder how shall I proceed to calculate the extinction probability from here?
