0
$\begingroup$

[This question is related to another question concerning normal numbers I asked at Math SE.]

Has it ever been found worth to ask the question if the Champernowne constants $C_m$, especially $C_2$ might be related to other (known or nameable, algebraic or transcendental, probably normal) numbers like $\sqrt{2}$, $\pi$ or $e$? Is there any chance, or are there good arguments that $C_2$ probably won't be related to e.g. $\pi$, i.e. there's no closed formula $\phi(\cdot)$ saying $C_2 = \phi(\pi)$.

An argument might go like this:

The value of $C_2$ depends on the arbitrary base 2 while the value of $\pi$ doesn't.

But maybe 2 isn't so arbitrary? (To say the very least: 2 is a prime number. And there's a deep connection between prime numbers and $\pi$.)

As infinite series they are defined by

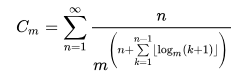
enter image description here
(http://mathworld.wolfram.com/ChampernowneConstant.html)

and take values like

$C_2 = 0.862240125868054571557790283249394578565764742768299094516\dots$

$C_3 = 0.598958167538433992500172217929436590978208768676105936754\dots$

We obviously don't see at a glance that $C_2 = \frac{\pi}{3} = 1.047\dots$ or $C_2 = \frac{\pi}{4} = 0.785\dots$ or $C_2 = \frac{\pi^3}{2^5} = 0.968\dots$. ( Endless combinations abound.)

But who assures us - just for example - that no enumerable sequence $(\alpha_k)$ with $\alpha_k \in \mathbb{Q}$ exists with $C_2 = \sum \alpha_k \pi^k$?

Note that many infinite series resulting in a closed expression over $\pi$ – first of all Leibniz' formula for $\pi$ – came somehow as a surprise. Can this be turned around?

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ It's trivial to construct lots of infinite sequences $\alpha_k\in\mathbb Q$ such that $C_2$ (or any real number you like) equals $\sum \alpha_k \pi^k$ (or also, if that's what you meant, $\sum \alpha_k \pi^{-k}$)—just by the density of $\mathbb Q$ in $\mathbb R$. Do you mean something else by "enumerable"? $\endgroup$
    – Greg Martin
    Commented Sep 14, 2018 at 18:34

1 Answer 1

Reset to default
3
$\begingroup$

One argument against $\pi$ being related to $C_2$: while $C_2$ is normal, its normality is really terrible in the sense that the convergence of its digit-sequence frequencies to uniform is extremely slow. Almost all real numbers, and (presumably) all algebraic expressions in $\pi$, should be not only normal but should approach the limiting frequencies about as fast as sequences of coin tosses would.

You can find out more about this concept of "strong normality" in this paper of Belshaw and Borwein.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Thanks a lot for the hint to the Belshaw/Borwein paper. Great reading! $\endgroup$
    – Hans-Peter Stricker
    Commented Sep 18, 2018 at 9:12
  • $\begingroup$ What can be learned from the fact, that it's not known whether the decimal expansion of $\sqrt{2}$ contains infinitely many zeros, while it is obvious, that its binary expansion must contain infinitely many zeros (and ones). The first base where it's unclear would be 3. $\endgroup$
    – Hans-Peter Stricker
    Commented Sep 18, 2018 at 9:15

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .