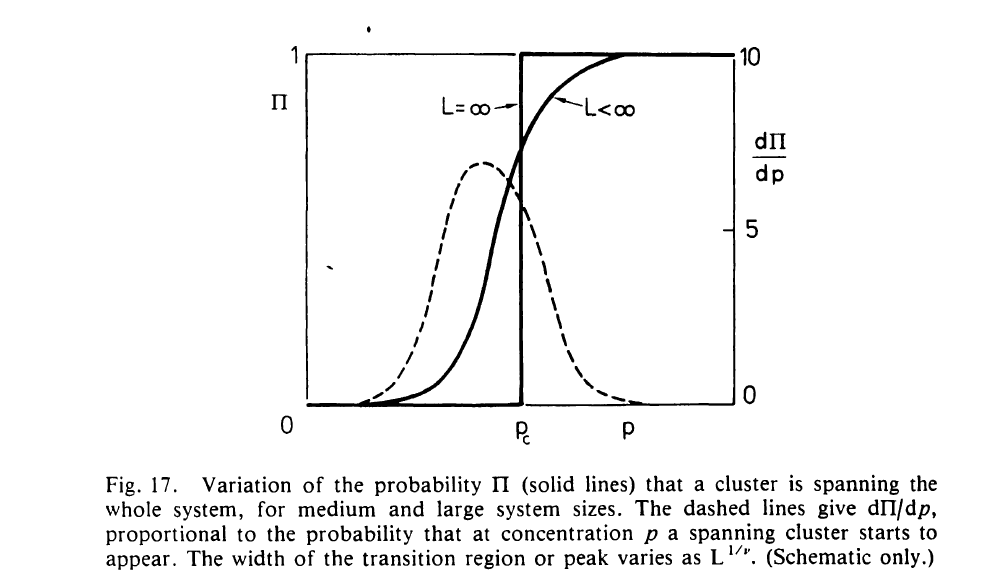
As mentioned in the textbook "Introduction to Percolation Theory" (Chapter 4) by Stauffer et al., the variation of spanning cluster percolation probability $\Pi$ in a finite $L < \infty$ square lattice has the following nature:
For finite sized lattices, there is no sharp value of percolation threshold but rather a range of values of $p$ over which the $\Pi$ transitions from $0$ to $1$. Now, my physics professor told me to write a program to estimate "average" site percolation threshold for finite square lattices with $L=125, 250, 500$ and $1000$ over $100$ iterations (i.e. over $100$ randomly generated binary matrices) for each such value of $L$. She said that the procedure I should follow is:
Find that value of $p$ at which in at least $50$ out of the $100$ random matrices, a spanning cluster exists
Label those values of $p$ as $p_{125}, \ p_{250}, \ p_{500}$ and $p_{1000}$ respectively.
Prepare a table like this:
$1000/L \ \ \ \ p_{\text{average}}$
$\ \ \ \ 8 \ \ \ \ \ \ \ \ \ p_{125}$
$\ \ \ \ 4 \ \ \ \ \ \ \ \ \ p_{250}$
$\ \ \ \ 2 \ \ \ \ \ \ \ \ \ p_{500}$
$\ \ \ \ 1 \ \ \ \ \ \ \ \ \ p_{1000}$
and apply linear regression on the above table to estimate the value of $p_{\text{average}}$ when $1000/L = 0$ (meaning $L = \infty$). That should give the approximate value of percolation threshold of an infinite square lattice.
My Confusions:
I'm not sure how taking $100$ iterations (i.e. over $100$ randomly generated matrices) is sufficient to estimate the "average" site percolation threshold for finite lattices. Or for that matter, even a $1000$ or a $10,000$ iterations doesn't seem mathematically justifiable to me. A binary matrix of size $L=1000$ could have millions of different configurations. This, in turn, I feel would give rise to very large inaccuracies in the estimation of the infinite lattice percolation threshold.
The bigger problem, I feel, is that my professor told me to define "average" percolation threshold for finite lattices as that probability at which $\Pi = 50 %$. But my objection is: while I might be able to estimate the
$p_{\text{100}}$ at which out of $100$ random matrices, $50$ have spanning clusters, the statistics might become completely different when I take more random matrices, say $1000$ or $10,000$ instead of $100$. In that case, at $p_{\text{100}}, $ maybe out of $1000$ random matrices only $300$ will turn out to contain spanning clusters!
Question:
Is there any possible way to mathematically/statistically justify the "random sampling" over only $100$ configurations to estimate site percolation thresholds? I'm particularly worried about the rigour of this procedure because my undergrad research supervisor intends to send this paper (which also contains some other results other than this) for publication in the future, but I really wouldn't be happy with mathematical crackpottery in my paper. Also, I'd be glad to hear if you can suggest some improvements to the method my physics professor asked me to follow. Thank you.