Compactification of 6d (2, 0) SCFT on 4-manifolds

Question

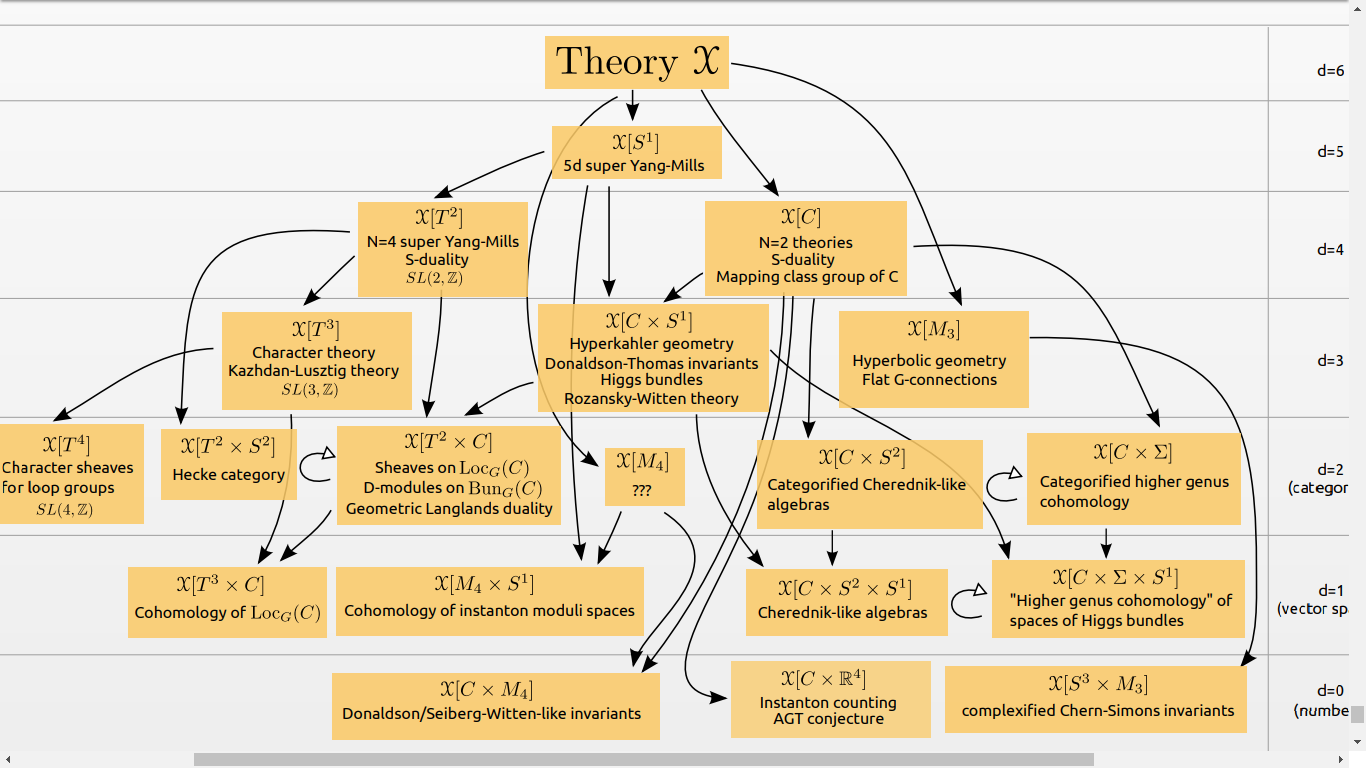
This question is about the 6d (2, 0) superconformal field theory (also called 'theory X' by some people). This SCFT, which can be considered as a relative quantum field theory (see here for a definition), exhibits a great deal of mathematical structure which is cogently expressed in the diagram below (taken from slides for D. Ben-Zvi's talk). In particular, its compactification on 2-torus is $N=4$ Yang--Mills theory. Note that the S-duality of the compactification of a topological twist of $N=4$ SYM (the so-called Kapustin--Witten TQFT) on a closed Riemann surface encodes geometric Langlands duality.

My question is: has there been any recent progress on understanding the compactification of theory X on general 4-manifolds (marked as '???' in the diagram)?

Answer 1

You could have a look at https://arxiv.org/abs/1806.02470 and references therein.

EDIT (taking into account the comment): compactification of the $\mathcal{N}=(2,0)$ 6d superconformal field theory on a 4-manifold (with an appropriate topological twist along the 4-manifold), produces a 2d (6=4+2) $\mathcal{N}=(2,0)$ superconformal field theory. Taking its chiral part, one should get a chiral algebra of operators, or vertex operator algebra (VOA), which is a well-defined mathematical object. So a weak version of the problem is to produce a VOA from a 4-manifold. This expected VOA is known in some examples and some strategy, used in the linked paper, is to construct more general examples by trying to identify the effect on the VOA of basic topological operations on the 4-manifold.

The character of the VOA (or the elliptic genus of the 2d superconformal field theory) is expected to be related to Vafa-Witten invariants of the 4-manifold. In general, Vafa-Witten invariants should be some counts of solutions of some gauge theoretic PDE (similar to Donaldson invariants). The required analysis dealing with the non-compactness of the relevant moduli spaces does not seem to have been done. Recent progress includes a definition of Vafa-Witten invariants via algebro-geometric techniques for projective complex surfaces, by Tanaka and Thomas (see https://arxiv.org/abs/1702.08487 ).