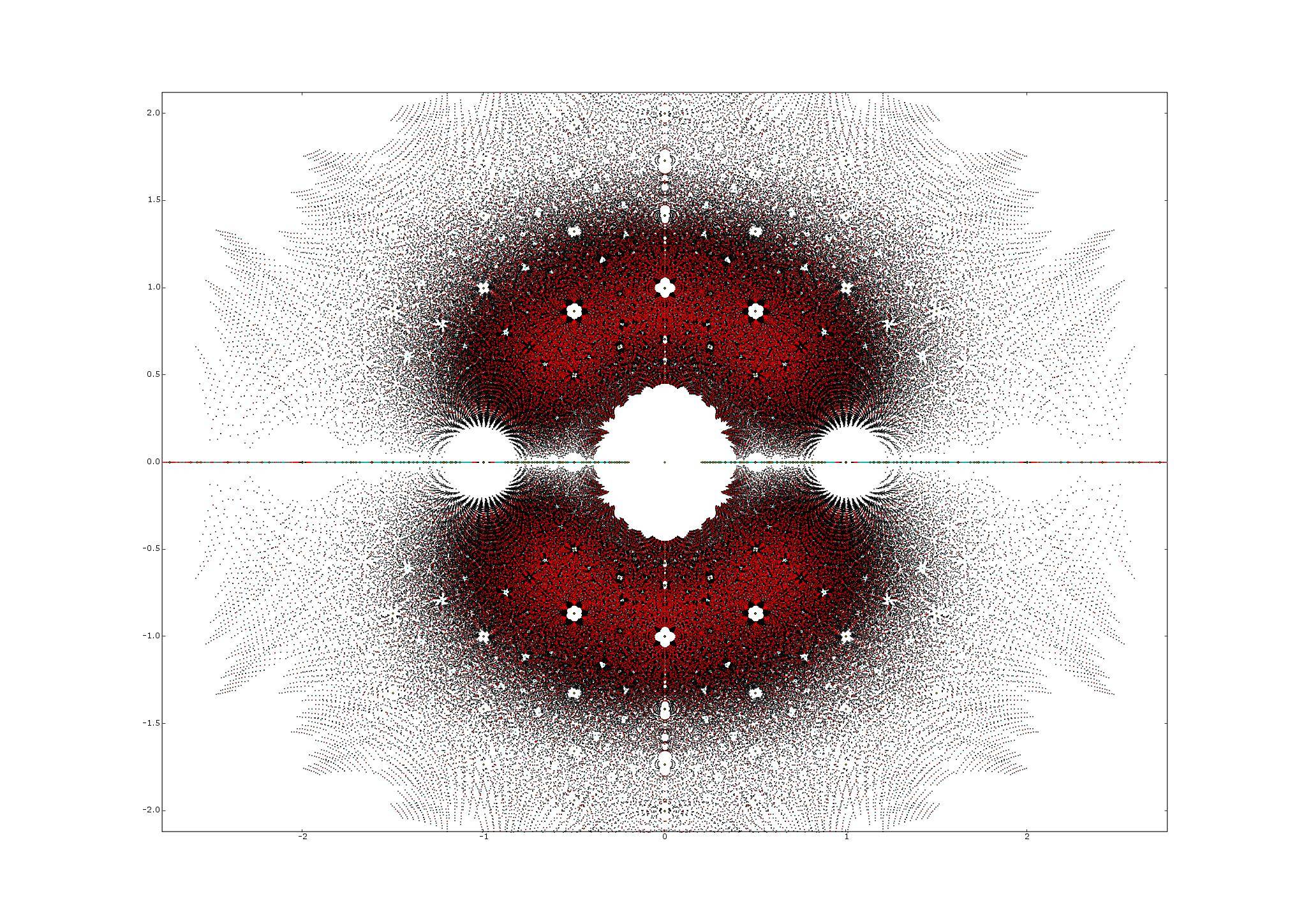
Below are shown two displays of all the roots of polynomials $$c_n x^n + c_{n-1} x^{n-1} + \cdots + c_1 x + c_0 \;=\; 0$$ with each coefficient $c_i$ an integer $|c_i| \le M$ (including $c_i=0$). No doubt this is all well-known, but I would be interested to learn what results explain the patterns in the distributions, especially the holes, both surrounding the real axis—in both shape and location—and the off-axis holes, perhaps more evident in the degree-$3$ plot than in the degree-$5$ plot.
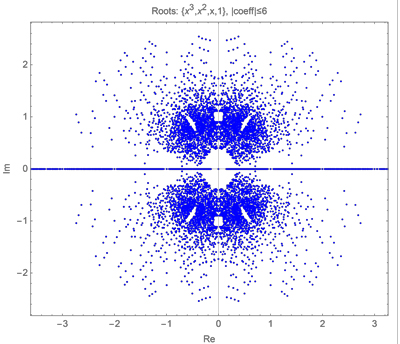
Roots of polynomials of degree $\le 3$ and integer coefficients $|c_i| \le 6$.

Roots of polynomials of degree $\le 5$ and integer coefficients $|c_i| \le 5$.
Addendum. User j.c. cited the article by John Baez on Dan Christensen's impressively detailed images, one of which I include below: