3
$\begingroup$

The lonely runner conjecture states that for $M=\{m_1,...,m_n\}$ a set of distinct positive integers the quantity $$ \kappa(M):=\sup_{t \in \mathbb{R}} \min_i ||tm_i|| $$ satisfies $\kappa(M) \geq \frac{1}{n+1}$, where $||x||$ denotes the distance of $x$ from the nearest integer.

It is stated without proof in Remark 1 of this paper that $\kappa$ is a rational number whose denominator divides $m_i+m_j$ for some $i,j$. I have seen this property of the denominator mentioned in several other places, without a satisfying reference.

Is a proof of this property of the denominator published anywhere? Is there some simple proof I am overlooking?

Improve this question
$\endgroup$
3
  • $\begingroup$ There is a point $t$ such that attained the $\sup$ and for some $m_i$ and $m_j$ we have $\|t m_i\| = \|t m_j \| = \kappa$ $\endgroup$
    – Mahdi - Free Palestine
    Commented May 25, 2018 at 20:42
  • $\begingroup$ That hopefully gives the solution. $\endgroup$
    – Mahdi - Free Palestine
    Commented May 25, 2018 at 21:16
  • $\begingroup$ @Mahdi Indeed. The only extra information needed is that $tm_i$ and $tm_j$ are on the opposite sides from the corresponding nearest integers. $\endgroup$
    – fedja
    Commented May 25, 2018 at 21:50

1 Answer 1

Reset to default
2
$\begingroup$

$\newcommand{mod}{\mathrm{mod} \ }$ Define $f_i(t) := \| t m_i \|$. We have

$$ f_i(t) = \begin{cases} m_i t - k, & \text{if } t\in[\frac{2k}{2m_i},\frac{2k+1}{2m_i})\\ -m_i t +(k+1), & \text{if } t \in [\frac{2k+1}{2m_i},\frac{2k+2}{2m_i}) \end{cases} \tag{1} $$ $f_i$ is a continuous piecewise linear function, which is $0$ at $\frac{2k}{2m_i}$ and is $\frac 12$ at $\frac{2k+1}{2m_i}$.

Now, consider the function $$f(t) := \min_i f_i(t)$$

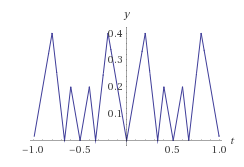
$\hskip2in$ a typical graph of $f$

We want to find $\sup_{t >0} f(t)$. Since $f$ is periodic, it is enough to consider $\sup_{0<t<1} f(t)$. Moreover, for sufficiently small $\epsilon >0$, it is enough to consider $\sup_{\epsilon \leq t \leq1-\epsilon} f(t)$. Since $f$ is a continuous function attains its supremum on $\epsilon \leq t \leq1-\epsilon$ at some point, like $t_0$. There is two function, like $f_i$ and $f_j$, such that $$f_i(t_0)=f_j(t_0)=f(t_0)$$ Note that the rules of $f_i$ and $f_j$ at $t_0$ are different in (1), i.e. $\lfloor 2 m_it_0 \rfloor \not\equiv \lfloor 2 m_j t_0 \rfloor (\mod 2)$. (otherwise $f(t_0)$ can not be supremum). For example, let $f_i(t_0)= m_it_0-k_i$ and $f_j(t_0)=-m_j t_0 +(k_j+1)$, for some $k_i,k_j \geq 0$. Now the equality $f_i(t_0)=f_j(t_0)$ gives $$t_0 = \frac{k_i+k_j + 1}{m_i+m_j},\quad \kappa=f(t_0)= \frac{m_ik_j-k_im_j+m_i}{m_i+m_j}$$ Now, the result at hand.

This may be a reference to your question. Just click on the 'cite' link below!

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .