Tangled random triangles: One giant component?

Question

Suppose you have $n$ triangles whose corners are random points on a sphere $S$ in $\mathbb{R}^3$. Viewing the triangles as built from rigid bars as edges, two triangles are linked if they cannot be separated without two edges passing through one another. A triangle that is not topologically linked with any other is loose. In the example below of $n=15$ triangles, $11$ are linked to at least one other triangle, and $4$ are loose.

---

         
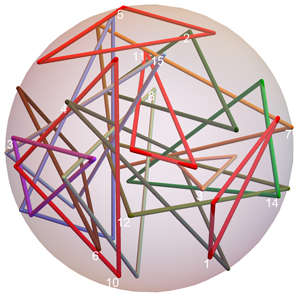
          ^{$n=15$. Magenta triangles $\{1,5,10,11\}$ are loose.}

---

It is easy to surmise that the proportion of linked triangles approaches $1$ as $n \to \infty$:

---

         
          ^{Fraction of triangles linked to at least one other triangle.}

---

But I wonder about the largest linked component, the collection of all triangles linked into one "giant" component, in the sense that if you picked up one triangle all the others would follow. I wonder that when $n \to \infty$, what is the probability that this linked component includes all the triangles.

Q. As $n \to \infty$, what is the probability that every triangle is linked into one giant component?

My sense is that this probability is zero: Even though the probability that each triangle is linked to another approaches $1$, the probability that all triangles are linked to one another approaches $0$. This would contrast with an earlier related question, Random rings linked into one component?, whose answer was the opposite: The rings form one component as $n \to \infty$.

Answer 1

Here is @fedja's clever example: The magenta triangle is topologically loose but metrically "stuck":

---

         
          ^{fedja: "(link two large ... triangles [blue & green] near vertices
          and surround the linkage by a small ... triangle [magenta])"}

---

Answer 2

Here is an idea which suggests why the probability is near zero as the number of triangles gets large.

Among the many random triangles, there is one which has the smallest area as measured when embedded in the plane. Let us call this triangle T, and consider how likely that another triangle links with it.

Pick a random point p on the sphere. From the vantage point of p, look at T. For p belonging to much of the sphere, T looks small and tilted, so that a chord from p passing through the interior of (the plane embedded version of ) T will strike the sphere on the other side in a small area. (Bisecting the sphere by the plane of T, such a chord has to have an endpoint in either piece. If the triangle is contained in a small cap, the strike area for much of the sphere is within this small cap.)

However, not only does this point opposite p (call it q) reside in this small cap, it has to "see" a point away from the triangle: if it just connects to another point by a chord passing through the triangle interior again, there is no link. In particular, the chord from q that does not head to p has to live in a minimal cap that contains q and one of the small edges of T. (I am ignoring the case that q is near the boundary of the small cap.)

To measure how unlikely this is, consider the following. For a given T and many points q in "the small cap" of T, compute the area of the three small regions given by that part of a new cap determined by a plane containing q and an edge of T and the region of interest being on that part of the cap "on the other side of the edge from q". If T has small area (and q lies "sufficiently inside" T), the area of these three regions will be even smaller than T. You now need the probability that a point q lands inside of (the small cap part of ) T, with a point r that lands in the special region that would give a link, which intuitively is of the same order as the area of T, multiplied by the probability that p permits a linking triangle with T (which is much larger than the previous probabilities).

This might result in a small but significantly far from zero probability of occurring. But now, take the number of small triangles to be large, and it becomes easy to believe that one of these small triangles is not linked to any other.

Gerhard "See The Lack Of Link?" Paseman, 2018.04.27.