Let $(W,S)$ be a Coxeter group and $*:S \to S$ be an automorphism of the Dynkin diagram of $W$ so that $*^2$ is the identity. This induces a bijection $*:W \to W$ mapping $w = s_1 \dots s_n$ to $w^* = s_1^* \dots s_n^*$. We say $y \in W$ is a twisted involution if $y^{-1} = y^*$. Viewing $S_n$ as a Coxeter group generated by simple transpositions, the only choices of $*$ are the identity and the map that sends $s_i = (i,i+1)$ to $s_i^* = s_{n-i}$. In this setting, we see $y$ is a twisted involution if and only if $y = w_0 y w_0$, i.e., if $y w_0$ is an involution (here $w_0$ is the reverse permutation $n \ n{-1} \dots 1$).
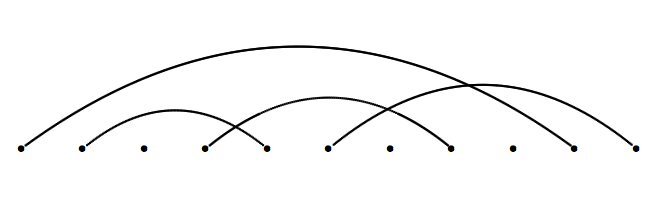
Involutions in the symmetric group $S_n$ have a clear combinatorial description as matchings on the complete $K_n$ (each 2-cycle corresponds to an edge). This is often depicted graphically:
corresponds to the involution $(1,10)(2,5)(4,8)(6,11)$.
Question: Is there a preferred combinatorial model in the same vein for twisted involutions in the symmetric group?