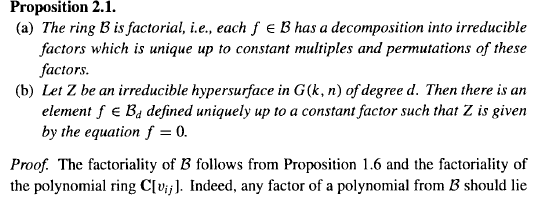
I'm studying this proof in the book cited in the title.
The author say that any multiplicative function $\chi\colon\mathsf{GL}(k)\longrightarrow\mathbb{C}^\times$ is a power of the determinant. But what if I take $\chi(g)=\overline{\det g}$? Is this a mistake? If not and I'm missing something where can I find a proof of this fact?