3
$\begingroup$

A certain family of graphs crossed my way while performing some quantum mechanics calculations, and I am very curious whether they have been studied in mathematics before in a different context.

Also I would be interested in properties of these graphs, other that they are planar multi-graphs.

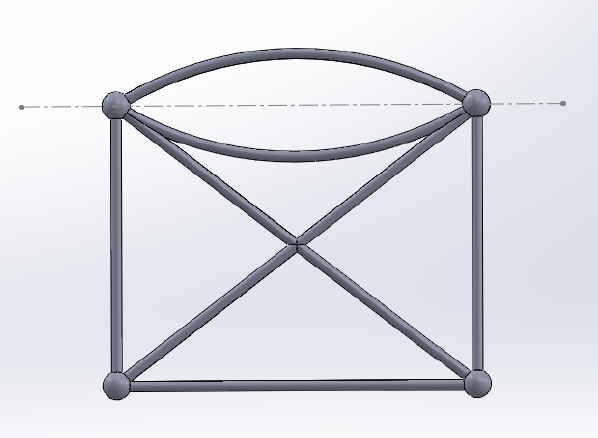
Here are the examples. Let's start with the simplest example. It is a complete $K_4$ graph with one additional edge, making one edge a double-edge (let's call it Wing$_1$ graph, for reasons that become clear soon):

Wing-1

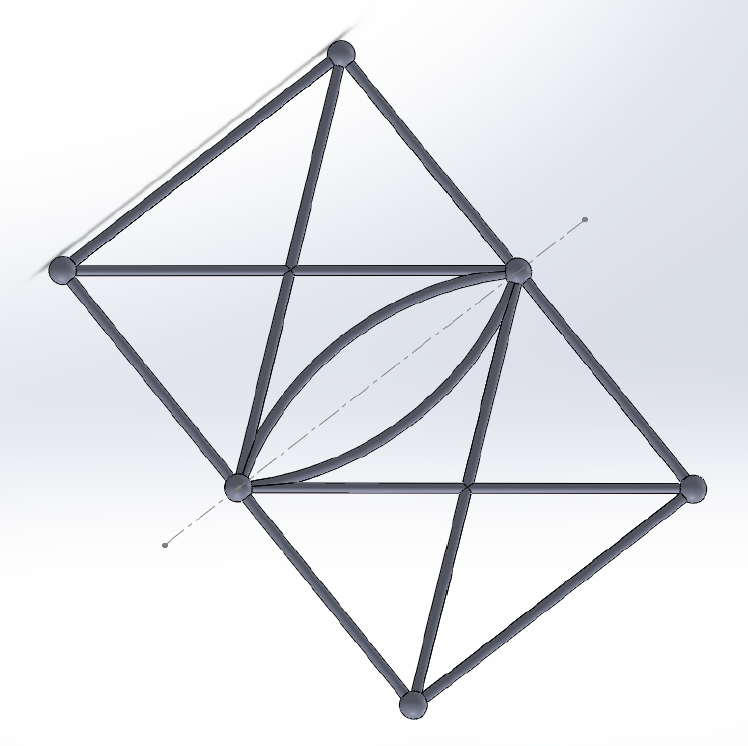
Now here the second member of the family. These are two $K_4$ graphs which are connected at two vertices (let's call it Wing$_2$ graph):

Wing-2

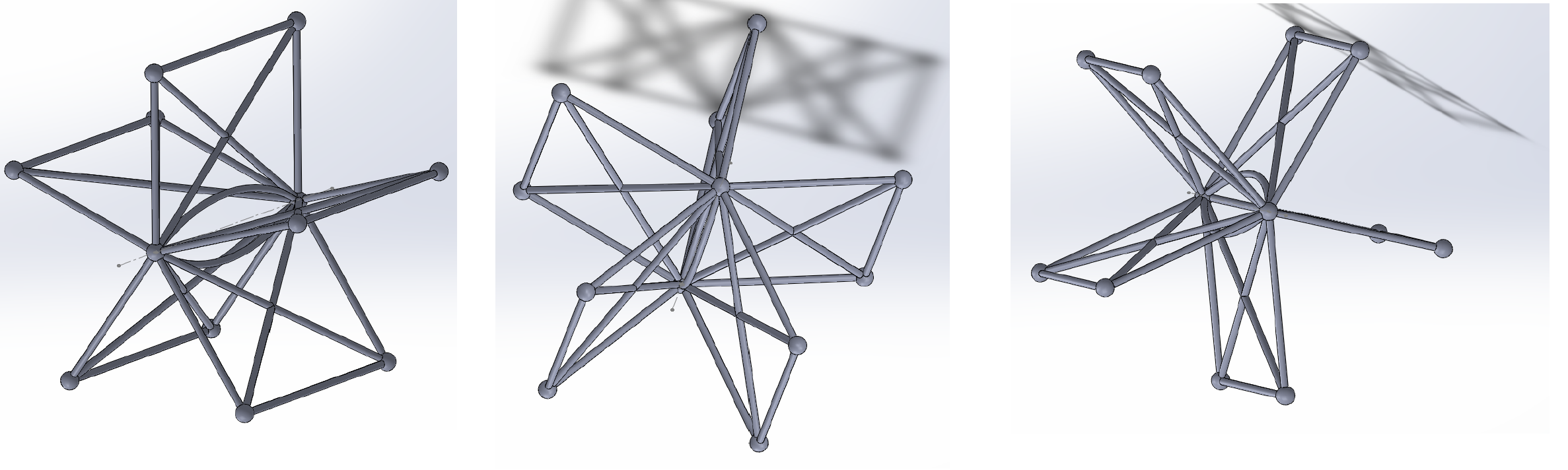
And now we add more and more $K_4$ graphs to the graph, but without increasing the number of edges between the central two vertices. Here is an example of the Wing$_5$ graph (from three different perspectives).

Wing-5

I am very curious whether this is family has been studied before, whether it belongs to a larger class of graphs with certain properties etc. (Also as a sort of meta-question: Is there a way how to find names of graphs in a simple way? Such as a OEIS for graphs?)

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ House of Graphs (hog.grinvin.org) can occasionally help you with this sort of question, but it is not nearly as large or well-established as the OEIS and it does not deal with multigraphs. $\endgroup$
    – D. Ror.
    Commented Mar 1, 2018 at 16:24
  • 2
    $\begingroup$ graphclasses.org has a rather extensive list of named graph classes; it is more browsable and less searchable than HoG and it also lacks multigraphs. $\endgroup$
    – D. Ror.
    Commented Mar 1, 2018 at 16:35
  • 1
    $\begingroup$ Because the number of possible variations explodes when multiple edges are allowed, you're unlikely to find much (if any) previous research on such a specific class as you've defined. $\endgroup$
    – D. Ror.
    Commented Mar 1, 2018 at 16:37
  • 2
    $\begingroup$ Off-the-cuff naming idea: (generally) multi-bound n-page clique-book; (specific to your case) 2-bound n-page K_4-book. $\endgroup$
    – D. Ror.
    Commented Mar 1, 2018 at 16:42
  • 1
    $\begingroup$ Personally I think that you cannot do better in this case than use the advice already given (essentially: to look at the much-studied class of 'book graphs', which your graphs are of course not isomorphic to), and for each and every result known about 'book graphs' investigate if and how the result changes on account of the one and only multiedge at the 'spine' of the book; incidentally, such a multiedge in graph theory is called a mathworld.wolfram.com/DipoleGraph.html, while in theoretical physics I have repeatedly read it referred to as 'banana graph'. $\endgroup$
    – Peter Heinig
    Commented Mar 1, 2018 at 18:50

1 Answer 1

Reset to default
3
$\begingroup$

In the case of simple graphs, you could search the House of Graphs (http://hog.grinvin.org) or browse the Information System on Graph Classes and their Inclusions (http://www.graphclasses.org).

Because the number of possible variations explodes when multiple edges are allowed, you're unlikely to find much (if any) previous research on such a specific class as you've defined. Here's one naming idea:

  • (in general) multi-spine clique-book;
  • (specific to your case) n-page 2-spine K_4-book.

Peter Heinig (2018-03-01, 18L15:20Z) suggested "for each and every result known about book graphs" (http://mathworld.wolfram.com/BookGraph.html), to "investigate if and how the result changes on account of one and only [one] multiedge at the spine of the book." He noted that a multiedge is sometimes called a dipole (http://mathworld.wolfram.com/DipoleGraph.html) or, in theoretical physics, a banana graph.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .