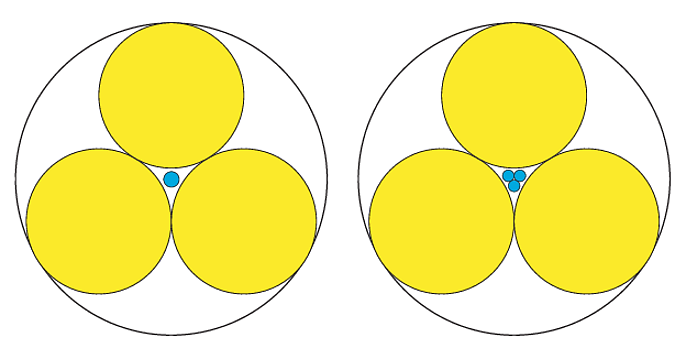
In the Euclidean plane, given is a collection of $k$ circular disks $D_1,…,D_k$ of radii $r_1,…, r_k$, supplied with weights $w_1,…,w_k$, assuming that each circle’s center of gravity coincides with the circle’s center (in general, the weights are independent from the radii). If the circles are packed in a circular container $D$ so that the center of gravity of their configuration coincides with the center of the container, then we say that the packing is balanced, and it is called the tightest balanced packing if the radius of the container is the smallest possible. The natural problem is, for a given finite family of weighted circular disks, to determine its tightest balanced packing. Generalizations to other shapes and higher dimensions are natural.
Question 1. Does anyone know of any references on this subject?
Some trivial inequalities. Let $r_b$ denote the minimum "balanced" radius, while $r$ - the minimum packing radius, no weights. Obviously, $r\le r_b$. But obviously as well, $r_b\le 2r$. A bit less obviously, $r_b<2r$ while $r_b$ can be arbitrarily close to $2r$.
Question 2. The natural case in which the weight of a disk coincides with its area, resp. volume, is of special interest: how much in this case can the radius of the container for a tightest balanced packing differ from the radius in the tightest packing without the balance requirement? To be more precise: what is the least upper bound on the ratio ${r_b}\over{r}$?