4
$\begingroup$

A set of Brunnian link is a nontrivial link such that if one component is removed, it becomes trivial. The best known example is the Borromean rings:

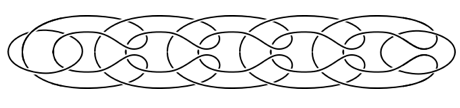
Here's a six component example:

There is likely a brunnian link with infinitely many components by generalizing the previous example.

My question is, is there a brunnian link with infinitely many components, such that each component has less $n$ crossings with other components, for some finite $n$? (By crossings, I mean crossings in some fixed link diagram of the link).

Improve this question
$\endgroup$
1
  • $\begingroup$ Why not replace "intersections" with "crossings"? $\endgroup$
    – PVAL
    Commented Jan 23, 2018 at 16:42

1 Answer 1

Reset to default
10
$\begingroup$

There are simpler diagrams of Brunnian links that have simple limits.

enter image description here

If you make an infinite chain of the C-shaped components, say periodically, then removing any one will let you ambient-isotope the others into separate balls.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Cool. These could probably arranged in a grid (by taking the connected sum of two C-shaped components), right? $\endgroup$
    – Christopher King
    Commented Jan 23, 2018 at 18:53
  • $\begingroup$ @PyRulez: You can make a doubly periodic version but the simplest ways would not be Brunnian. If you add to this, it would not completely unravel if you delete an added component. Perhaps you could spiral out from the center, though. $\endgroup$
    – Douglas Zare
    Commented Jan 23, 2018 at 19:33
  • 1
    $\begingroup$ By the way, you can make models of these using rubber bands plus one twist-tie. $\endgroup$
    – Douglas Zare
    Commented Jan 23, 2018 at 19:34
  • $\begingroup$ Is there a doubly periodic version that is Brunnian? $\endgroup$
    – Christopher King
    Commented Jan 23, 2018 at 20:01
  • $\begingroup$ @PyRulez: I suspect so, but I don't know of one yet. $\endgroup$
    – Douglas Zare
    Commented Jan 23, 2018 at 21:54

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .