1
$\begingroup$

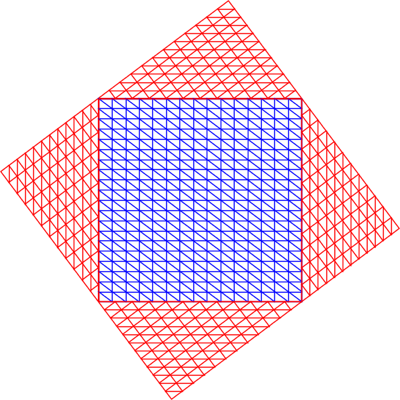
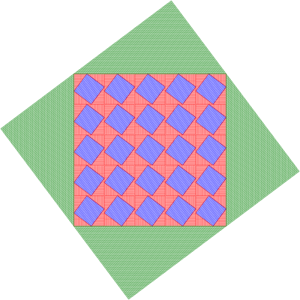
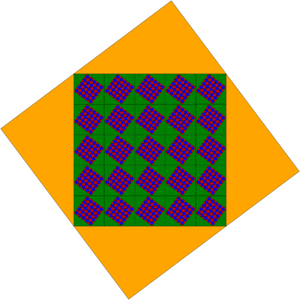
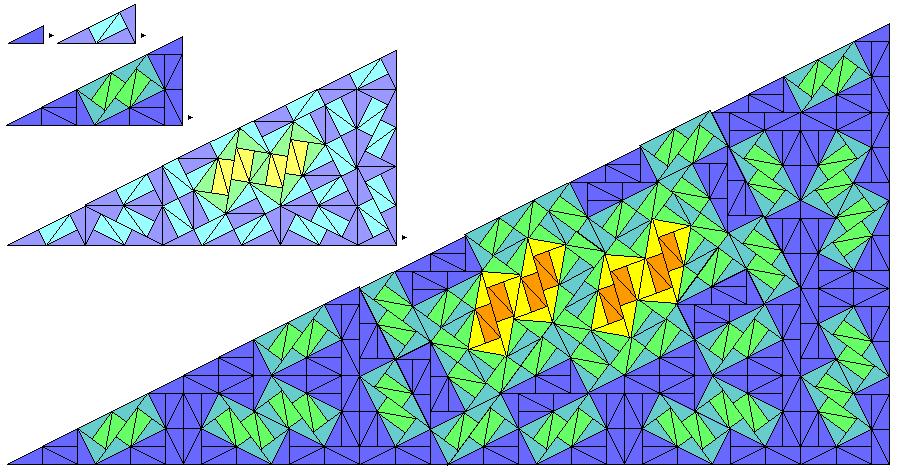
My recent question about polygonal tilings where tiles can occur in infinitely many positions has been answered by two nice constructions (besides Jan Kyncl's answer, there is the Conway tessellation evoked in Robin Houston's comment), which are both of self-similar nature. For each of the positions, there are only finitely many tiles, because each step of the constructions (in terms of self-similarity: each "change of scale") involves an irrational rotation of a so far finite pattern.

So I would like to push it further by asking:

  • Is there a tiling such that not only the number of positions is infinite, but that also all positions (or to start with, say just ONE position) occurs infinitely often?

Of course, for a given tile, say a pythagorean triangle, we can start with building a square that contains an arbitrary (but finite) number of different positions, then paving the plane with instances of that square repeats each position infinitely often. But this does not answer the question. As soon as self-similarity is involved, the "growth" happens in two dimensions. It does not seem possible e.g. to tile an infinite strip by some self-similar pattern.

Or is it?

Improve this question
$\endgroup$
5
  • 4
    $\begingroup$ I think that there are already infinitely many tiles in each position for those two constructions. It seems to me that in each iteration, the number of tiles in each distinct position gets multiplied by some factor > 1, so that in the limit, the number goes to infinity. $\endgroup$
    – j.c.
    Commented Dec 21, 2017 at 14:16
  • $\begingroup$ @j.c. Oh I see, the "factor > 1" is a good argument at least for the Conway pattern with the rightmost tile repeated twice (or three times if swapping the diagonal in the inner rectangle). But I still have to convince myself that the same holds for the other triangles in this configuration! $\endgroup$
    – Wolfgang
    Commented Dec 21, 2017 at 15:11
  • $\begingroup$ OK sure enough, in Jan Kyncl's construction, each step produces 25 instances of what is already there, I missed that. $\endgroup$
    – Wolfgang
    Commented Dec 21, 2017 at 15:22
  • 3
    $\begingroup$ For the pinwheel tiling, you can check that by the second iteration, each of the positions in the first iteration get realized by multiple tiles. See e.g. the images here quadibloc.com/math/til04.htm While there are new positions that appear in each iteration, I think you can argue that they will appear again with multiple tiles after two further iterations. $\endgroup$
    – j.c.
    Commented Dec 21, 2017 at 15:31
  • $\begingroup$ Yes, and thank you for the link to those nice pictures! :) $\endgroup$
    – Wolfgang
    Commented Dec 21, 2017 at 16:40

1 Answer 1

Reset to default
1
$\begingroup$

I may as well turn my comments into an answer:

There are infinitely many tiles in each position for both of the constructions in answers to your previous question.

In Jan Kyncl's construction, the number of tiles in each distinct position in iteration $j$ gets multiplied by 25, as is clear from these images:

picture of $Q'_2$ $Q'_3$$Q'_4$

For the pinwheel tiling, the images here, e.g. this one:

pinwheel tiling iterations

show that each of the positions in the $j$th iteration gets realized by multiple tiles in the $(j+2)$th iteration.

In both cases, in the limit, the number of tiles in each position goes to infinity.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .