Let $T$ be a polygon which tiles the plane. For an instance of $T$ (mirrored or not), call the set of its translates a position of $T$.
My question:
- How many different positions can occur in a tiling $\mathcal T$ of the plane?
Call this number $p(\mathcal T)$ and define for a tile $p(T):=\max_{\mathcal T} p(\mathcal T)$.
For example, the Voderberg tile has $p(T)\ge60$ and supposedly $p(T)=60$.
But is it even clear that $p(T)$ is always finite? Theoretically a tile containing irrational angles might occur in infinitely many positions, or is there an easy combinatorial argument against that?
A related probably easier question arises if we require $T$ to be convex.
- For convex tiles $T$, is $p(T)=12$ best possible?
The picture shows a tiling of a certain pentagon with $p(\mathcal T)=12$, where the $12$ marked tiles form a fundamental domain.
It comes from the bottom right tiling of this picture quoted here.
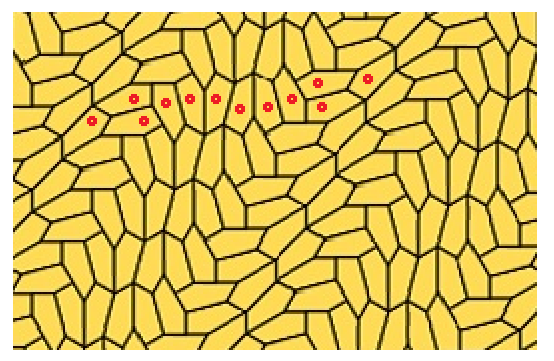 .
.
Are there similar constructions, possibly spiral tilings, with bigger $p(\mathcal T)$?
Note that the Voderberg tilings cannot be reduced to tilings with isoceles 12-degree triangles. But in fact, talking about triangles: by subdividing the regular hexagon paving, we see that the triangle $T$ with angles $30°,60°,90°$ has already $p(T)=12$, so there is no need for "sophisticated" tilings as the above to illustrate the (supposedly) extremal case. But I'll keep the picture because of the funny symmetries (with two privileged "directions") which that particular tiling exhibits.
- And of course we can ask similar questions for tilings in higher dimensions.