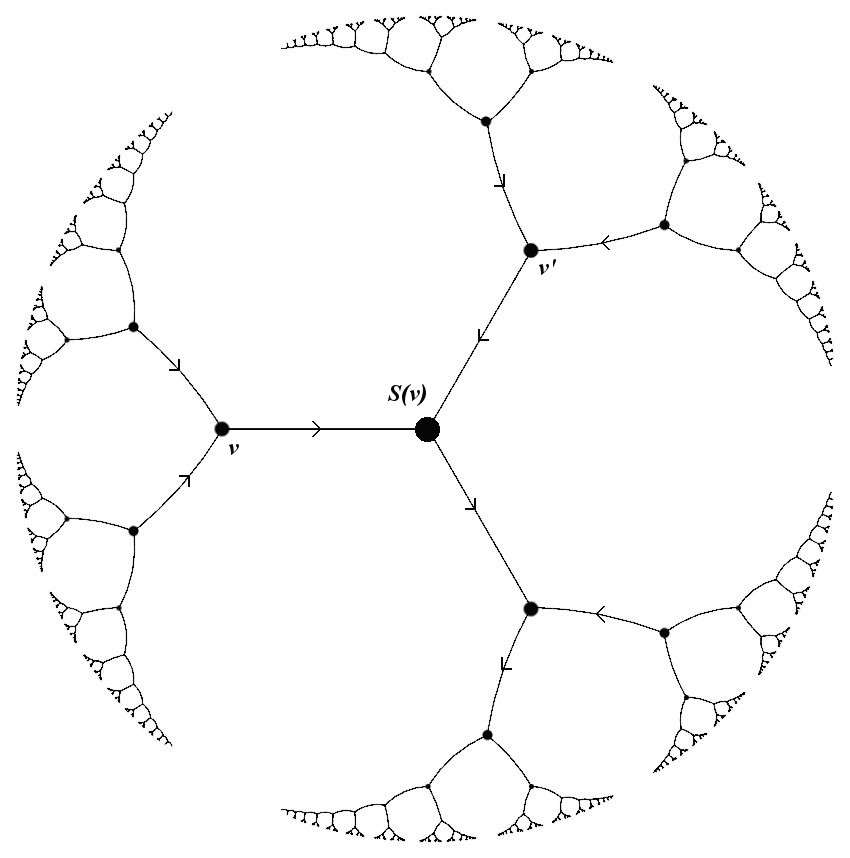
Let $\mathcal{T}$ be the trivalent directed tree, with two parents and one child for each vertex (see below). Let $\mathcal{V}$ be the set of vertices of $\mathcal{T}$ and $H$ be the Hilbert space $\ell^2(\mathcal{V})$. Let $S$ be the shift operator on $H$ defined so that $S(v)$ is the child of the vertex $v$. Note that $S$ is bounded and its adjoint $S^{\star}$ is given by reversing the direction of each edge.
Question: What is the von Neumann algebra generated by $S \in B(H)$ and denoted $W^{\star}(S)$?
Is it hyperfinite? Is it a factor? of each type?
Note that $W^{\star}(S)$ is not abelian because the operator $S$ is not normal, because for any vertex $v$, $$(SS^{\star}-S^{\star}S) \cdot v = v-v'$$ with $v'$ the other parent of the child of $v$.