3
$\begingroup$

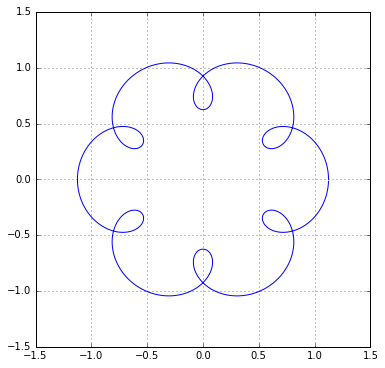
After playing with spirograph, a bit I realized all these curves I'm drawing should be an algebraic curve and it's birational equivalent to a $\mathbb{P}^1$. In the example below, I have a six-sided hypotrochoid. The equation is $z = e^{i\theta} ( \frac{7}{8} + \frac{1}{4} e^{6i\theta}) $, so this already gives a (birational?) map from $S^1 \to \mathbb{C}$.

I learned one way to estimate the degree of this map is to intersect a line (or hyperplane divisor) and computing the number of intersection point (the degree)

Typical solutions in algebraic geometry involve deforming the problem to one that is more generic. Or complexifying the problem.

The intersection I am looking for (a bitangent) is not generic and I believe this curve is singular (at least it has self-intersections).

So far, I have counted about 48 or 50 (by literally) drawing with a pencil. I will try to sketch my partial results. This question certainly has a finite, closed-ended meaningful answer. This certainly falls under classical enumerative algebraic geometry (such as the Schubert calculus).

enter image description here

Improve this question
$\endgroup$
6
  • $\begingroup$ dumb question- are you in $(z,\theta) $ space or what are your coordinates $\endgroup$
    – meh
    Commented Dec 16, 2017 at 14:53
  • 1
    $\begingroup$ Have you tried finding the equatio of the dual curve? You are counting its nodes. $\endgroup$
    – Mariano Suárez-Álvarez
    Commented Dec 16, 2017 at 15:04
  • $\begingroup$ My strategy was going to "integrate" across the space of lines in the $z = x + iy$ plane and count the intersections that way. We know the curve should be a sextic since the "generic" line intersects the curve at 6 points. But this is algebraic geometry over $\mathbb{R}$ and there are significant portions of lines that intersect with fewer pts or have non-typical intersections. This is how we were led to the discussion of bitangents. $\endgroup$
    – john mangual
    Commented Dec 16, 2017 at 15:11
  • $\begingroup$ Here are two blogs [ 1 , 2 ] on dual curves, and they indicate a relation between nodes (on one dual) and bitangents (on the original curve). Doesn't look any easier. $\endgroup$
    – john mangual
    Commented Dec 16, 2017 at 15:35
  • 1
    $\begingroup$ Use this question with $d=14$ (see my answer to this question on MSE) to get a bound of 14784. $\endgroup$
    – Jan-Magnus Økland
    Commented Dec 16, 2017 at 16:19

2 Answers 2

Reset to default
3
$\begingroup$

Too long for a comment. Using GKZ p. 17, a parametric representation of the dual curve is: $$X={\frac {8}{7}}\,{\frac {\cos \left( \theta \right) \left( -7+128\, \left( \cos \left( \theta \right) \right) ^{6}-224\, \left( \cos \left( \theta \right) \right) ^{4}+112\, \left( \cos \left( \theta \right) \right) ^{2} \right) }{288\, \left( \cos \left( \theta \right) \right) ^{2}+512\, \left( \cos \left( \theta \right) \right) ^{6}-768\, \left( \cos \left( \theta \right) \right) ^{4}-5} }$$

$$Y=8\,{\frac { \left( -1+128\, \left( \cos \left( \theta \right) \right) ^{6}-160\, \left( \cos \left( \theta \right) \right) ^{4}+48 \, \left( \cos \left( \theta \right) \right) ^{2} \right) \sin \left( \theta \right) }{288\, \left( \cos \left( \theta \right) \right) ^{2}+512\, \left( \cos \left( \theta \right) \right) ^{6}- 768\, \left( \cos \left( \theta \right) \right) ^{4}-5}}$$

And as @MarianoSuárez-Álvarez says; nodes $(X,Y)$ corresponds to bitangents $Xx+Yy+1=0$ to your curve.

dual hypo

Improve this answer
$\endgroup$
1
$\begingroup$

I've never posted here before and meant to post this as a comment but accidentally posted it as an answer. Apologies.

A very naive, elementary approach: No line through the origin is a bitangent, so every bitangent has a leftmost point of tangency as viewed from the origin. Fix an arc A such that the curve is the disjoint union of A and 5 congruent copies of A. Count all bitangents whose leftmost point of tangency is on A and multiply by 6.

To count all bitangents whose leftmost point of tangency is on A, consider a point P which moves from one end of A to the other, and note all possible positions for P for which the tangent at P could also be a tangent somewhere to the right of P.

Robert Hill

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .