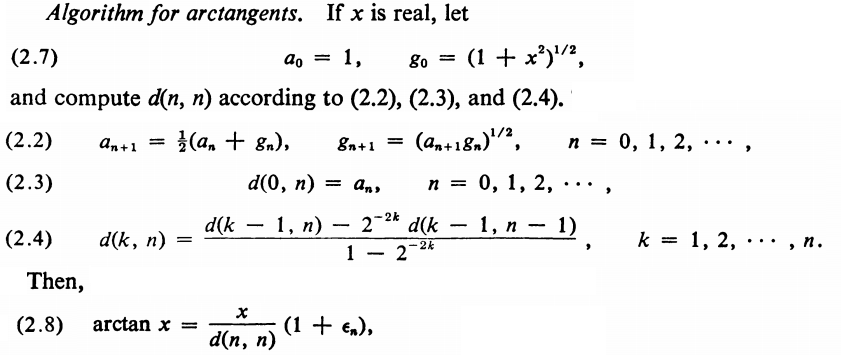In the article An algorithm for computing logarithms and arctangents, by B. C. Carlson, the following iterative algorithm for arctangents is given:
The algorithm uses that $2^n\tan(2^{-n}\arctan(x))=\arctan(x)+e(4^{-n})$ where the coefficients of the power series $e$ do not depend on $n$, and uses Richardson extrapolation to accelerate the convergence by increasing the convergence order.
More precisely, with $x=\tan(t)$ one has $a_n=\frac{x}{2^n\tan(2^{-n}t)}$, $g_n=\frac{x}{2^n\sin(2^{-n}t)}$. Both converge towards $\frac{x}{t}=\frac{x}{\arctan x}$. The error $a_n-\frac{x}{t}$ is a power series in $4^{-n}$ with coefficients depending on $x$. The derived sequences from the Richardson extrapolation are denoted as $d_{n,k}=d(n,k)$.
To replicate this idea for the tangent computation, one would need to find some iterative procedure for the computation of $t_n= 2^n\arctan(2^{-n}\tan x)$ as then $\tan x=t_n+e(4^{-n})$ would again have an error term that has constant coefficients for the powers of $4^{-n}$ so that convergence can be sped up using Richardson extrapolation. How this can be done?
Is it possible to apply some inversion process to this algorithm to generate an algorithm to compute the function $\tan x$?