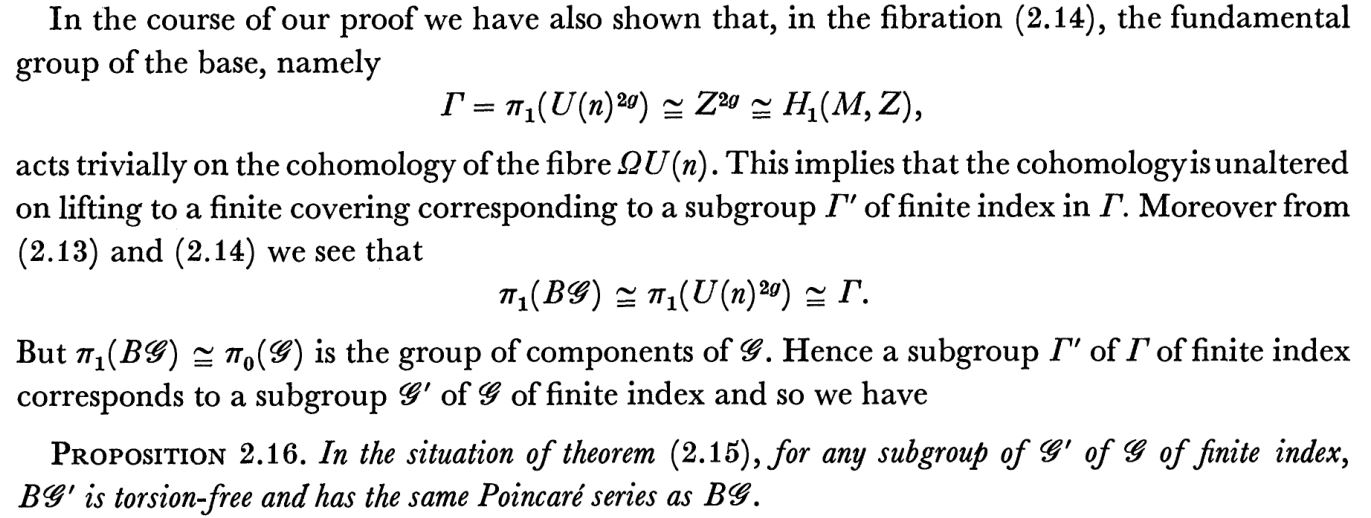
I am reading Atiyah and Bott's classic paper "Yang-Mills Equations over Riemann surfaces" and struggling with proposition 2.16 (p. 542)
Let $P$ be a principal $U(n)$-bundle over a compact Riemann surface $M$. Let $\mathscr{G}=\mathrm{Aut}(P)$ be the gauge group. They show that $\mathscr{G}=\mathrm{Map}_P(M,BU(n))$ which is the component in $\mathrm{Map}(M,BU(n))$ that determines $P$. Using this, they compute the Poincare polynomial of $B\mathscr{G}$. Then, they use the following two fibrations
$$\mathrm{Map}^*(M,BU(n))\to\mathrm{Map}(M,BU(n))\to BU(n)$$ $$\Omega U(n)\to\mathrm{Map}^*(M, BU(n))\to U(n)^{2g}$$ to show that $B\mathscr{G}$ has no torsions. Moreover, the fundamental group $\Gamma=\pi_1(U(n)^{2g})$ acts trivially on the cohomology of $\Omega U(n)$
Then, they use the following argument that are really confusing to me (2.13 and 2.14 are two fibrations mentioned above):
So, here is my question:
What does it mean by "This implies that the cohomology is unaltered on lifting to a finite covering corresponding to a subgroup $\Gamma'$ of finite index $\Gamma$"? Whose covering space and cohomology are they referring to? Why is this cohomology unaltered? If I am assuming they are talking about the cohomology of the covering space of $U(n)^{2g}$, then how does it relate to the proposition 2.16?
Thank you!