0
$\begingroup$

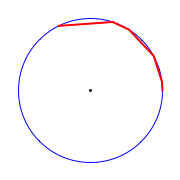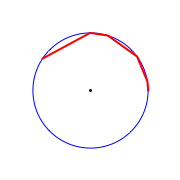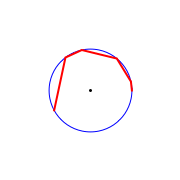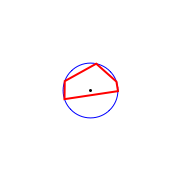
Suppose we break a stick of length one at four randomly and independently chosen points and that the resulting pieces form a pentagon.

Such a pentagon can be formed with probability $1-(5/16) = {11\over16}$ (see https://atlas.mat.ub.edu/personals/dandrea/emiliano_gomez.ps, which states that an $n$-gon is formed from $n-1$ breaks with probability $1-{n\over2^{n-1}}$).

Using this distribution of lengths and assuming that a cyclic pentagon has been formed, what is the expected value of the pentagon's area?

Improve this question
$\endgroup$
8
  • 1
    $\begingroup$ Are there reasons to expect this value to be computable in 'elementary functions and standard constants'? $\endgroup$
    – Fedor Petrov
    Commented Nov 13, 2017 at 20:44
  • 2
    $\begingroup$ Cyclic pentagon means vertices on a circle, right? If that is the case, then the area is uniquely determined by the side lengths - draw the 5 lines from the circle center, and you get 5 equilaterial triangles, whose sum of areas is independent of how (the order) you glue together the pieces. $\endgroup$
    – Per Alexandersson
    Commented Nov 13, 2017 at 22:17
  • 1
    $\begingroup$ @PerAlexandersson: You mean 5 isosceles triangles. The fact that your interpretation makes the area well-defined (which it otherwise isn't) makes me think your interpretation of "cyclic" is the right one. $\endgroup$
    – Hugh Thomas
    Commented Nov 14, 2017 at 4:55
  • 5
    $\begingroup$ I think, "cyclic" is a standard term for a convex polygon inscribed in the circle. It is not obvious at all, but there is unique cyclic $n$-gon with given length sides (each of which is less than the sum of the others) in a given order, and its area does not depend on the order of sides (this already is clear: permute the segments cut by the sides.) $\endgroup$
    – Fedor Petrov
    Commented Nov 14, 2017 at 7:09
  • 2
    $\begingroup$ I wonder why questions like that pop up with astonishing regularity? The answer is always the same: one can set up the integral (in this case the OP decided to go beyond quadrilaterals and thus challenge us with either implicit functions or parametric representations; as to myself, I prefer the latter) and evaluate it numerically. So, the answer is a long string of decimal digits that you can figure out up to any length you can memorize, given some cheap computer and minimal programming skills. No need to bother other people. Voting to close. $\endgroup$
    – fedja
    Commented Nov 15, 2017 at 3:01

1 Answer 1

Reset to default
1
$\begingroup$

Not an answer, just an illustration of the uniqueness as mentioned in Fedor Petrov's comment. Start with a large radius $r$, and inscribe the segments along the arc of the circle. Then shrink $r$ until the ends meet:

          Pent.gif
          Edge lengths: $1, 3, 4, 2, 6$. Final circle radius $\approx 3.045$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ (The image should animate twice; if not, click on it.) $\endgroup$
    – Joseph O'Rourke
    Commented Nov 14, 2017 at 17:52

Not the answer you're looking for? Browse other questions tagged .