Let $L$ be an oriented homogeneous link and let $D$ be an oriented diagram of $L$ wich is not necessarily a homogeneous diagram. Fix some crossing $c$ in $D$ and construct the diagram $D_0$ by smoothing $c$.
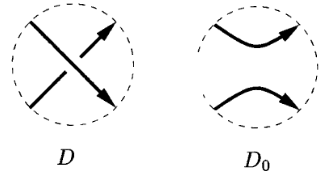
Now let $L_0$ be the oriented link represented by $D_0$. Is $L_0$ also a homogeneous link ?
No. Any two links are related by repeated applications of that move.
To see this, take a braid presentation of $L$, i.e. a braid word $\beta$ whose closure is $L$. At some point, insert $\sigma_i \sigma_i^{-1}$ into $\beta$. This does not change the closure. Now the above move allows one to remove $\sigma_i^{-1}$. Effectively, we have inserted $\sigma_i$ somewhere in the braid word. Clearly, by repeatedly doing this, we can reach any braid word and thus any link.
