3
$\begingroup$

This is a crosspost from Math.SE.

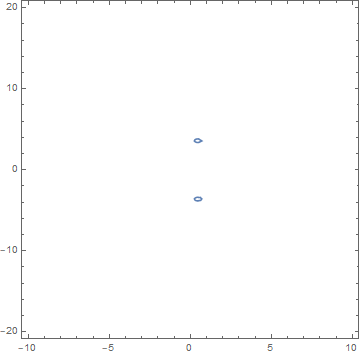
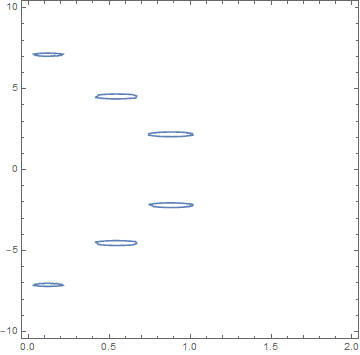
Is there a known increasing sequence of positive integers $\{\textbf{a}\} = a_0<a_1<a_2<.....$ such that all the zeros $z_k$ on $\Re[z]>0$ of the complex function $F(z;\{\textbf{a}\})= \frac{1}{a_0^z}+\frac{1}{a_1^z}+\frac{1}{a_2^z}+.......$ with $F(z_k;\{\textbf{a}\})=0$ are such that $\Re[z_0]=\Re[z_1]=\Re[z_2]=... =c$ for some real number $c$? Also, if we are given an arbitrary real $c>0$ is there always a sequence $\{\textbf{a}\}$ with this property? Thank you.

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ In general this converges for $\Re z>1$. Do we consider a meromorphic continuation of this function, or what? $\endgroup$
    – Fedor Petrov
    Commented Oct 18, 2017 at 14:11
  • $\begingroup$ @Fedor_Petrov I left that important detail out intentionally, I just wanted to know if it is possible at all that kind of distribution of zeros for some integer sequence. This seems to be an "easier" question than the Riemann hypothesis with specific $c=\frac{1}{2}$ and $\textbf{a} = 1,2,3,...$ . If assuming meromophy helps, so be it, whatever works (an engineer's view of the problem)! $\endgroup$
    – hyportnex
    Commented Oct 18, 2017 at 16:57
  • $\begingroup$ If you want an easier question forget about strictly increasing sequences of integers and construct analogs of $\frac{-\zeta'(s)}{\zeta(s)} = \sum_{n=1}^\infty \frac{\Lambda(n) }{n^{s}} =\sum_{n=2}^\infty \frac{\psi(n+\frac12)-\psi(n-\frac12)}{n^{s}}$ $=\sum_{n=2}^\infty \frac{1- \sum_\rho \frac{(n+\frac12)^\rho-(n-\frac12)^\rho}{\rho}}{n^{s}}$ where $\rho$ are the non-trivial and trivial zeros $\endgroup$
    – reuns
    Commented Oct 19, 2017 at 22:37

3 Answers 3

Reset to default
3
$\begingroup$

An example which is almost trivial: take the sequence $\{1,4,8,16,32\ldots\}$, i.e. powers of $2$ omitting two itself. For real part of $s>0$, the Dirichlet series is a convergent geometric series, summing as $$ \frac{1-2^s+4^s}{1-2^s} $$ which has a meromorphic continuation. The zeros are all purely imaginary, at $$ \frac{\pi i(6n\pm1)}{3\log(2)},\qquad \text{integer } n $$ if I've done the calculation correctly.

Building on this, one can sum $$ 1+2^{-s}+\sum_{k=3}^\infty 2^{-ks}=\frac{-2^{-2 s} \left(1-2^s+2^{3 s}\right)}{1-2^s}. $$ The numerator has a term which is a cubic in 2^s. A Mathematica computation then gives three infinite sets of zeros, one along the line with real part equal $$ \frac{\log \left(\sqrt[3]{\frac{2}{3 \left(9-\sqrt{69}\right)}}+\frac{\sqrt[3]{\frac{1}{2} \left(9-\sqrt{69}\right)}}{3^{2/3}}\right)}{\log (2)}\approx 0.405685 $$

Improve this answer
$\endgroup$
3
  • $\begingroup$ This is all true but what about a function having zeros that are on the right half plane $\Re[z]>0$ (have positive real parts) as I was asking in my question admittedly with some tortured grammar. $\endgroup$
    – hyportnex
    Commented Oct 20, 2017 at 19:35
  • $\begingroup$ @hyportnex see revision. $\endgroup$
    – Stopple
    Commented Oct 20, 2017 at 20:30
  • $\begingroup$ You are right! This has its zeros at two lines parallel with the imag axis, one at $c \approx 0.405$ and the other at $c \approx -0.202$. So then I should rephrase my question: could you find a sequence so that its zeros are only either on the negative real axis and/or a single line parallel with the imag axis on the RHS? $\endgroup$
    – hyportnex
    Commented Oct 20, 2017 at 22:50
1
$\begingroup$

Can we not proceed in a manner similar to how Riemann did with the Mellin transform?

For example, let me define $\theta(u) = \sum_{n=0}^{\infty}e^{-\pi a_n^2u}$

$$\int_0^{\infty} \theta(y) y^{s/2} dy/y = \int_0^{\infty}\sum_{n=0}^{\infty}e^{-\pi a_n^2y} = \sum_{n=0}^{\infty} \int_0^{\infty}e^{-\pi a_n^2y} y^{s/2} dy/y $$

$$= \pi^{-s/2} \sum_{n \ge 0} \frac{1}{a_n^s} \int_0^{\infty}e^{-u} u^{s/2} du/u$$ (after substituting $\pi a_n^2 y = u$)

$$= \pi^{-s/2} \Gamma(s/2) F(s)$$

I don't know if Jacobi's identity $\theta(u) = u^{-1/2}\theta(1/u)$ holds or not. It is probable that the identity holds, and then the functional equation will look similar to RFE and then one can probably go on to guess all zeros have real part $1/2$.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Of course for $a_n$ integers $\theta(u) = u^{-1/2}\theta(1/u)$ doesn't hold except in some very particular cases $\endgroup$
    – reuns
    Commented Oct 28, 2017 at 5:58
  • $\begingroup$ Good point. You think periodicity is the property that we need? If the sequence is periodic, then JI may hold true. $\endgroup$
    – sku
    Commented Oct 28, 2017 at 6:04
  • $\begingroup$ If $a_{n+k} = a_n$ and are integers then yes $\theta$ is modular of weight $1/2$ for some group, not necessary containing any $u \mapsto m/u$ $\endgroup$
    – reuns
    Commented Oct 28, 2017 at 6:08
0
$\begingroup$

Just a remark, this is true for any two element sequence (no, it's not infinite).

For example

$$1 + \frac{1}{2^s}.$$ All zeros have real part zero.

A less obvious experimental fact is that the same (the zeros lie on a vertical line, not zero) is true for

$$1 + \frac{1}{2^s} + \frac{1}{3^s}.$$

Here is the picture from mathematica (contour lines of $|f| = 0.2,$ in case you are wondering).

enter image description here

The next picture is what happens when you have the $\sum_{k=1}^{10} k^{-s}.$ enter image description here

Improve this answer
$\endgroup$
10
  • $\begingroup$ and a more obvious fact is that it holds for $\{1,m,n,mn\}$ (with $1<m<n$). $\endgroup$
    – Noam D. Elkies
    Commented Oct 19, 2017 at 22:40
  • $\begingroup$ ( . . . as reuns noted a few seconds before me in the case $(m,n)=(2,3)$; but Igor Rivin probably does mean just $\{1,2,3\}$, not $\{1,2,3,6\}$.) $\endgroup$
    – Noam D. Elkies
    Commented Oct 19, 2017 at 22:41
  • $\begingroup$ Did you mean $1 + \frac{1}{2^s} + \frac{1}{3^s}+\frac{1}{6^s}$ ? I think in "the structure of the Selberg class" they proved such Dirichlet polynomials need to be of the form $\alpha\sum_{d |n} d^{c-s} (a_d+d^b a_{n/d})$ (if the zeros of a Dirichlet polynomial are all on $\Re(s) = \sigma$ then $\log P(s)$ is almost invariant under $\sigma+s \to \sigma-s$. How can it be the case for $1+2^{-s}+3^{-s}$ ?) $\endgroup$
    – reuns
    Commented Oct 19, 2017 at 22:43
  • 1
    $\begingroup$ Of course the first two zeros have the same real part because they're complex conjugates. But in general the real parts can fall anywhere between $-1$ and the positive root $.7878849\ldots$ of $2^{-\sigma} + 3^{-\sigma} = 1$. Applying Newton's method to the first $10$ odd multiples of $\pi i \, / \log 3$ finds complex roots with real parts approximately $$ 0.4544,\; -.9406,\; .7332,\; -.1326,\; -.4565,\; .7798,\; -.7860,\; .2357,\; .6106,\; -.9992 \;. $$ $\endgroup$
    – Noam D. Elkies
    Commented Oct 20, 2017 at 1:41
  • 1
    $\begingroup$ Thank you for taking my question seriously. Originally, I was hoping to learn about an infinite series with all zeros on a line on the right half plane but I have no idea who downvoted your attempt to solve it. If you and @NoamD.Elkies and others in this forum do not have a ready answer then may surmise that this problem has not been explored? This is actually quite surprising. $\endgroup$
    – hyportnex
    Commented Oct 20, 2017 at 19:46

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .