Analytic formula and an exponential bound
For $r \in [0, 1]$, define $\tau_m(r) \in [0, 1]$ by
$$
\tau_m(r) := \frac{1}{\lambda(B_m(r))}\int_{B_m(r)} \frac{\lambda(B_m(1) \cap (B_m(1) + x))}{\lambda(B_m(1))}dx.
$$
The case $r=1$ has been analysed in accepted answer. Extending user @Matt's comment, one can establish that (for the core idea, see this post)
$$
\begin{split}
\tau_m(r) &= \frac{1}{V_m^{cap}(r, 0)}\int_{0}^r ms^{m-1}V_m^{cap}(1, s/2)ds = \frac{1}{r^mV_m^{cap}(1, 0)}\int_{0}^r ms^{m-1}V_m^{cap}(1, s/2)ds\\
&= \frac{1}{V_m^{cap}(1, 0)}\int_{0}^1 ms^{m-1}V_m^{cap}(1, rs/2)ds,
\end{split}
$$
where $V_m(r; h)$ is the volume (i.e Lebesgue measure) of the half-lens $\{x \in B_m(r) \mid x_1 \ge h\}$.
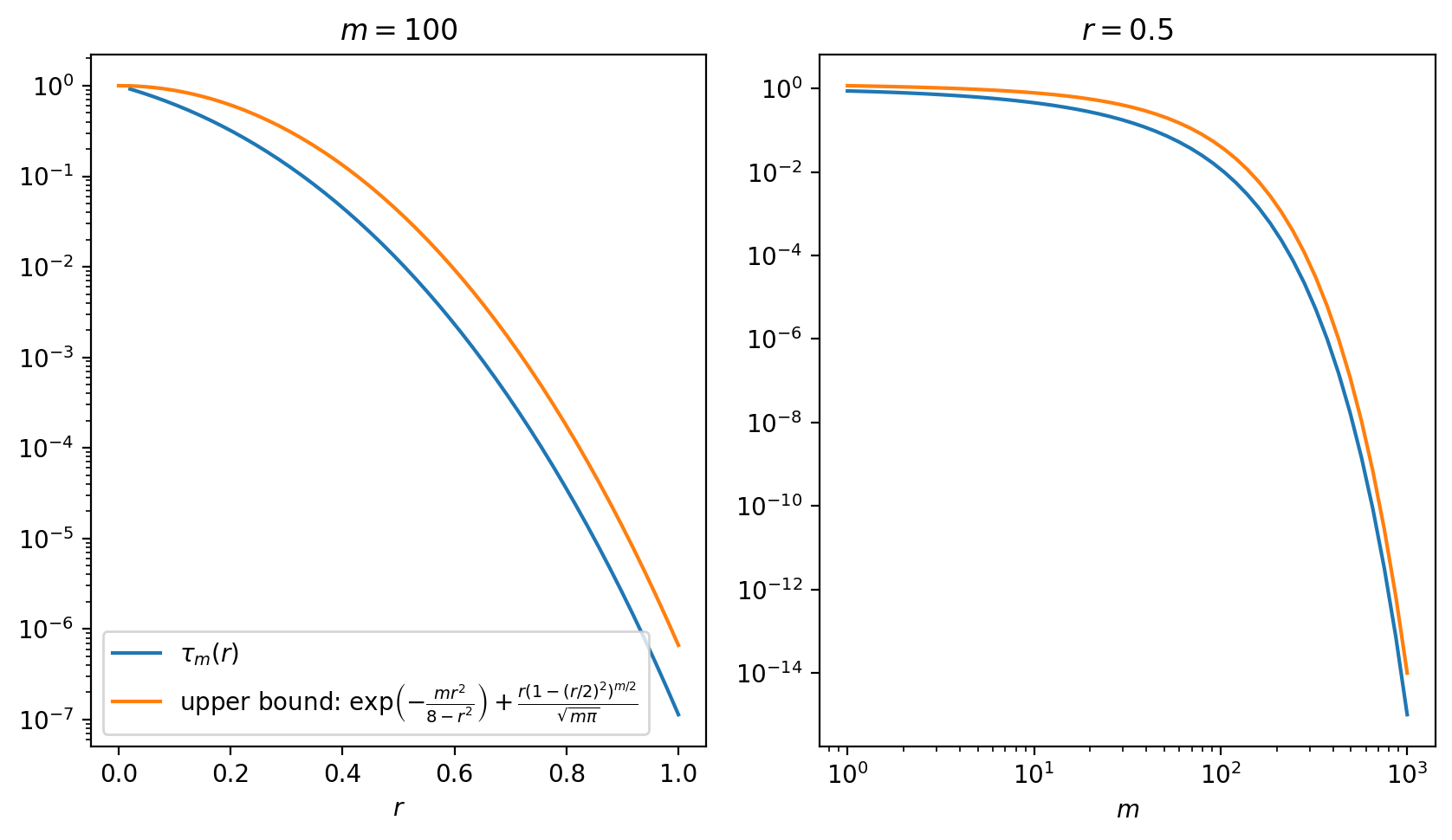
Preamble. I propose to obtain an analytic formula for $\tau_m(r)$ in terms of special functions (mostly hypergeometric functions). We will also obtain a good upper bound for $\tau_m(r)$.
The end result will be the following exponential decay of $\tau_m(r)$ as a function on the dimension $m$.
Theorem. For every $r \in [0, 1]$ and large integer $m$, we have the upper bound
$$
\begin{split}
\tau_m(r) &\lesssim \exp\left(-\frac{mr^2}{8-r^2}\right) + \frac{r(1-r^2/4)^{m/2}}{\sqrt{m\pi}}\\
&\lesssim \exp\left(-\frac{mr^2}{8}\right) + \frac{r(1-r^2/4)^{m/2}}{\sqrt{m\pi}}.
\end{split}
$$
 Proof.
Now, it is a classical computation that for $h \ge 0$ we have $V_m^{cap}(1, h) = \frac{\lambda(B_{m - 1}(1))}{2}\beta_{1-h^2}\left(\frac{m+1}{2},\frac{1}{2}\right)$, where $\beta_x(a, b) := \int_0^x t^{a-1}(1-t)^{b-1}dt$ defines the incomplete beta function. In particular,
$V_m^{cap}(1, 0) = \frac{\lambda(B_{m - 1}(1))}{2}\beta_{1}\left(\frac{m+1}{2},\frac{1}{2}\right)$.
Proof.
Now, it is a classical computation that for $h \ge 0$ we have $V_m^{cap}(1, h) = \frac{\lambda(B_{m - 1}(1))}{2}\beta_{1-h^2}\left(\frac{m+1}{2},\frac{1}{2}\right)$, where $\beta_x(a, b) := \int_0^x t^{a-1}(1-t)^{b-1}dt$ defines the incomplete beta function. In particular,
$V_m^{cap}(1, 0) = \frac{\lambda(B_{m - 1}(1))}{2}\beta_{1}\left(\frac{m+1}{2},\frac{1}{2}\right)$.
Thus, integrating by parts, we get
$$
\begin{split}
V_m^{cap}(1,0)\tau_m(r) &= \int_{0}^1 ms^{m-1}V_m^{cap}(rs/2)ds = \int_{0}^1 \beta_{1-(rs)^2/4}\left(\frac{m+1}{2},\frac{1}{2}\right)ds^m\\
&= \left[s^m\beta_{1-(rs)^2/4}\left(\frac{m+1}{2},\frac{1}{2}\right)\right]_0^1 + r\int_0^1 s^m(1-(rs)^2/4)^{\frac{m-1}{2}}ds \\
&= \beta_{1-(r/2)^2}\left(\frac{m+1}{2},\frac{1}{2}\right) + R_m,
\end{split}
\tag{1}
$$
where $R_m := r\int_0^1 s^m(1-(rs/2)^2)^{\frac{m-1}{2}}ds$. Now, consider the change of variable $s = 2\sqrt{t}$. This gives $s^m(1-s^2/4)^{(m-1)/2}ds=2^mt^{m/2}\cdot(1-t)^{(m-1)/2}\cdot t^{-1/2}dt = 2^mt^{(m-1)/2}(1-t)^{(m-1)/2}dt$. Thus,
$$
\begin{split}
R_m &= r\int_0^1 s^m(1-(rs)^2/4)^{\frac{m-1}{2}}ds = \frac{2^m}{r^m}\int_0^{(r/2)^2}t^{(m-1)/2}(1-t)^{(m-1)/2}dt\\
&=: \frac{2^m}{r^m}\beta_{(r/2)^2}\left(\frac{m+1}{2},\frac{m+1}{2}\right).
\end{split}
\tag{2}
$$
Combining (1) and (2) then gives the analytic formula,
Analytic formula. For any $r \in [0, 1]$ and positive integer $m$, it holds that
$$
\tau_m(r) = I_{1-(r/2)^2}\left(\frac{m+1}{2},\frac{1}{2}\right) + \frac{(2/r)^m\beta_{(r/2)^2}\left(\frac{m+1}{2},\frac{m+1}{2}\right)}{\beta_1\left(\frac{m+1}{2},\frac{1}{2}\right)},
$$
where $u \mapsto I_u(a, b) := \beta_u(a,b) / \beta_1(a, b)$ is the CDF of $(a,b)$-beta distribution.
Bounding the first term.
Let $\delta := (r/2)^2$ and $p = I_{1-\delta}((m+1)/2,1/2)$.
It is known since Temme (1992) that for $p \in (0, 1)$ and large $a > 0$, the solution of the equation $p = I(t; a,b)$ is given (approximately) by
$$
t=t_p(a, b) \approx e^{-(1/a)Q_{1-p}(\Gamma(b,1))}, \tag{4}
$$
where $Q_{1-p}(\Gamma(b,1))$ is the $1-p$ quantile of the unit-scale gamma distribution with shape parameter $b$. Now by standard concentration results (e.g see Boucheron et al. textbook),
$$
Q_{1-p}(\Gamma(b,1)) \le \log(1/p) + \sqrt{2b\log(1/p)}. \tag{5}
$$
In particular, for $a=(m+1)/2$ and $b=1/2$ we get
$$
Q_{1-p}(\Gamma(1/2,1)) \le \log(1/p) + \sqrt{\log(1/p)}. \tag{6}
$$
Putting (2), (4), and (6) together and using the basic inequality $1-e^{-z} \le 2z/(2+z)\;\forall z \ge 0$, we see that
$$
\begin{split}
\delta &= 1 - t_{p}\left((m+1)/2,1/2\right) \approx 1-e^{-\frac{2Q_{1-p}(\Gamma(1/2,1))}{m+1}} \le 1-e^{-\frac{2}{m+1}\left(\log\left(\frac{1}{p}\right) + \sqrt{\log\left(\frac{1}{p}\right)}\right)} \le \frac{2\alpha}{2+\alpha},
\end{split}
$$
where $\alpha = \frac{2}{m+1}(\log(1/p)+\sqrt{\log(1/p)})$. Thus, $\alpha \ge \delta/(1 - \delta/2)$, and this wolfram-alpha query tells us that we must have $p \lesssim \exp(-mr^2/(8-r^2))$. Putting things together then gives
$$
\begin{split}
I_{1-(r/2)^2}((m+1)/2,1/2) = p \lesssim \exp(-mr^2/(8-r^2)) \le \exp(-mr^2/8).
\end{split}
$$
Bounding the second term. It is a well-known result that
$$
\beta_z(a,b) \equiv a^{-1}z^a{}_2F_1(a,1-b,a+1;z),
$$
where $z \mapsto {}_2F_1(u, v, w; z)$ is the hypergeometric function. Thus, one computes
$$
\begin{split}
(2/r)^m\beta_{(r/2)^2}\left(\frac{m+1}{2},\frac{m+1}{2}\right) &= \frac{r}{m+1}{}_2F_1\left(\frac{m+1}{2},\frac{1-m}{2},\frac{m+3}{2},\frac{r^2}{4}\right) \\
&= \frac{r}{m+1}{}_2F_1\left(\frac{1-m}{2},\frac{m+1}{2},\frac{m+3}{2},\frac{r^2}{4}\right)\\
&\approx \frac{r}{m+1}{}_2F_1\left(\frac{1-m}{2},\frac{m+1}{2},\frac{m+1}{2},\frac{r^2}{4}\right)\\
&= \frac{r}{m+1}(1-r^2/4)^{(m-1)/2},
\end{split}
$$
where we have used the powerful identity ${}_2F_1(u, v, v; z) \equiv (1 - z)^{-u}$.
On the other hand, Stirling's formula gives
$$
\beta_1\left(\frac{m+1}{2},\frac{1}{2}\right) \approx \Gamma(1/2)\left(\frac{m+1}{2}\right)^{-1/2} = \sqrt{\frac{\pi}{m+1}}.
$$
Thus,
$$
\frac{(2/r)^m\beta_{(r/2)^2}\left(\frac{m+1}{2},\frac{m+1}{2}\right)}{\beta_1\left(\frac{m+1}{2},\frac{1}{2}\right)} \approx \frac{r(1-(r/2)^2)^{(m-1)/2}}{\sqrt{\pi(m+1)}}
$$
Putting every together then completes the proof of the theorem. $\quad\quad\quad\Box$

