16
$\begingroup$

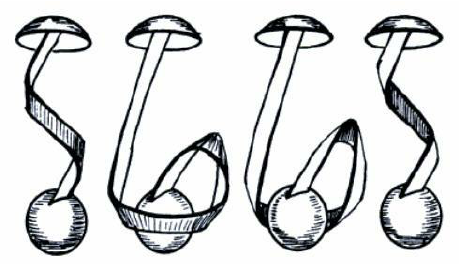
The question is triggered by the wonderful animations by Jason Hise:

https://www.youtube.com/watch?v=LLw3BaliDUQ

https://www.youtube.com/watch?v=6Ul_-ABYaYU

https://www.youtube.com/watch?v=aYVt1UiERIQ

All these animations are based on the well-known belt trick (a way to represent SU(2) as double cover of SO(3)) and suggest that a solid sphere that is glued into a soft mattress can rotate continuously, if the region of mattress close to the sphere performs certain appropriate motions around the sphere. Is this conclusion correct? Are there papers on this very counter-intuitive issue?

The belt trick is shown impressively in https://youtu.be/DHFdBWU36eY . It is also called the plate trick, the string strick, the balinese dance trick or the balinese candle trick. The trick shows that an object connected to spatial infinity can rotate indefinitely, coming back to its original position after a rotation by 4 pi = 720 degrees.

It is not evident that the belt trick implies that a sphere can rotate when glued in a mattress. Many discrete belts do not make a continuous and smooth mattress. So it could be that the mattress property does not follow from the belt trick - it could be that the belt trick requires discontinuities. So the question is: is the mattress trick a continuous operation on the mattress, or does it require cuts in the mattress?

Did anybody ever perform the experiment in real life? For two dimensions, this has been done, as shown in this video: https://www.youtube.com/watch?v=UtdljdoFAwg that shows that a ball glued into a handkerchief can be rotated continuously. But it is really possible also for three dimensions, for a full mattress?

The issue is interesting because a ball in a mattress can be seen, if this works, as a model for a spin 1/2 particle. Above all, if we assume that the mattress is a model for space itself, the ball in the mattress would be a way to model a spin 1/2 particle with the help of a smooth 3-dimensional manifold, something which is often assumed to be impossible. It would solve one of the contradictions between general relativity and quantum theory.

The newest animation by Jason Hise of "Dirac's handkerchief", made after this question was posted, is here: https://youtu.be/tazjVJcxm50 It realizes the answer of André Henriques. The answer to the original question is thus a clear "yes".

If anybody every manages to do this in an experiment, I'd like to see the video -- and to put it into my free physics text!

Improve this question
$\endgroup$
3
  • $\begingroup$ If you actually try this with a ball and a table cloth (or, say, a bed sheet) you will need to have a good bit of slack. $\endgroup$
    – Colin McLarty
    Commented Sep 25, 2017 at 13:40
  • $\begingroup$ Motion Mountain: If both accounts belong to you, you could probably tried to merge them, so that you regain access to your own question. $\endgroup$
    – Martin Sleziak
    Commented Sep 26, 2017 at 6:05
  • $\begingroup$ The account issue is fixed now.. $\endgroup$
    – Messages from various people
    Commented Oct 3, 2017 at 6:09

2 Answers 2

Reset to default
13
$\begingroup$

The answer is "yes":
A sphere glued into a soft 3d-mattress can rotate continuously.

Let $R_t\in SO(3)$ be the rotation by angle $t$ around the $z$-axis.

Pick a nullhomotopy $R_{t,s}$ ($s\in [0,1]$) of the map $[0,4\pi]\to SO(3):t\mapsto R_t$.
So $R_{t,0}=R_t$ and $R_{t,1}=\mathrm{id}$, for all $t\in [0,4\pi]$.

Now here's the description of the motion of the mattress:

At time $t$, the sphere of radius $1+s$ performs the rotation $R_{t,s}$.
(The mattress is immobile outside the sphere of radius 2.)

Improve this answer
$\endgroup$
1
  • $\begingroup$ The new animation youtu.be/tazjVJcxm50 illustrates your answer. Thank you for the clear argument. I cannot tick it as the official answer because I had problems logging in when I posted the question. Sorry! $\endgroup$
    – Messages from various people
    Commented Sep 26, 2017 at 4:58
2
$\begingroup$

This is sometimes called Dirac's belt trick. There is a Wikipedia article under the name the plate trick. As that article says, it demonstrates the theorem that "SU(2) (which double-covers SO(3)) is simply connected."

          Dirac Belt Trick illustrated by George Francis.
Dirac Belt Trick illustrated by George Francis. From Carlo H. Séquin, "Torus Immersions and Transformations," 2013.

Improve this answer
$\endgroup$
4
  • 2
    $\begingroup$ True, but the belt/plate trick does not imply that the mattress trick works - at least not so simply. That is the reason for the question. $\endgroup$
    – Messages from various people
    Commented Sep 24, 2017 at 11:36
  • 3
    $\begingroup$ (This answer was posted before the OP significantly revised the question.) $\endgroup$
    – Joseph O'Rourke
    Commented Sep 24, 2017 at 11:51
  • 1
    $\begingroup$ Jason Hise has many other great visualizations of the belt trick on entropygames.net . Additional ones are found on motionmountain.net/videos.html and in my free physics text. $\endgroup$
    – Messages from various people
    Commented Sep 24, 2017 at 12:04
  • $\begingroup$ There are even papers on the deep relation between the belt trick and Dirac's equation. $\endgroup$
    – Messages from various people
    Commented Sep 24, 2017 at 12:05

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .