Assume that $\Omega$ is a bounded domain such that $\partial\Omega=\Gamma_1\cup \Gamma_2$, where $\Gamma_1$ and $\Gamma_2$ are disjoint and closed. Let us consider the following elliptic equations. $$ \left\{\begin{array}{l} \nabla\cdot (\gamma(x) \nabla u(x))=0,\quad x\in\Omega,\\ -\gamma(x) \frac{\partial u(x)}{\partial n}=k u(x),\quad x\in\Gamma_1, \\ \gamma(x)\frac{\partial u(x)}{\partial n}=q, \quad x\in\Gamma_2 \end{array}\right. $$ There are two questions that I have about this problem:
(1) If $q\in L^2(\partial\Gamma_2)$, then can we get weak solution $u$ in $H^{3/2}(\Omega)$. From Theorem 7.3 in the book "Non-Homogeneous Boundary Value Problems and Applications" it seems that the solution exists if $\Omega$ is smooth. The argument is just too long, because it focus on general elliptic equations of order $2m$ $$ \left\{\begin{array}{l} Au=f\\ B_ju=g \quad 0\leq j\leq m-1 \end{array}\right. $$ and say that the map $u\to \{Au, B_0u, B_1u,\cdots, B_{m-1}u\}$ is onto from $H^{s}$ to $H^{s-2}(\Omega)\times H^{s-1/2-m_0}(\partial\Omega)\times H^{s-1/2-m_1}(\partial\Omega)\cdots H^{s-1/2-m_{m-1}}(\partial\Omega)$. Is there any other books or material on this result? I means the ones with short proof or you think are better written.
(2) On the other hand, I know the following result, which only requrie $\Omega$ to be Lipschitz, and the boudnary data is only $L^2$.
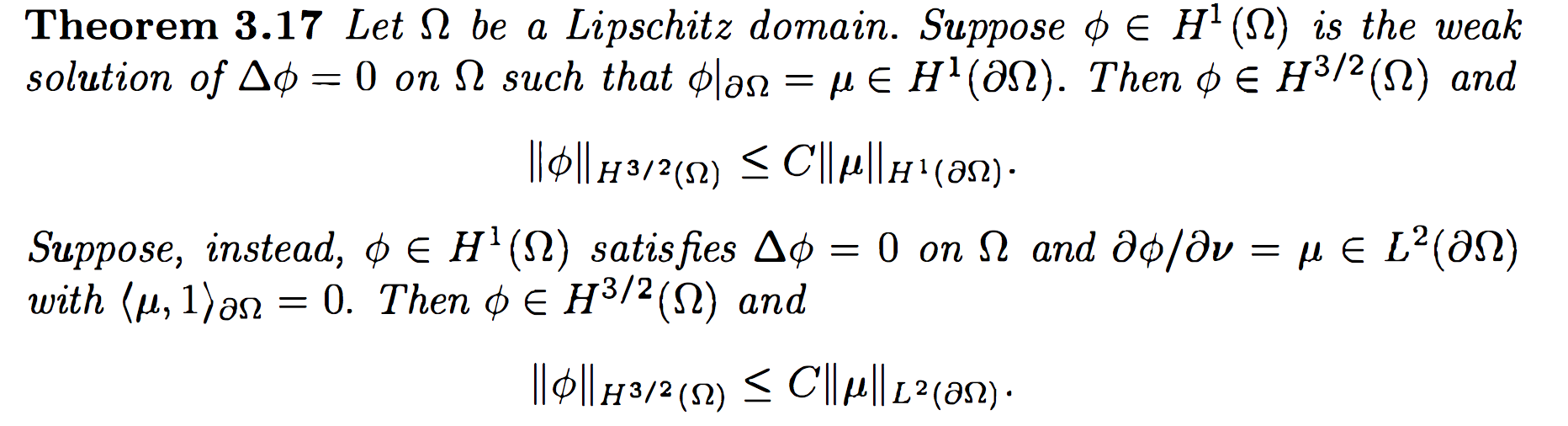
Does similar result apply the elliptic equation above.
