Let $V(\lambda)$ be the unique irreducible representation of a Kac—Moody algebra $\mathfrak{g}$ with the highest weight $\lambda$. If $\mathfrak{g}$ is not of finite type, then even for $\lambda$ one of the fundamental weights the weights of $V(\lambda)$ usually have multiplicities $\geqslant2$.
Is there a nice way to pick a basis in each of the weight spaces $V(\lambda)_\mu$?
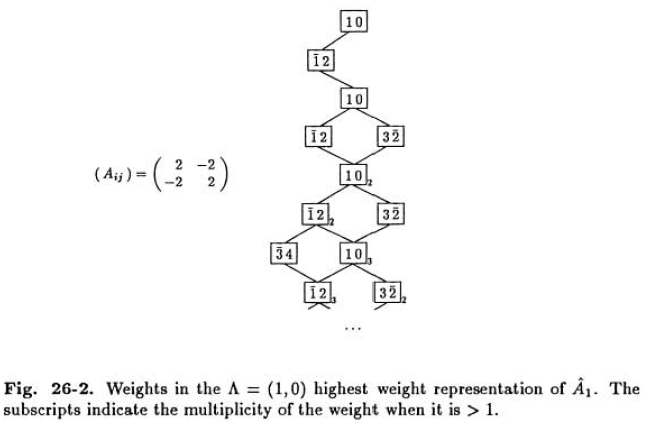
Here "nice" means that there is an explicit description of the action of the standard generators of $\mathfrak{g}$, so one could actually write (infinite) matrices for the Lie algebra elements. I have checked some sources including Lie Algebras of Finite and Affine Type by R. Carter and Affine Lie Algebras, Weight Multiplicities and Branching Rules by Kass, Moody, Patera and Slansky, but none contains such details even for the smallest cases like $\tilde{A}_1$. Here is a picture from the second:
As you can see, this diagram gives no hint to how exactly the generators act inside the weight spaces. I guess this can be reconstructed from the desription of $V(\lambda)$ as a quotient of the Verma module, but this seems quite computationaly extensive, so I was wondering whether someone has already done it in some cases (say, for the untwisted affine KM algebras for some particular choice of $\lambda$)?