8
$\begingroup$

The famous and remarkable Voronin's universality theorem states:

Theorem (Voronin 75): let $0<r<1/4$ and suppose that $g(s)$ is a nonvanishing continuous function on the disk $ \vert s \vert \leq r$ that is analytic in the interior. Then for any $\epsilon>0$, there exists a positive real number $\tau$ such that: $$max_{\vert s \vert \leq r} \vert \zeta(s+3/4+i \tau)-g(s) \vert <\epsilon. $$

Which practically means that $g(s)$ could be approximated by $\zeta(s)$ for some "high enough" values of $\tau$ - on the right hand side of the critical strip.

The thing is that there is a certain numerical "mystery" with respect to this theorem - when it comes to the question "how high is high enough"? Of course - there are effective analytic studies in various cases. But its worthwhile to pay attention to direct calculation via computer - it turns out to be really "hard" to verify Voronin numerically (in my eyes, the illustration makes the theorem even more impressive).

For instance - let us take the constant function $g(z)=e^{3}$.

Question: Is there any estimate on $\tau$ for which Voronin's approximation works for $g(s)=e^{3}$?

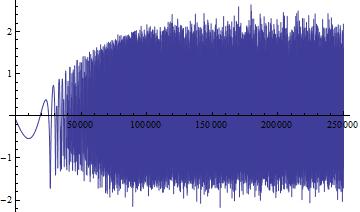
It is important to point out and compare, for instance, the following graph of $\log \vert \zeta(0.75+e^{0.0001 \tau} i) \vert$ for $\tau = 0,...,250000$ in this case:

\log \vert \zeta(0.75+e^{0.0001 \widetilde{\tau}} i) \vert$ for $\widetilde{\tau} = 0,...,250000$

As you can see - for quite big values - the function still doesn't seem to cross the bound $\pm 3$. So when does Voronin's theorem start to kick in this case?

(It is interesting to note also some implications to zeros of zeta (RH), for instance.)

Improve this question
$\endgroup$
8
  • $\begingroup$ See Titchmarsh's book chapter XI for a detailed proof of the universality of $\zeta$. $\endgroup$
    – reuns
    Commented Aug 7, 2017 at 14:50
  • 1
    $\begingroup$ Dear reuns. I am aware of the results in Titchmarsha's book (btw the first edition was written before Voronin). However, as I mentioned, these results are not effective at all. In the sense that they do not give an insight on where the first $ \tau$ should occur for a given function $g(s)$. The mystery I'm trying to point out is that, when coming to validate specific effective examples, this seems to be a non-trival question even when g(s) is a constant function! The thing is that it also has direct bearing on RH! $\endgroup$
    – Yochay Jerby
    Commented Aug 7, 2017 at 16:23
  • $\begingroup$ Well, the graph clearly illustrates that $\zeta$ doesn't have zeros for $Re(s)=0.75$ with $Im(s)$ up to around $e^{25}$. Thus - extenting the bound from below further - which is what this question is about - is quite interesting. $\endgroup$
    – Yochay Jerby
    Commented Aug 7, 2017 at 16:47
  • $\begingroup$ My question is very simple - do you have an estimate on when the graph is going to pass -3. If so you have an estimate on where zeta is non vanishing. $\endgroup$
    – Yochay Jerby
    Commented Aug 7, 2017 at 16:53
  • $\begingroup$ Of course no the answer isn't simple, but what you need if you are interested in the subject is in chapter XI. If you only want a result (not sure why) then search "effective universality zeta" $\endgroup$
    – reuns
    Commented Aug 7, 2017 at 16:55

1 Answer 1

Reset to default
4
$\begingroup$

Consider $e^3$ and $e^{-3}$ separately.

For $e^3$: Think about the order of $\zeta(s)$ in the critical strip: Titchmarsh Chapter V. Define $\mu(\sigma)$ as the lower bound of the numbers $\xi$ such that $$ \zeta(\sigma+it)=O(|t|^\xi). $$ The Lindelof Hypothesis (which is a consequence of the Riemann Hypothesis) is that $\mu(\sigma)=1/2-\sigma$ for $\sigma\le 1/2$ and $\mu(\sigma)=0$ for $\sigma>1/2$. In this case $\zeta(3/4+it)=O(|t|^\xi)$ is true for every $\xi>0$, so the zeta function grows very slowly on this vertical line. It's simply going to take a long time to reach $e^3$.

For $e^{-3}$: $\zeta(s)$ is near $e^{-3}$ when $1/\zeta(s)$ is near $e^3$. But on the Riemann Hypothesis, $1/\zeta(s)=O(t^\epsilon)$ for $\sigma>1/2$ and every positive $\epsilon$. (Titchmarsh (14.2.6)). So again, the function $1/\zeta(s)$ can grow only very slowly on the $3/4$ line.

Update: "Can you give an estimate...?" The point of my answer was to show why $\tau$ will likely be quite large for the function you chose, beyond the range where computation is easy. Your comment below indicates you're not really asking about Voronin's Theorem; you're asking about making effective the constants implied by Titchmarsh's use of O. Many of these can be made effective by paying careful attention to the proofs. It depends how badly you need them whether it's worth the effort.

Improve this answer
$\endgroup$
5
  • 3
    $\begingroup$ That's the whole point - You are using big O! I'm asking a very simple question which is not included in big O argument. The question is -can you give an estimate on when the graph as a function of $\tau$ would pass the value 3? $\endgroup$
    – Yochay Jerby
    Commented Aug 7, 2017 at 16:35
  • $\begingroup$ @YochayJerby See Titchmarsh's book chapter XI :-) $\endgroup$
    – reuns
    Commented Aug 7, 2017 at 16:50
  • 2
    $\begingroup$ Even in the second edition, Titchmarsh only considers the earlier work of Bohr and Jessen on the distribution of values of zeta on a fixed vertical line, and not the functional distribution as in Voronin's Theorem. $\endgroup$
    – Denis Chaperon de Lauzières
    Commented Aug 7, 2017 at 17:34
  • 1
    $\begingroup$ I am familiar with chapter XI. However - note that when you are making an asymptotic argument with big O - you are saying nothing about what happens with the "first" values of the function - also you do not specify where the asymptotics start to "kick in". In the past - computing millions of values of $\zeta$ and viewing them in a graph form - was way out of reach. But note that an asymptotic argument doesn't tell you that $log \vert \zeta(0.75 + \tau i ) \vert $ is actually bounded in $[-2,2]$ for $[0,e^{25}]$. $\endgroup$
    – Yochay Jerby
    Commented Aug 7, 2017 at 19:58
  • $\begingroup$ Hey Stopple - thanks for the new comment - yes the point I am really trying to make is that, at least from a computation in this initial domain (up to $e^{25}$) it seems that together with the universality property there is some regulated behavior of $\vert \zeta(x+yi) \vert $ for $x \in (0.5,1]$ - maybe some global very slowly decreasing natural function that bounds $\vert \zeta(x+yi) \vert $ from below? Again - it's just a question based on how the graph looks like until $e^{25}$. $\endgroup$
    – Yochay Jerby
    Commented Aug 10, 2017 at 11:10

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .