0
$\begingroup$

Questions.

EDIT: readers please note that while this question arose in research, the OP was so hung-up on a question concerning infinite planar graphs that a strong a-forteriori-reason, kindly pointed out by the answerer below, for why (A) is true was overlooked. The question as it stands is trivial. It will perhaps be edited to include the (still open) graph theoretical question, in due course. END OF EDIT

(0) How would you prove, in usual topology, the following assertion:

(A) There does not exist any plane isotopy carrying the subset $S=\{ (\exp(t)\ \cos(t),\exp(t)\ \sin(t)|\quad t\in\mathbb{R}\}\subseteq\mathbb{R}^2$ onto the subset $R=\{ (t,0)|\quad t\in\mathbb{R}\}\subseteq\mathbb{R}^2$.

(1) Independent of (0), in a bibliographic/reference-requestish vein:

(B) In what literature references does (something equivalent to) (A) recognizably appear? (I'm interested in as many relevant references as possible, in any of the media: book, lecture notes, research paper, website.)

Remarks.

  • In $A$, all technical terms are standard terms of basic topology nowadays. The term plane isotopy would in some contexts often called by the more general term ambient isotopy. For what it's worth, a definition of the central notion here is the following.

Let

$\eta_S\colon \mathbb{R}\rightarrow\mathbb{R}^2$ be given by $\eta_S(t) = (\exp(t)\cdot\cos(t),\exp(t)\cdot\sin(t))$,

and

$\eta_R\colon \mathbb{R}\rightarrow\mathbb{R}^2$ be given by $\eta_R(t) = (t,0)$.

Then a plane isotopy is any continuous set-map

$\theta\colon \mathbb{R}^2\times[0,1]\rightarrow\mathbb{R}^2$

satisfying the three axioms

(A.0) for all $v\in\mathbb{R}^2$, $v=\theta(v,0)$,

(A.1) for all $t\in[0,1]$, $v\mapsto\theta(v,t)$ defines a homeomorphism $\mathbb{R}^2\rightarrow\mathbb{R}^2$,

(A.2) $(v\mapsto \theta(v,1))\circ\eta_S = \eta_R$, equal as set-maps.

  • I am less, but also, interested in the correct answer here, more in different writing- and proof-styles, some more efficient than others, localized at this very question.

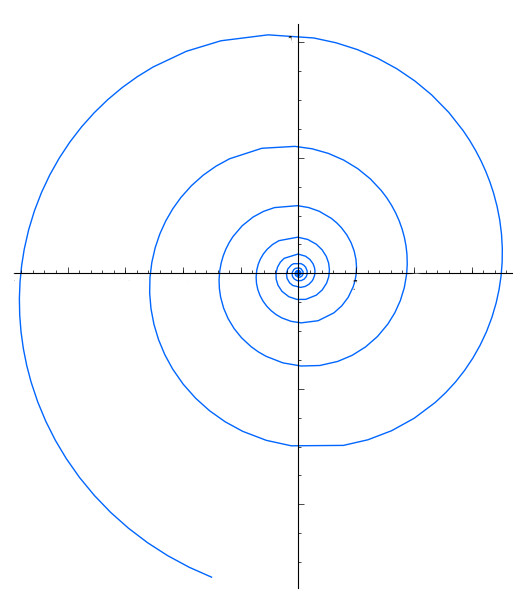
  • Of course, $S\subseteq\mathbb{R}^2$ 'looks' something like the blue line in the following illustration: enter image description here

(Made with Sage.)

  • Motivation for this question is that (A) came up in research about (three-connected) infinite planar graphs, and I need to know more about and around it.
Improve this question
$\endgroup$
12
  • 8
    $\begingroup$ Why the emphasis on "classical logic"? As opposed to what? (I mean, most of mathematics uses classical logic by default: I find the qualification as bizarre as if my box of breakfast cereals advertised "does not contain plutonium!") $\endgroup$
    – Gro-Tsen
    Commented Aug 5, 2017 at 8:33
  • 2
    $\begingroup$ Dear Gro-Tsen, thanks for the question. To me, there is nothing bizarre about this and the polemical culinary and chemical words were brought in by you. Re "As opposed to what": as opposed to some well-known contemporary foundational research on how to prove certain basic statements of algebraic topology in non-classical logic, and which to describe this comment is too small. This is not about one logic being right and the other wrong, these are straight technical matters, "proof in classical logic" is just about as precisely defined as "proof in constructive logic" (modulo details). $\endgroup$
    – Peter Heinig
    Commented Aug 5, 2017 at 8:50
  • 5
    $\begingroup$ @PeterHeinig Of course there is a contemporary foundational research, but by default, if you ask a question, it is in classical logic. You don't have to say it each time, hence the analogy in Gro-Tsen's comment. $\endgroup$
    – Maxime Ramzi
    Commented Aug 5, 2017 at 8:51
  • 9
    $\begingroup$ The spiral is not a closed subset of the plane, while the line is, so they can't be identified by a homeomorphism of the plane, let alone an isotopy. Can you help me see what I am missing here? $\endgroup$
    – Ben McKay
    Commented Aug 5, 2017 at 9:17
  • 1
    $\begingroup$ @PeterHeinig I'm sorry you found my comment offensive, it was not meant to be. (The analogy was mostly meant to be funny — I think there was an xkcd comic along those lines, even though I can't find it any more. But it was also meant to convey my sincere confusion and my impression that I was missing something.) $\endgroup$
    – Gro-Tsen
    Commented Aug 5, 2017 at 14:17

1 Answer 1

Reset to default
7
$\begingroup$

A stronger result is true: there is no homeomorphism of the plane which takes the spiral to the line. If the spiral is defined exactly as in your question (does not contain the origin) this is evident: your spiral in not a closed subset of the plane, while the line is.

But even if you add the origin to your definition of the spiral, the result still holds. Simply because the spiral with the origin added is not homeomorphic to the line.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you. I had intentionally not added the origin since I thought "Now that would really trivialize the question." since, like you said, then $R$ and $S$ would not even be homeomorphic (which in the OP they are). FYI: adding the origin correspond to adding an end to the double ray (if one associates a two-way infinite double ray graph with the spiral). $\endgroup$
    – Peter Heinig
    Commented Aug 5, 2017 at 13:47

Not the answer you're looking for? Browse other questions tagged .