Using a Pappian-ish configuration, we can get $n = 5$ and $n = 7$.
For $n = 7$, put points at $(x,0)$ and $(x,1)$ for integer $x$ with $0 \le x \le 3$, at $(x,1/2)$ for integer or half-integer $x$ with $1/2 \le x \le 5/2$, and at horizontal infinity. Color them like this:
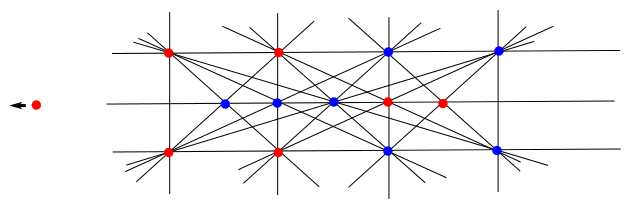
For $n = 5$, remove the rightmost two blue points and the rightmost two red points, turn the rightmost blue point on the central horizontal line red, and make one of the red points on the middle vertical line blue. This construction breaks down for $n > 7$ since you start to get lines of shallow enough slope to just connect two points lying entirely in one "half" of the diagram.
Edited following the suggestion of Jan Kyncl:
A modification of the above in fact gives a configuration for any $n \ge 4$. For $n = 3k+1$ with $k \ge 1$, put points at $(x,0)$ and $(x,1)$ for integer $0 \le x \le 2k-1$, at $(x,1/2)$ for integer or half-integer $(k-1)/2 \le (3k-1)/2$, and at horizontal infinity. Then color the points on the horizontal lines $y = 0$ and $y = 1$ red if $x < k$ and blue if $x \ge k$, color the points on the horizontal line $y = 1/2$ blue if $x < k$ and red if $x \ge k$, and color horizontal infinity red. (This directly extends the $n = 7$ case drawn above)
For $n = 3k$ with $k \ge 2$, just omit the leftmost and rightmost point on the central horizontal line.
For $n = 3k + 2$ with $k \ge 1$, put points at $(x,0)$ and $(x,1)$ with integer $0 \le x \le 2k$, at $(x,1/2)$ with $k/2 \le x \le 3k/2$, and at horizontal infinity. On the outer lines $y = 0$ and $y = 1$, color points red if $x < k$ and blue if $x > k$, and on the central line $y = 1/2$ color points red if $x > k$ and blue if $x < k$. On the line $x = k$, color $(k,1)$ red, $(k,1/2)$ and $(k,0)$ blue, and color horizontal infinity red.

