2
$\begingroup$

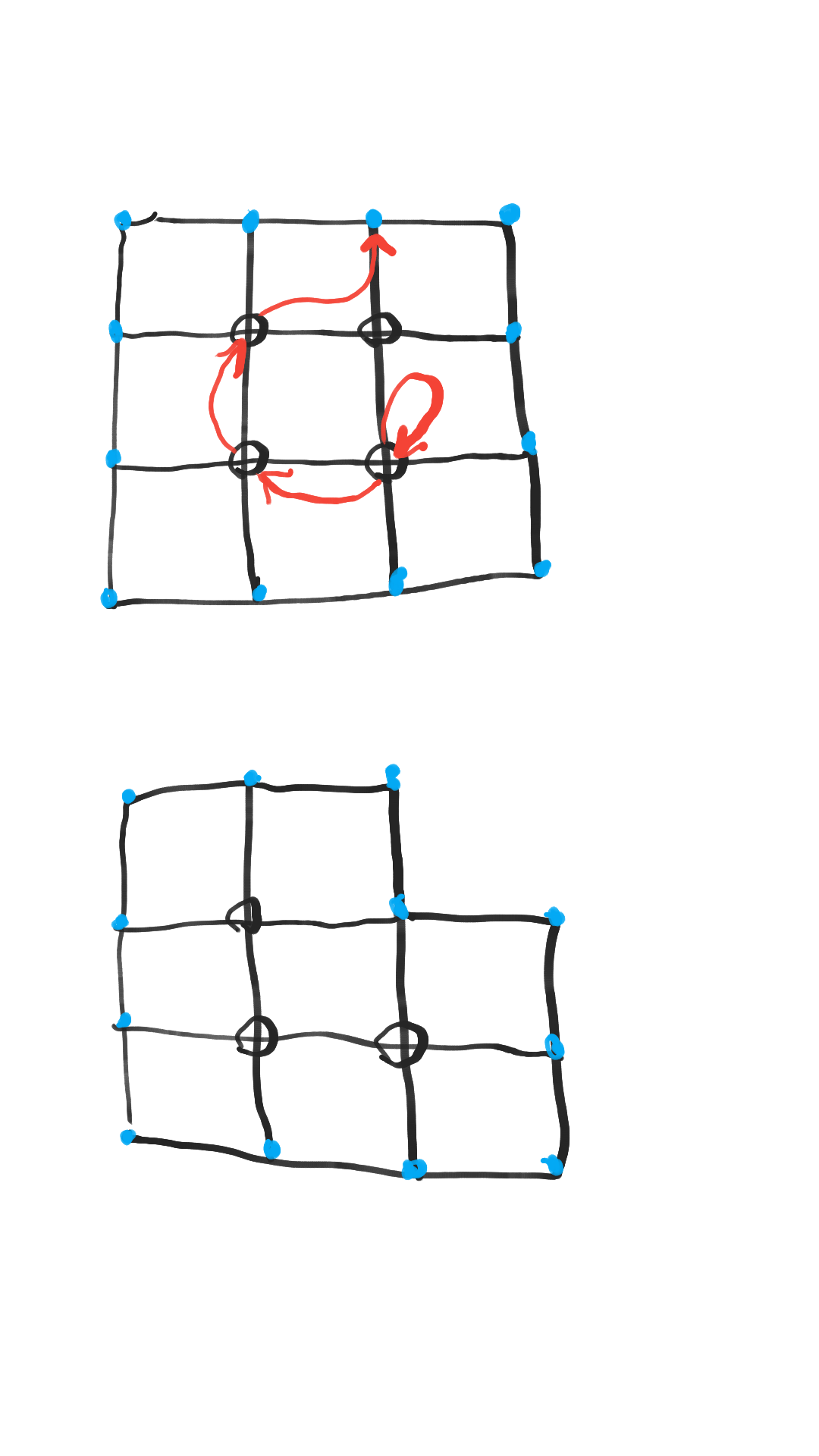
The first picture below has $v=12$ vertices, $e=16$ edges, and seems to have $k=4$ crosscaps (denoted by something like $\oplus$). The number of faces $f$ should satisfy $$v-e+f=2-k$$ which gives $f=2$. However looking at the picture we see $f=3$. A possible explanation is that one crosscap is superfluous as the red path shows.

The second picture has no superfluous crosscaps.

My question is, is there a simple way to identify superfluous crosscaps in more complicated situations? My only heuristic so far is that a "loop" of crosscaps has 1 superfluous crosscap.enter image description here

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
4
$\begingroup$

The Euler characteristic formula only applies when all the faces are homeomorphic to disks. This is the case in the second picture. However, in the first picture, one of the faces is homeomorphic to an annulus. It is possible to modify the formula so that the faces are counted with a weighting based on their individual Euler characteristics. The number of crosscaps is intrinsic to the topology of the surface so I would not describe any of them as superfluous.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for the annulus idea. By superfluous I meant that that crosscap doesn't really help in drawing the graph without crossings on the surface. $\endgroup$
    – Bjørn Kjos-Hanssen
    Commented Jul 3, 2018 at 7:40

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .